A thin rod of length f/3 lies along the axis of a concave mirror of focal length f. One end of its magnified image touches an end of the rod. The length of the image is
f
12f
2f
14f
The correct Answer is:D
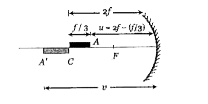
If end A of rod act as an object for mirror then its image will be A. and if u=2f−f3=5f3
So using 1f=1v+1u,1−f=1v+1−5f3⇒v=−52f
∴ Length of image =52f−2f=f2
Similar Questions
A short linear object of length b lies along the axis of a concave mirror or focal length f at a distance u from the pole of the mirror. The size of the image is approximately equal to
View SolutionA thin rod of length (f3) is lying along the principal axis of a concave
mirror of focal length f.Image is real , magnified and inverted and one of the end or rod coincides with its image itself. Find length of the image.
View Solution
Knowledge Check
A short linear object of length / lies along the axis of a concave mirror of focal length f at a distance d from the pole of the mirror. The approximate size of the image is
AIf(d+f)Bd+ffICIf2(d+f)2DI(d+f)2f2A short linear object of length b lies along the axis of a concave mirror or focal length f at a distance u from the pole of the mirror. The size of the image is approximately equal to
Alfd−fBd−flfClf2(d−f)2D(d−f)2f2lA short linear object of length / lies along normal to the axis of a concave mirror of focal length f at a distance d from the pole of the mirror. The size of the image is then (nearly)
 Af2l(d+f)2Bd+fflCfl(f+d)D(d+f)2lf2
Af2l(d+f)2Bd+fflCfl(f+d)D(d+f)2lf2
A thin rod of length (f3) is lying along the principal axis of a concave
mirror of focal length f.Image is real , magnified and inverted and one of the end or rod coincides with its image itself. Find length of the image.
View SolutionA rod of length (f/2) is placed along the axis of a concave mirror of focal length f. If the near ead of the real image formed by the mirror just touches the far end of the rod, find its magnification.
View SolutionA thin rod of length f/3 is placed along the optic axis of a concave mirror of focal length f such that its image which is real and elongated, just touches the rod. The magnification is _____________
View SolutionA short linear object of length b lies along the axis of a concave mirror or focal length f at a distance u from the pole of the mirror. The size of the image is approximately equal to
View SolutionA short linear object of length b lies along the axis of a concave mirror of focal length f at a distanee u from the pole of the mirror. The size of the image is approximately equal to
View Solution
