Question

The size of the image of an object, which is at infinity, as formed by a convex lens of focal length 30 cm is 2 cm. Ifa concave lens of focal length 20 cm is placed between the convex lens and the image at a distance of 26 cm from the convex lens, calculate the new size of the image

Options

A. 1.25 cm
B. 2.5 cm
C. 1.05 cm
D. 2 cm

Answer

B

Explanation

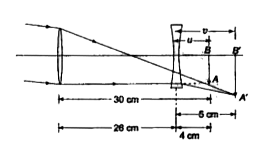
AB is the image of size 2 cm formed by the convex lens at its focal plane. It serves as the virtual object for the concave lens. A.B. is the final image. For concave lens, f = -20 cm, u = +4 cm.
`(1)/(v) - (1)/(u) = (1)/(f)` or `(1)/(v) - (1)/(4) = -(1)/(20)`
which gives v = 5 cm. Thus the final image at a distance of 5 cm from the concave lens.
Now `(A.B.)/(AB) = (v)/(u)` or `(A.B.)/(2 cm) = (5 cm)/(4 cm)` or A.B. = 2.5 cm.