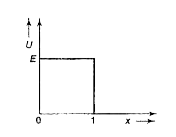
The potential energy U of a moving particle of mass m varies with x as shown in fig. The de Broglie wavelengths of the particle in the regions $0 \leq x \leq 1 and x > 1$ are $λ_{1} and λ_{2}$ respectively. If the total energy of the particle is the ratio $λ_{1} / λ_{2}$ is

A

$\frac{n}{(n - 1)}$

B

$\sqrt{\frac{n}{(n - 1)}}$

C

$\sqrt{\frac{n^{2}}{(n^{2} - 1)}}$

D

$\frac{n^{2}}{(n^{2} - 1)}$

Text Solution

Verified by Experts

The correct Answer is:B

Total energy $= K E + P E = K + U$
In the region $0 \leq x \leq 1, U_{1} = E .$ Therefore, kinetic energy is
$K_{1} =$ total energy $- U_{1} = n E - E (n - 1) E ∴ λ_{1} = \frac{h}{\sqrt{2 m K_{1}}} = \frac{h}{\sqrt{2 m (n - 1) E}}$
In the region $x > 1, U_{2} = 0 .$ Therefore, kinetic energy
$K_{2} = n E - 0 = n E$
$∴ λ_{2} = \frac{h}{\sqrt{2 m K_{2}}} = \frac{h}{\sqrt{2 m m E}} (2)$
Dividing (1) by (2), we get $\frac{λ_{1}}{λ_{2}} = \sqrt{\frac{n}{(n - 1)}}$

|

Class 12 PHYSICS DUAL NATURE OF RADIATION AND MATTER

Similar Questions

Knowledge Check

The potential energy of particle of mass m varies as
$U (x) = {\begin{matrix} E_{0} for 0 \leq x \leq 1 0 for > 1 \end{matrix}$
The de Broglie wavelength of the particle in the range $0 \leq x \leq 1 is λ_{1}$ and that in the range $x > 1 is λ_{2}$ .
If the total of the particle is $2 E_{0}, find λ_{1} / λ_{2}$ .
A $\sqrt{2}$
B $\sqrt{3}$
C $\sqrt{\frac{1}{2}}$
D $\sqrt{\frac{2}{3}}$
The potential energy of particle of mass m varies as
$U (x) = {\begin{matrix} E_{0} for 0 \leq x \leq 1 0 for > 1 \end{matrix}$
The de Broglie wavelength of the particle in the range $0 \leq x \leq 1 is λ_{1}$ and that in the range $x > 1 is λ_{2}$ .
If the total of the particle is $2 E_{0}, find λ_{1} / λ_{2}$ .
A $\sqrt{2}$
B $\sqrt{3}$
C $\sqrt{\frac{1}{2}}$
D $\sqrt{\frac{2}{3}}$
The potential energy of a particle of mass m is given by $U (x) {\begin{matrix} E_{0} & 0 \leq x < 1 0 & x > 1 \end{matrix}$
$λ_{1}$ and $λ_{2}$ are the de-Broglie wavelength of the particle, when $0 \leq x \leq 1$ and $x > 1$ respectively. If the total energy of particle is $2 E_{0}$ , then the ratio $\frac{λ_{1}}{λ_{2}}$ will be
A2
B1
C $\sqrt{2}$
D $1 / \sqrt{2}$

Recommended Questions

Home
Ask Doubt
Profile