A proton is bombarded on a stationary lithium nucleus. As a result of the collision two $α$ -particles are produced. If the direction of motion of the $α$ -particles with the initial direction of motion makes an angle ${cos}^{- 1} (\frac{1}{4}),$ find the kinetic energy of the striking proton. Given binding energies per nucleon of $L i^{7} and H e^{4}$ are 5.60 and 7.06 MeV respectively. (Assume mass of proton = mass of neutron)

34.56 MeV

1.728 MeV

17.28 MeV

71.28 MeV

Text Solution

Verified by Experts

Q value of the reaction is $Q = (2 \times 4 \times 7 .06 - 7 \times 5.6) M e V = 17.28 M e V$
Applying conservation of energy for collision, $K_{P} + Q = 2 K_{α} (i)$
(Here $K_{P} and K_{α}$ are the kinetic energies of proton and $α -$ partice respectively)

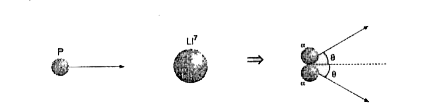
From conservation of linear momentum, $\sqrt{2 m_{p} K_{p}} = 2 \sqrt{2 m_{a} K_{a}} cos θ (i i)$
$∴ K_{p} = 16 K_{a} {cos}^{2} θ = (16 K_{a}) {(\frac{1}{4})}^{2} (a s m_{a} = 4 m_{p})$
$∴ K_{a} = K_{p} (i i i)$
Solving Eqs. (i) and (iii) with $Q = 17.28 M e V$
we get $K_{P} = 17.28 M e V$

Updated on:21/07/2023

The energy of the reaction $L i^{7} + p \to 2 H e^{4}$ is ( the binding energy per nucleon in $L i^{7}$ and $H e^{4}$ nuclei are $5.60$ and $7.60 M e V$ respectively.)
A $17.3 M e V$
B $1.73 M e V$
C $1.46 M e V$
Ddepends on binding energy of proton
Calculate the energy of the reaction ,
$L i^{7} + p \to 2_{2} H e^{4}$
If the binding energy per nucleon in $L i^{7}$ and $H e^{4}$ nuclei are 5.60 MeV and 7.06 MeV , respectively .
A19.6 MeV
B2.4 MeV
C8.4 MeV
D17.28 MeV
If the binding energy per nucleon in $L i^{7}$ and $H e^{4}$ nuclei are respectively $5.60 M e V$ and $7.06 M e V$ . Then energy of reaction $L i^{7} + p \to 2_{2} H e^{4}$ is.
A19.6 MeV
B2.4 MeV
C8.4 MeV
D17.3 MeV