When a.c. flows through a capacitor, the peak value of current in the circuit is........
To find the peak value of current when alternating current (AC) flows through a capacitor, we can follow these steps:
1. Understand the Circuit:
- We have a capacitor connected to an AC voltage source. The voltage across the capacitor can be expressed as V(t)=Vmsin(ωt), where Vm is the peak voltage and ω is the angular frequency.
2. Charge on the Capacitor:
- The charge Q on the capacitor is related to the voltage by the equation Q=C⋅V, where C is the capacitance. Thus, substituting the expression for voltage, we get:
Q(t)=C⋅Vmsin(ωt)
3. Current in the Circuit:
- The current I flowing through the capacitor is the rate of change of charge with respect to time:
I(t)=dQdt
- Differentiating Q(t):
I(t)=ddt(C⋅Vmsin(ωt))=C⋅Vm⋅ddt(sin(ωt))
- Using the derivative of sine, we have:
ddt(sin(ωt))=ωcos(ωt)
- Therefore, the expression for current becomes:
I(t)=C⋅Vm⋅ωcos(ωt)
4. Peak Value of Current:
- The peak value of the current Im occurs when cos(ωt) is at its maximum value (which is 1):
Im=C⋅Vm⋅ω
- We can also express this in terms of the peak voltage and the capacitive reactance XC:
Im=VmXC
- Where XC=1ωC. Thus, substituting this into the equation gives:
Im=Vm⋅ωC
Final Result:
The peak value of current in the circuit when AC flows through a capacitor is given by:
Im=Vm⋅ωC
- We have a capacitor connected to an AC voltage source. The voltage across the capacitor can be expressed as V(t)=Vmsin(ωt), where Vm is the peak voltage and ω is the angular frequency.
- The charge Q on the capacitor is related to the voltage by the equation Q=C⋅V, where C is the capacitance. Thus, substituting the expression for voltage, we get:
Q(t)=C⋅Vmsin(ωt)
- The current I flowing through the capacitor is the rate of change of charge with respect to time:
I(t)=dQdt
- Differentiating Q(t):
I(t)=ddt(C⋅Vmsin(ωt))=C⋅Vm⋅ddt(sin(ωt))
- Using the derivative of sine, we have:
ddt(sin(ωt))=ωcos(ωt)
- Therefore, the expression for current becomes:
I(t)=C⋅Vm⋅ωcos(ωt)
- The peak value of the current Im occurs when cos(ωt) is at its maximum value (which is 1):
Im=C⋅Vm⋅ω
- We can also express this in terms of the peak voltage and the capacitive reactance XC:
Im=VmXC
- Where XC=1ωC. Thus, substituting this into the equation gives:
Im=Vm⋅ωC
The peak value of current in the circuit when AC flows through a capacitor is given by:
Im=Vm⋅ωC
Topper's Solved these Questions
- E.M.I. and A.C.BOOK - MBD -HARYANA BOARDCHAPTER - E.M.I. and A.C.EXERCISE - Exercise
10 Videos
- DUAL NATURE OF MATTER AND RADIATIONSBOOK - MBD -HARYANA BOARDCHAPTER - DUAL NATURE OF MATTER AND RADIATIONSEXERCISE - OBJECTIVE TYPE QUESTIONS
17 Videos
- Electromagnetic WavesBOOK - MBD -HARYANA BOARDCHAPTER - Electromagnetic WavesEXERCISE - Example
7 Videos
Similar Questions
A capacitor of capacitance 10μF is connected to a 220 V , 30 Hz alternating current source . Calculate the peak value of voltage across the capacitor . Also calculate the rms value of current in the circuit .
View SolutionA 2μF capacitor, 100 ohm resistor and 8 H inductor are connected in series with an a.c. source. What should be the frequency of soure for which the current drawn in the circuit is maximum? If peak value of emf of the source is 200 V, find the maximum current, inductive reactance, capactive reactance, total impedance, peak value of current in the circuit. What is the phase relation between voltages across inductor and resistor ? Also, give the phase relation between voltages acorss inductor and capacitor.
View Solution
Knowledge Check
At resonance the peak value of current in L−C−R series circuit is
AE0/RBE0√R2+(ωL−1ωC)2CE0√R2+(ωL−1ω2C∘)2DE0√2RAt resonance the peak value of current in a series LCR circuit is
Ae0ZBe0/ZCe0De0/RLet f = 50 Hz, and C = 100 μF in an AC circuit containing a capacitor only. If the peak value of the current in the circuit is 1.57 A at t = 0. The expression for the instantaneous voltage across the capacitor will be
AC=50sin(100πt−π/2)BC=100sin(50πt)CC=50sin100πtDC=50sin(100πt+π/2)
A 60 μF capacitor is connected to a 110 V, 60 Hz a.c. supply. Determine the rms value of the current in the circuit.
View Solution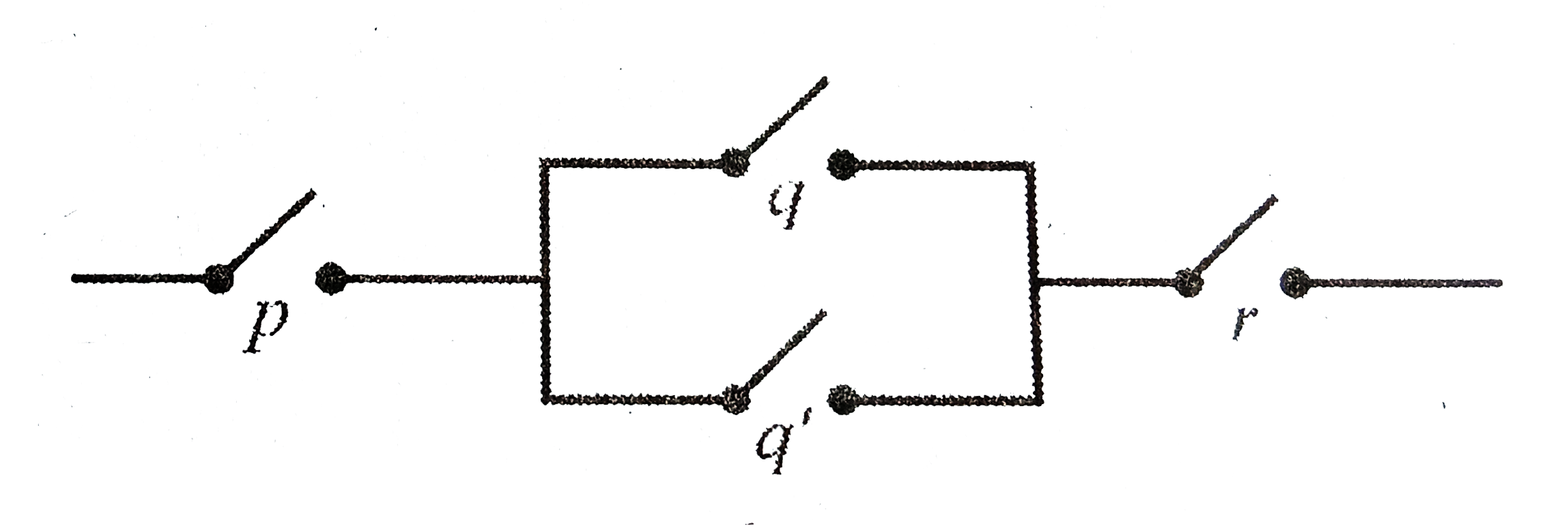
Current flows through the above circuit when
View SolutionWhen AC passes through capacitor the current
View SolutionThe peak value of 220 a.c. is
View SolutionTotal current flowing through the circuit:

View Solution
