Find all the values of the parameter 'a' for which the inequality 4x−a2x−a+3≤0 is satisfied by at least one real x.Solution in Gujrati
Putting 2x=t>0 then the original equation reduced in the form
t2−at−a+3=0………i
that the quadratic eq. I should have atleast one positive root (t>0) then
Discriminant D=(−a)2−4.1.(−a+3)≥0
⇒a2+4a−12≥0
⇒(a+6)(a−2)≥0
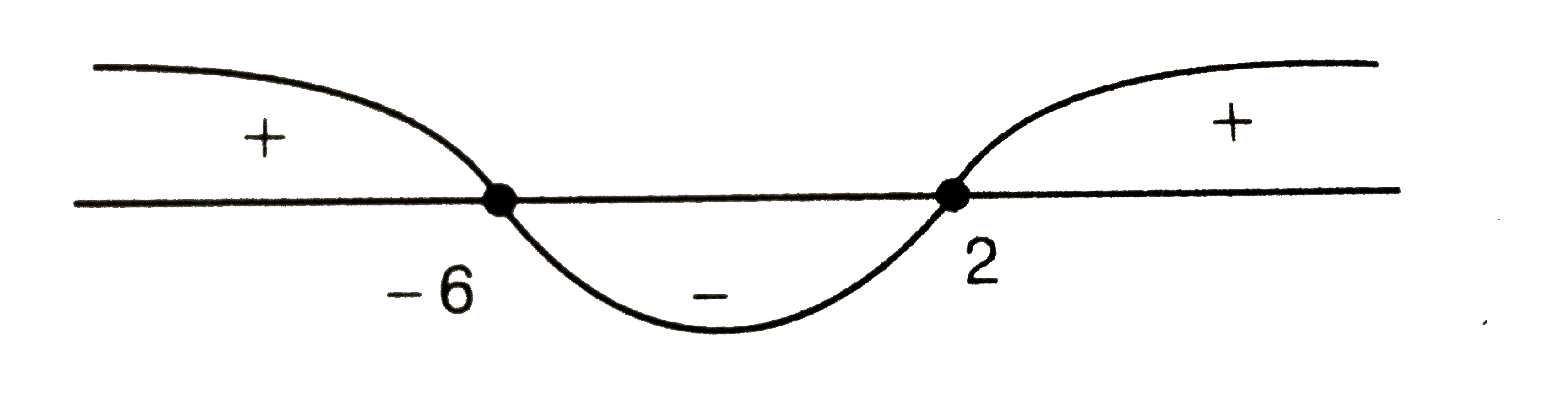
∴aε(−∞,−6]∪[2,∞)
If roots of eq i are t1 and t2 then
{t1+t2=at1t2=3−a
For aε(−∞,−6]
t1+t2<0 and t1t2>0. Therefore both roots are negatie and consequently the original equation has no solutions.
for aε[2,∞)
t1+t2>0 and t1t2>0 consequently atleast one of the roots t1 or t2 is greater than zero.
Thus, for aε[2,∞) the given equation has atleast one solution.
Similar Questions
Consider the inequation 9x−a3x−a+3≤0, where a is real parameter.
The given inequality has at least one negative soluiton for a∈
View SolutionConsider the inequation 9x−a3x−a+3≤0, where a is real parameter.
The given inequality has at least one real solutions for a∈.
View SolutionFind all values of x that satisfies the inequality 2x−3(x−2)(x−4)< 0.
View SolutionFind all values of x that satisfies the inequality 2x−3(x−2)(x−4)<0
View SolutionConsider the inequation x2+x+a−9<0
The values of the real parameter a so that the given inequations has at least one negative solution.
View SolutionFind all values of the parameter a for which the inequality a.9x+4(a−1)3x+a>1 is satisfied for all real values of x
View SolutionThe values of 'a' for which the equation 4x−a2x−a+3=0 has at least one solution.
View SolutionConsider the inequation x^(2) + x + a - 9 lt 0 The values of the real parameter a so that the given inequations has at least one negative solution.
View Solution
