If α,β be the roots of 4x2−16x+c=0,c∈R such that 1<α<2and2<β<3, then the number of integral values of c is Solution in Gujrati
Video Solution
Text Solution
The correct Answer is:3
∵4x2−16x+c=0
⇒x2−4x+c4=0
Let f(x)=x2−4x+c4
Then the following case arises:
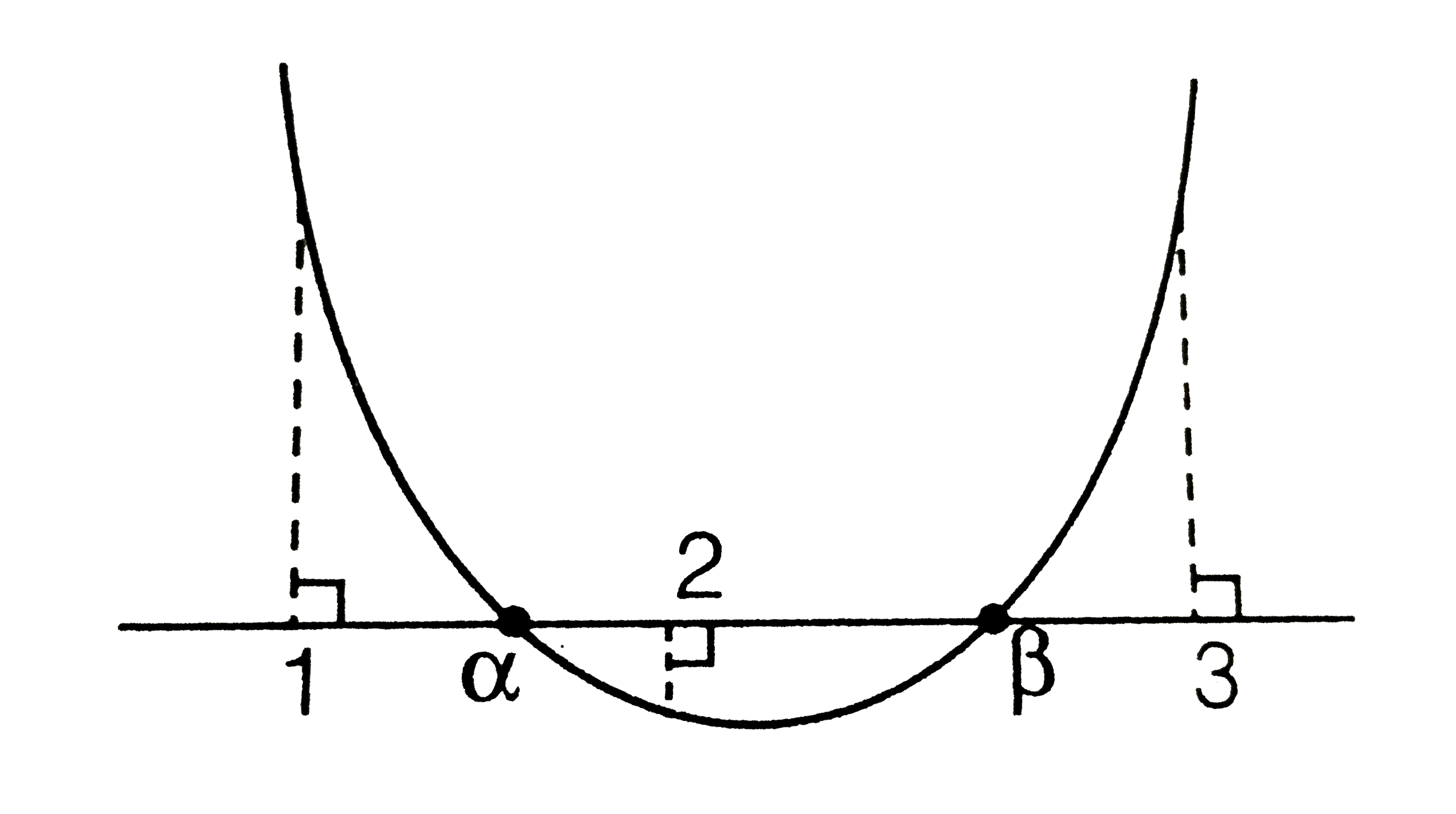
Case I D>0
=16−c>0
∴c<16
Case II f(1)>0 ltbr ⇒1−4+c4>0
⇒c4>3
∴c>12
Case III f(2)<0
⇒4−8+c4<0
⇒c4<4
c<16
Case IV f(3)>0
⇒9−12+c4>0
⇒c4>3
⇒c>12
Combining all cases, we get
12<c<16
Thus, integral values of c are 13, 14 and 15.
Hence number of integral values of c is 3.
|
Similar Questions
यदि α और β, समीकरण 4x2−16x+t=0,∀t>0 के मूल इस प्रकार हैं कि 1<α<2<β<3 है, तब t के पूर्णांक मानों की संख्या है -
View SolutionIf αandβ(α<β) are the roots of the equation x2+bx+c=0, where c<0<b, then
View SolutionIf αandβ(α<β) are the roots of the equation x2+bx+c=0, where c<0<b, then
View SolutionIf 0 lt c lt b lt a and the roots alpha, beta the equation cx^(2)+bx+a=0 are imaginary, then
View SolutionIf αandβ(α<β) are the roots of the equation x2+bx+c=0, where c<0<b, then
View SolutionIf αandβ(α<β) are the roots of the equation x2+bx+c=0, where c<0<b, then
View SolutionIf αandβ(α<β) are the roots of the equation x2+bx+c=0, where c<0<b, then
View SolutionIf αandβ(α<β) are the roots of the equation x2+bx+c=0, where c<0<b, then
View Solution
