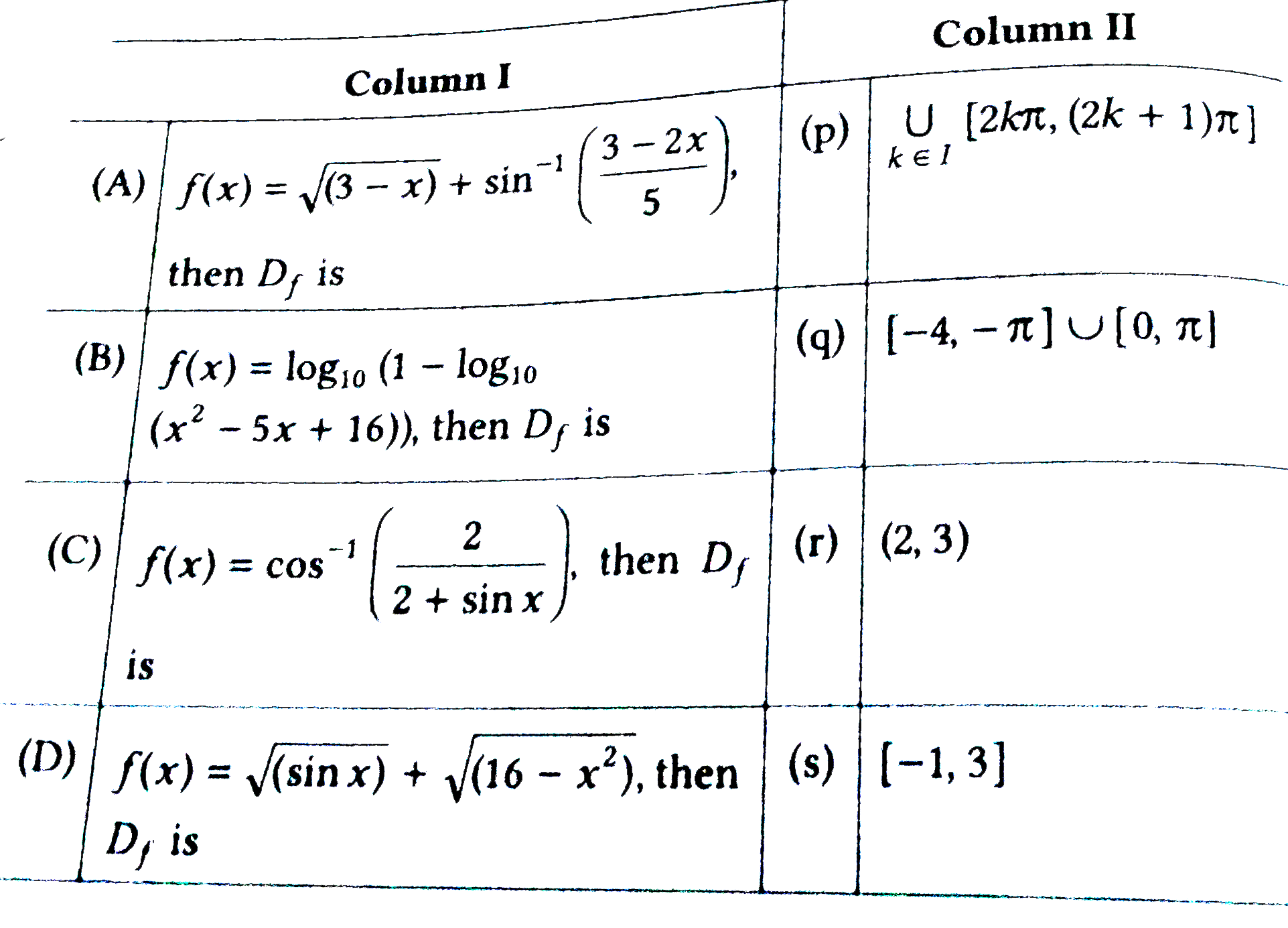
The domain of the function f(x) is denoted by $D_{f}$
Solution in Gujrati

Video Solution

Text Solution

Verified by Experts

The correct Answer is:(A) $\to$ (s); (B) $\to$ (r); (C ) $\to$ (p); (D) $\to$ (q)

Let $y = \sqrt{3 - x} + {sin}^{- 1} (\frac{3 - 2 x}{5})$
For y to be defined $3 - x \geq 0 on - 1 \leq \frac{3 - 2 x}{5} \leq 1 x \leq 3 ... (i)$
$- 5 \leq 3 - 2 x \leq 5$
and $- 1 \leq x \leq 4 ... (ii)$
From Eqs. (i) and (ii), we get
$x \in [- 1, 3]$
(B) Let $y = {log}_{10}$ ${1 - {log}_{10} (x^{2} - 5 x + 16)}$ for y to be defined $x^{2} - 5 x + 16 > 0 and 1 - {log}_{10} (x^{2} - 5 x + 16) > 0$
${(x - \frac{5}{2})}^{2} + \frac{39}{4} > 0 and {log}_{10} (x^{2} - 5 x + 16) < 1$
which is true, $\forall x \in R ... (i)$
$\Rightarrow x^{2} - 5 x + 16 < 10$
$\Rightarrow x^{2} - 5 x + 6 < 0 \Rightarrow (x - 3) (x - 2) < 0$
$\Rightarrow 2 < x < 3 ... (ii)$
From Eqs. (i) and (ii), $x \in (2, 3)$
(C ) Let $y = {cos}^{- 1} . \frac{2}{2 + sin x}$ , for y to be defined
$- 1 \leq \frac{2}{2 + sin x} \leq 1 [\begin{matrix} ∵ - 1 < sin x \leq 1 1 < 2 + sin x \leq 3 \end{matrix}]$
Multiplying by (2+sinx)
$- (2 + sin x) \leq 2 \leq 2 + sin x$
$\Rightarrow - 2 - sin x \leq 2 ∣ 2 \leq 2 + sin x$
$\begin{matrix} \Rightarrow . - sin x \leq 4 \Rightarrow . sin x \geq - 4 \end{matrix} ∣ ∣ ∣ \begin{matrix} sin x \geq 0 2 n π \leq x \leq (2 n + 1) π . n \in z ... (i) \end{matrix}$
We know that sin $x \in [- 1, 1]$
$∴ x \in R ... (ii)$
From Eqs. (i) and (ii), $x \in [2 k π, (2 k + 1) π]$
Domain $\cup k \in I [2 k π, (2 k + 1 π)]$
(D) $y = \sqrt{sin x} + \sqrt{16 - x^{2}}$ for y to be defined
$\begin{matrix} sin x \geq 0 x \in [2 k π, (2 k + 1) π],k \in I ... (i) \end{matrix} ∣ ∣ ∣ \begin{matrix} 16 - x^{2} \geq 0 - 4 \leq x \leq 4 ... (ii) \end{matrix}$
From Eqs. (i) and (ii), we get
$x \in [- 4, - π] \cup [0, π]$

|

Class 12 MATHS SETS, RELATIONS AND FUNCTIONS

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile