If sum of two unit vectors is a unit vector; prove that the magnitude of their difference is $\sqrt{3}$ Solution in Gujrati

Video Solution

Text Solution

Verified by Experts

Let $\hat{a}$ and $\hat{b}$ be two unit vectors represented by sides OA and AB of a $Δ O A B$ .

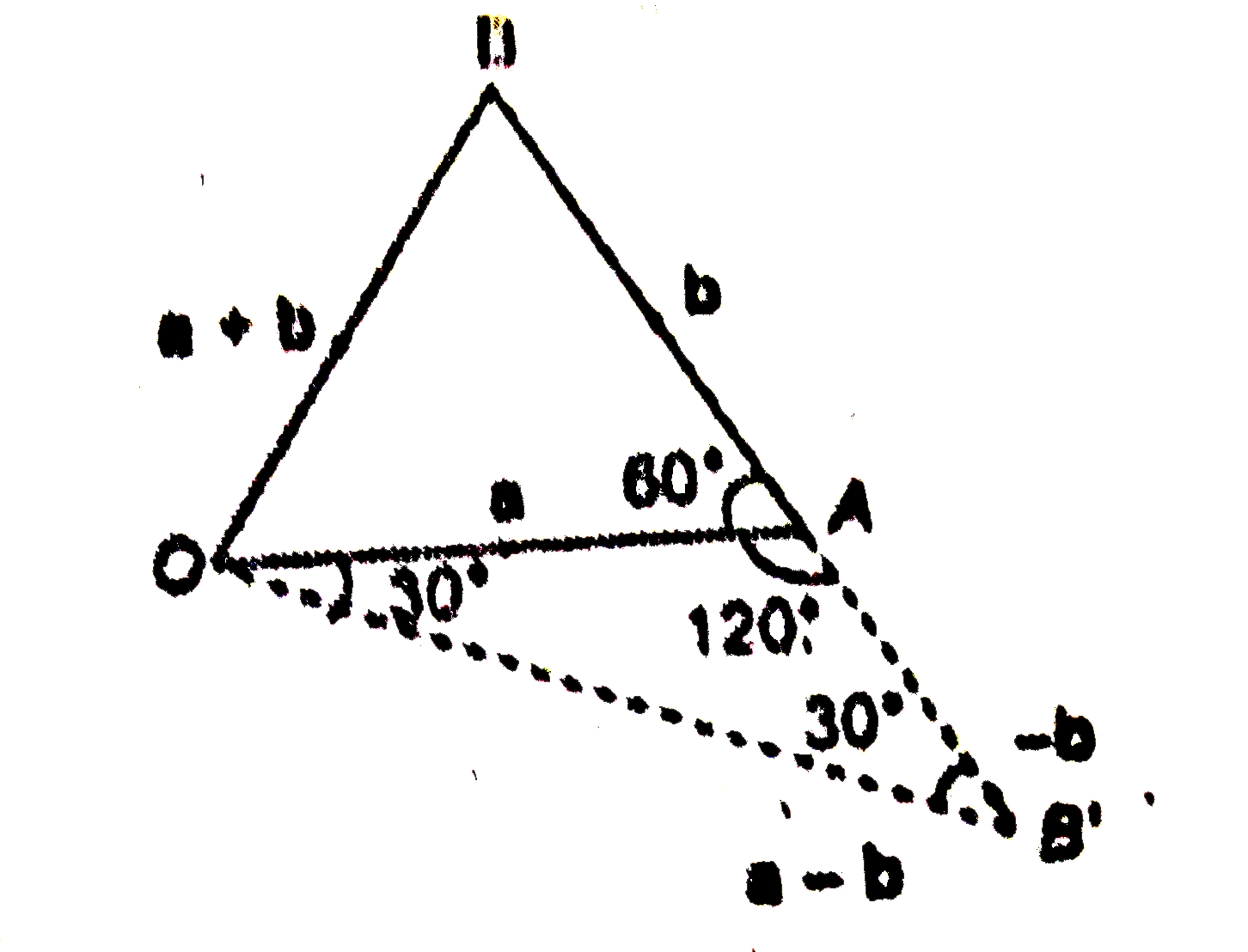
Then, $O A = \hat{a}, A B = \hat{b}$
$O B = O A + A B = \hat{a} + \hat{b}$ (using triangle law of vector addition)
It is given that, $| \hat{a} | = | \hat{b} | = | \hat{a} + \hat{b} | = 1$
$\Rightarrow | O A | + | A B | = | O B | = 1$
$Δ O A B$ is equilateral triangle.
Since, $| O A | = | \hat{a} | = 1 = | = | - \hat{b} | = | A B' ∣$
therefore, $Δ O A B'$ is a isosceles triangle.
$\Rightarrow ∠ A B' O = ∠ A O B' = 30^{\circ}$
$\Rightarrow ∠ B O B' = ∠ B O A + ∠ A O B' = 60^{\circ} + 30^{\circ} = 90^{\circ}$ (since, $Δ B O B'$ is right angled)
$∴$ In $Δ B O B'$ , we have
$| B B' | = {| O B |}^{2} + {| O B' |}^{2}$
$= {| \hat{a} + \hat{b} |}^{2} + {| \hat{a} - \hat{b} |}^{2}$
$2^{2} = 1^{2} + {| \hat{a} - \hat{b} |}^{2}$
$\hat{a} - \hat{b} ∣ = \sqrt{3}$ . . . hence proved.

|

Updated on:21/07/2023

Class 12 MATHS VECTOR ALGEBRA

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile