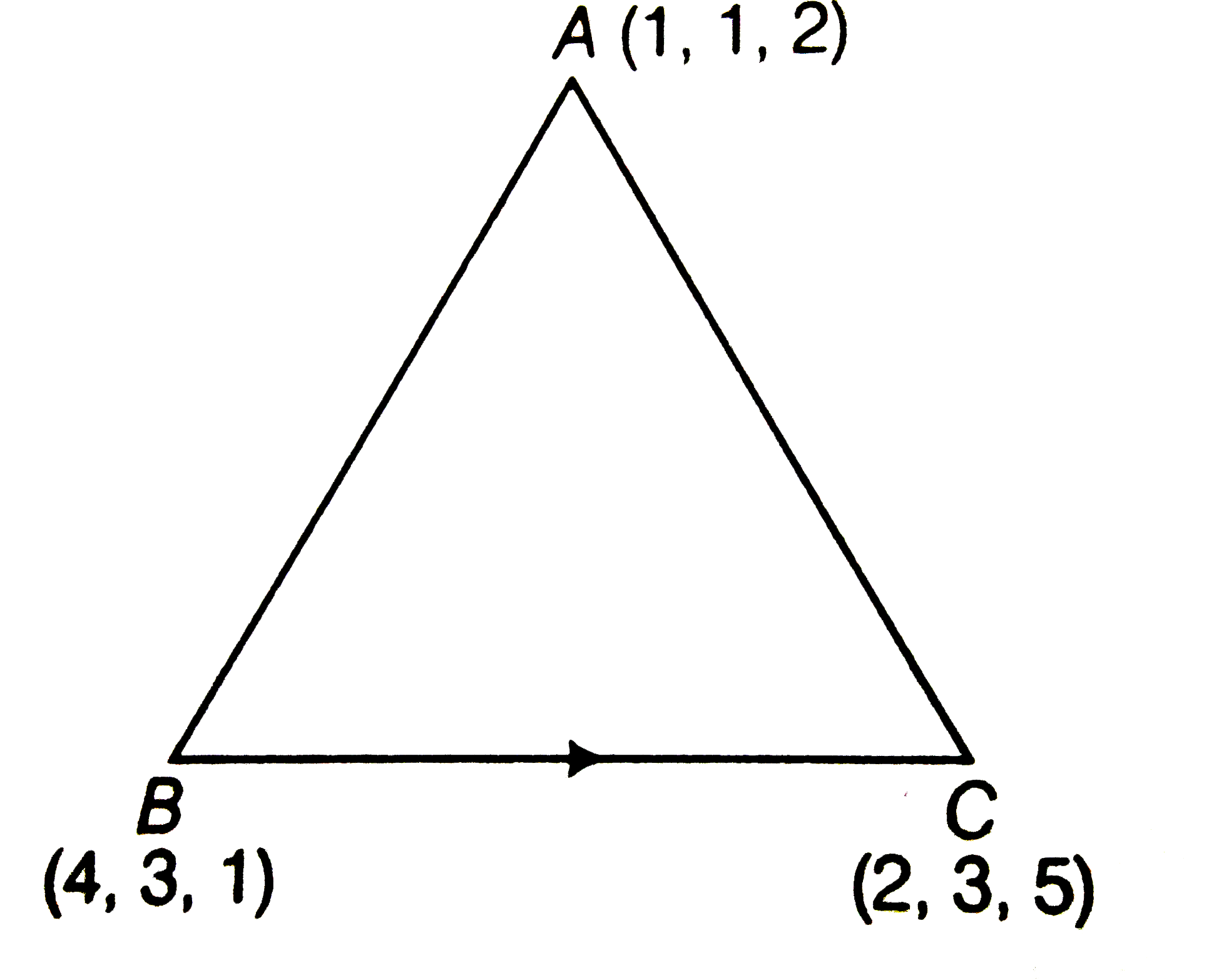
The vertices of a triangle are A(1,1,2), B (4,3,1) and C (2,3,5). The vector representing internal bisector of the angle A isSolution in Gujrati

A

$ˆ i + ˆ j + 2 ˆ k$

B

$2 ˆ i - 2 ˆ j j + ˆ k$

C

$2 ˆ i + 2 ˆ j + ˆ k$

D

none of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

From the figure, we have
$b = A C = ˆ i + 2 ˆ j + 3 ˆ k$
and $c = A B = 3 ˆ i + 2 ˆ j + ˆ k$

$∴$ Unit vector along the bisector of $∠ A$ is given by
$= \frac{b + c}{2} = \frac{(ˆ i + 2 ˆ j + 3 ˆ k) + (3 ˆ i + 2 ˆ j - ˆ k)}{\sqrt{14}}$
$= \frac{2 ˆ i + 2 ˆ j + ˆ k}{\sqrt{14}}$
$∴$ Any vector along the angle bisector of
$∠ A = 2 ˆ i + 2 ˆ j + ˆ k$ .

|

Updated on:21/07/2023

Class 12 MATHS VECTOR ALGEBRA

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile