Prove that the points (a, b), (c, d) and (a-c, b-d) are collinear, if ad = bc. Solution in Gujrati
Step by step video & image solution for Prove that the points (a, b), (c, d) and (a-c, b-d) are collinear, if ad = bc. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
In Figure, A, B, C and D, E, F are two sets of collinear points. Prove that AD∣∣CF
View SolutionIf a, b, c, d are positive numbers in HP then prove that ad>bc.
View SolutionIf a, b, c and d are four coplanr points, then prove that [abc]=[bcd]+[abd]+[cad].
View SolutionIf b+ca+d=bcad=3(b−ca−d) then a,b,c,d are in (A) H.P. (B) G.P. (C) A.P. (D) none of these
View SolutionShow that the points (a, b), (c, d) and (a - c, b - d) are collinear if ad = bc.
View SolutionIn Fig. 2.15, points A,B,C,D and E are collinear such that AB = BC = CD= DE. Then
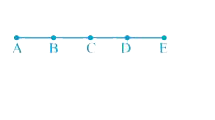
AD = AB + ________
View SolutionIn Fig. 2.15, points A,B,C,D and E are collinear such that AB = BC = CD= DE. Then
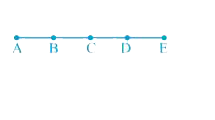
AD = AC + ___________
View SolutionProve that the points (a,b+c),(b,c+a)and(c,a+b) are collinear.
View Solution
