A particle of mass m is in a uni-directional potential field where the potential energy of a particle depends on the x-coordinate given by ϕx=ϕ0(1−cosax) & 'ϕ0' and 'a' are constants. Find the physical dimensions of 'a' & ϕ0.Solution in Gujrati
The correct Answer is:L−1,ML2T−2
Dimensions of ax=M∘L∘T∘
⇒[a]=M∘L∘T∘[L]=L−1 and [ϕ0]=[M1L2T−2]
Topper's Solved these Questions
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Exersice-4[B]
14 Videos
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - EXERCISE-5(A)
15 Videos
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - DATA SUFFICIENCY QUESTIONS
3 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLEN CHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-IV
7 Videos
Similar Questions
If Energy (E), velcity (v) and time (T) are fundamental units. What will be the dimension of surface tension ?
View SolutionIdentify the pair whose dimensions are equal
View SolutionWhat is the angle between →a and →b :
(i) Magnitude of →a and →b are 3 and 4 respectively.
(ii) Area of triangle made by →a and →b is 10.
View SolutionA football player kicks a ball at an angle of 30∘ with an initial speed of 20 m/s. Assuming that the ball travels in a vertical plane, calculate (a) the time at which the ball reaches the highest point (b) the maximum height reached (c) the horizontal range of the ball (d) the time for which the ball is in the air, (g=10m/s2)
View SolutionAssertion : Gravitational forces do not depend on the medium between the masses.
Reason : Mediating particle responsible for gravitational force is graviton.
View SolutionLet Phi_(n)=2^(2^(n))+1. Prove that if n
View SolutionPQ is a chord joining the points phi_(1) and phi_(2) on the hyperbola (x^(2))/(a^(2))-(y^(2))/(b^(2))=1. If phi_(1) and phi_(2)=2 alpha, wherealha is constant, prove that PQ touches the hyperbola (x^(2))/(a^(2))cos^(2)alpha-(y^(2))/(b^(2))=1
View SolutionThe graph between the stopping potential (V0)and(1λ) is shown in the figure ϕ1,ϕ2andϕ3 are work function , which of the following is //are correct
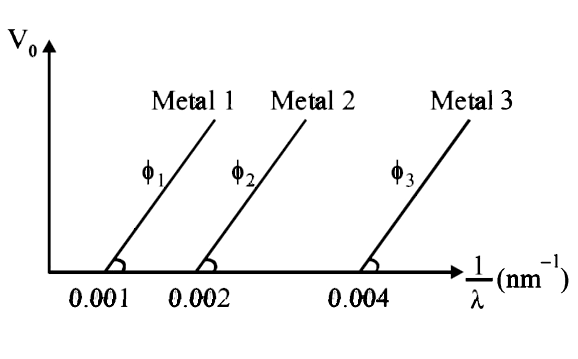
View SolutionIn the relation ( dy)/( dt) = 2 omega sin ( omega t + phi_(0)) , the dimensional formula for omega t + phi_(0) is
View SolutionA particles is in a unidirectional potential field where the potential energy (U) of a partivle depends on the x-coordinate given by Ux=k(1−cosax) & and 'a' are constant. Find the physical dimensions of 'a' & k.
View SolutionLet ϕ1(x)=x+a1,ϕ2(x)=x2+b1x+b2and
Δ=∣∣ ∣ ∣∣111ϕ1(x1)ϕ1(x2)ϕ1(x3)ϕ2(x1)ϕ2(x2)ϕ2(x3)∣∣ ∣ ∣∣, then
View Solutionε0dϕEdt की विमाएँ बताइए |
View SolutionDetermine the potential difference phi_(A)-phi_(B) between points A and B of the circuit
View SolutionDimensions of ε0dϕEdt are of :
View Solutionविद्युत क्षेत्र की तीव्रता का मात्रक एवं विमा लिखिए
View SolutionEquation for two waves is given as y1=asin(ωt+ϕ1),y2=asin(ωt+ϕ2).
If ampitude and time period of resultant wave does not change, then calculate (ϕ1−ϕ2).
View Solution
