The figure shows a system of two concentric spheres of radii r1 and r2 and kept at temperatures T1 and T2, respectively . The radial rate of flow of heat in a substance between the two concentric spheres, is proportional to :- _E01_132_Q01.png) Solution in Gujrati
Solution in Gujrati
(r2−r1(r1r2)
ln(r2r1)
r1r2(r2−r1)
(r2−r1)
The correct Answer is:C
Rate of flow of heat =KAΔTΔx=K(4πr1r2)(T1−T2r2−r1)
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLEN CHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 05 (B)
58 Videos
- GEOMETRICAL OPTICSBOOK - ALLEN CHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 05 (B) (MCQ)
9 Videos
- GEOMETRICAL OPTICSBOOK - ALLEN CHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 04 (B)
15 Videos
- CURRENT ELECTRICITYBOOK - ALLEN CHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
- GRAVITATIONBOOK - ALLEN CHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
A vessel contains a mixture of one mole of oxygen and two moles of nitrogen at 300K. The ratio of the average rorational kinetic energy per O2 molecules to that per N2 molecules is
View SolutionLet ¯v,vrms and vp respectively denote the mean speed, the root-mean - square speed, and the most probable speed of the molecules in an ideal monoatomic gas at absolute temperature T. The mass of a molecule is m:-
View SolutionThe rectangular box shown in Fig has partition which can slide without friction along the length of the box. Initially each of the two chambers of the box has one mole of a mono-atomic ideal gas (λ=5/3) at a pressure P0, volume V0 and temperature T0. The chamber on the left is slowly heated by an electric heater. The walls of the box and the lead wires of the heater is negligible. The gas in the left chamber expands pushing the partition until the final pressure in both chambers becomes 243P0/32. Determine (i) the final temperature of the gas in each chamber and (ii) the work done by the gas in the right chamber.
View SolutionIn the Bohr's model of hy drogen atom, the electron moves around the nucleus in a circular orbit of radius 5×10−11m. Its time period is 1.5×10−16s. The current associated with the electron motion is:-
View SolutionAssertion : The difference in the value of acceleration due to gravity at pole and equator is proportional to square of angular velocity of earth.
Reason : the value of acceleration due to gravity is minimum at the equator and maximum at the pole.
View SolutionThe figure shows a system of two concentric spheres of radii r1andr2 are kept at temperature T1andT2, respectively. The radial rate of flow of heat in a substance between the two concentric spheres is proportional to
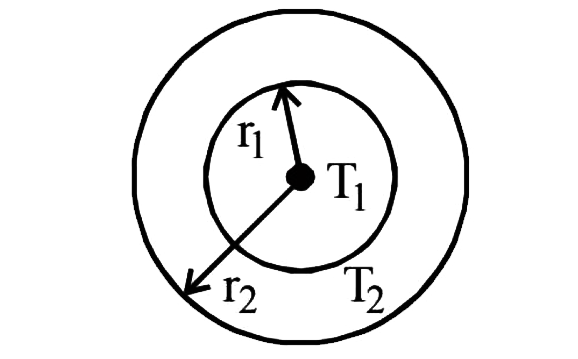
View SolutionFig, shows a system of two concentric spheres of radii r_(1) and r_(2) and kept at temperature T_(1) and T_(2) (T_(1) gt T_(2)) respectively. Find the expression for radial rate of flow of heat through the substance.
View SolutionTwo spherical black bodies of radii R1 and R2 and with surface temperature T1 and T2 respectively radiate the same power. R1/R2 must be equal to
View SolutionConducting sphere of radius R1 is covered by concentric sphere of radius R2. Capacity of this combination is proportional to
View SolutionA binary star system consists of two stars of masses M1 and M2 revolving in circular orbits of radii R1 and R2 respectively. If their respective time periods are T1 and T2, then
View SolutionFigure shows a system of two concentric spheres of radii r_(1)" and "r_(2) at the temperatures T_(1)" and "T_(2) , respectively. The radial rate of flow of heat in a substance filled between the two concentric spheres is proportional to
View SolutionTwo spherical black bodies of radii r1 and r2 and with surface temperatures T1 and T2 respectively, radiate the same power. Then r1/r2 must be equal to
View SolutionIn the following picture r_(1) And r_(2) A body of two concentric circles of radii is shown, respectively. T_(1) And T_(2) Is placed on the heat. The rate of spatial flow of heat in the material between the two concentric spheres is proportional.
View SolutionA charge Q has been divided on two concentric conducting spheres of radii R1 and R2(R1>R1) such that the surface charge densities on both the spheres are equal. The potential at their common centre is
View SolutionTwo concentric metallic shells are of radii r1andr2(r2>r1). If charge given to outer sphere is q. and the inner sphere is ground. Then the charge on the inner sphere q1 is

View SolutionTwo spheres of radii r_(1) and r_(2) have densities rho_(1) and rho_(2) and spheats C_(1) and C_(2) respectively . If they are heated to same temperature, the ratio of their rates of fall of temperature in the same surroundings will be
View Solution
