A particle is moving in a circular path. The acceleration and moment of the particle at a certain moment are . The motion of the particle isSolution in Gujrati
(a)uniform circular motion
(b) accelerated circular motion
(c)de-accelerated circular motion
(d)we can not say anything with a and p only
The correct Answer is:B
Step by step video & image solution for A particle is moving in a circular path. The acceleration and moment of the particle at a certain moment are a=(4 hat(i)+3hat(j))m//s^(2) and p=(8 hat(i)-6hat(j))"kg-m/s". The motion of the particle is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - ALLEN CHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-M)
5 Videos
- CIRCULAR MOTIONBOOK - ALLEN CHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-A)
6 Videos
- CIRCULAR MOTIONBOOK - ALLEN CHAPTER - CIRCULAR MOTIONEXERCISE - Martrix match
1 Videos
- BASIC MATHSBOOK - ALLEN CHAPTER - BASIC MATHSEXERCISE - Question
1 Videos
- CURRENT ELECTRICITYBOOK - ALLEN CHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
Similar Questions
For a particle in uniform circular motion , the acceleration →a at a point p(R,θ) on the circle of radiu R is ( Here θ is measured from the x−aξs )
View SolutionA ball of mass (m)0.5kg is attached to the end of a string having length (L)0.5m. The ball is rotated on a horizontal circular path about vertical axis. The maximum tension that the string can bear is 324N. The maximum possible value of angular velocity of ball (radian/s) is -
 .
.
View SolutionA block is placed on a horizontal table which can rotate about its axis.The block is placed at certain distance from centre as shown in figure. Table rotates such that particle does not slide. See possible direction of net acceleration of block at the instant shown in figure
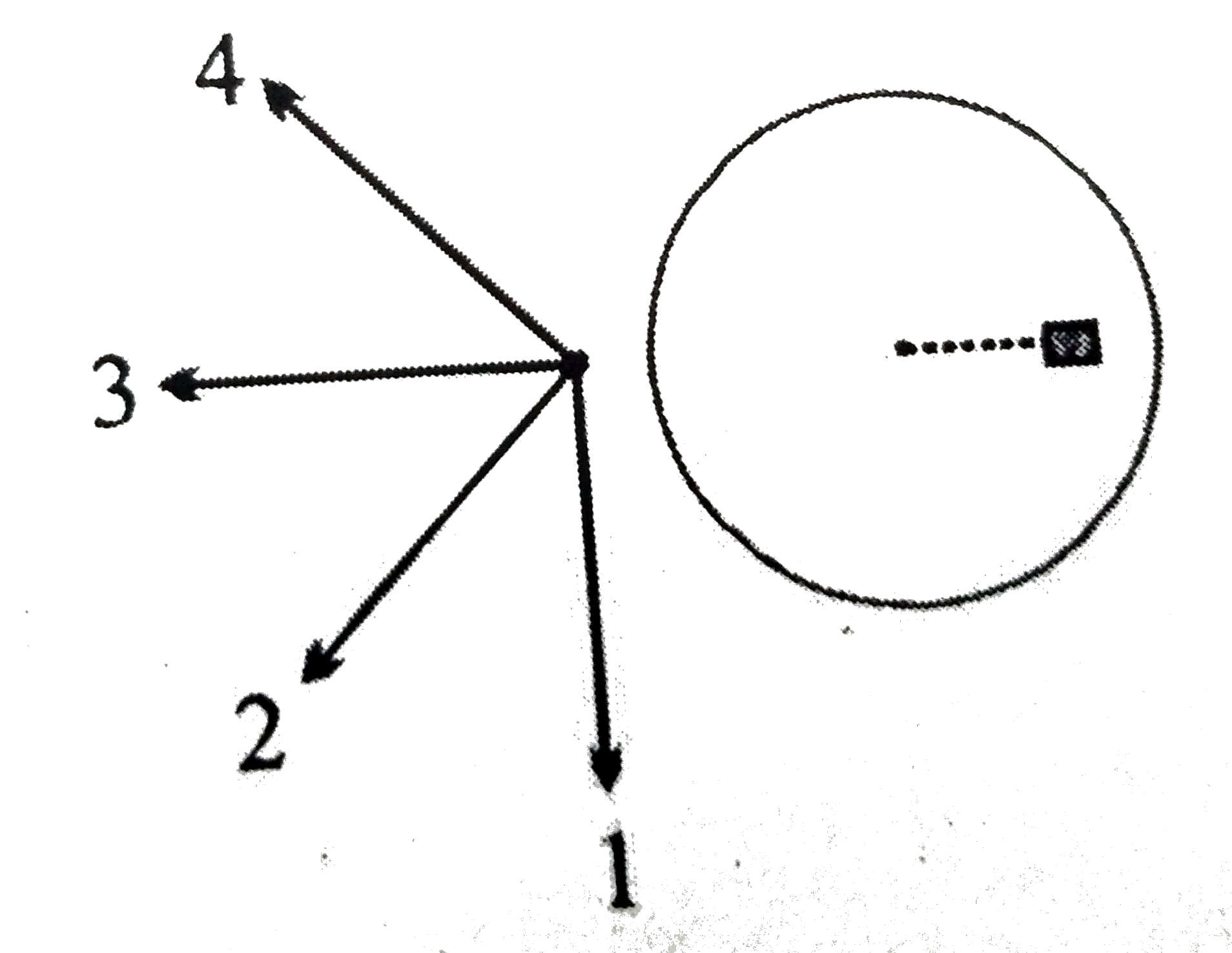
Column-IColumn-II(A)When rotation is clockwise with constant ω(P)1(B)When rotation is clock wise with decreasing ω(Q)2(C)When rotation is clockwise with increasing ω(R)3(D)Just after clockwise rotation beings from rest(S)4
View SolutionFind dydx for the following :
(i) y=x7/2 (ii) y=x−3
(iii)y=x (iv) y=x5+x3+4x1/2+7
(v) y=5x4+6x3/2+9x (vi) y=ax2+bx+C
(Vii)y=3x^(5)-3x-1/x
View SolutionIn the Bohr's model of hy drogen atom, the electron moves around the nucleus in a circular orbit of radius 5×10−11m. Its time period is 1.5×10−16s. The current associated with the electron motion is:-
View SolutionAt a particular instant velocity and acceleration of a particle are (−ˆi+ˆj+2ˆk)m/s and (3ˆi−ˆj+ˆk)m/s2 respectively at the given instant particle's speed is :
View SolutionA particle is moving with a constant acceleration vec(a) = hat(i) - 2 hat(j) m//s^(2) and instantaneous velocity vec(v) = hat(i) + 2 hat(j) + 2 hat(k) m//s then rate of change of speed of particle 1 second after this instant will be :
View SolutionA particle is moving in x-y plane. At an instant, it has velocity (4ˆi+4ˆj)m/s and acceleration (3ˆi+5ˆj)m/s2 At that instant, the radius of curvature of its path will be :
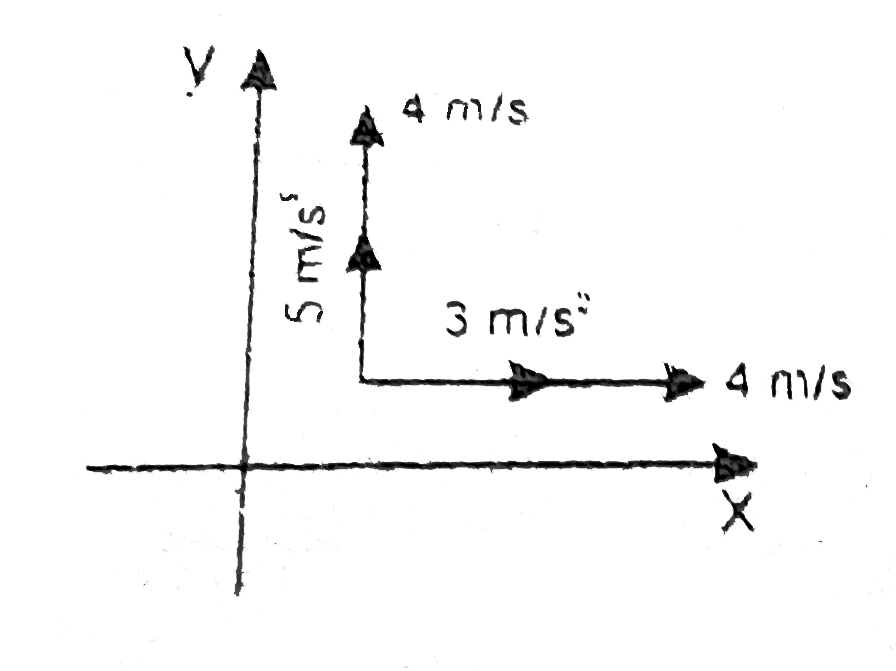
View SolutionThe velocity and acceleration vectors of a particle undergoing circular motion are v=2ˆim/sanda=2ˆi+4ˆjm/s2 respectively at some instant of time. The radius of the circle is
View SolutionA particle is moving in a circular path. The acceleration and momentum of the particle at a certain moment are a=(4 hat(i)+3hat(j))m//s^(2) and p=(8 hat(i)-6hat(j))"kg-m/s" . The motion of the particle is
View SolutionA particle starts with an initial veloctiy (ˆi+ˆj)m/s and changes it to (3ˆi−5ˆj)m/s in 2 sec. What is the magnitude of displacement of the particle in 2s, if the acceleration is constant ?
View SolutionA force 3hat i+4 hat j - 5 hatk N is acting on a particle. If the velocity of particle at an instant is 2hati + 2 hat j + 2 hatk m/s , find the instantaneous power developed by the force (in watts).
View SolutionA particle has an initial velocity of 3ˆi+4ˆj and an A A acceleration of 0.4ˆi+0.3ˆj . Its speed after 10 s is :
View SolutionA particle is moving in a circular path. The acceleration and momentum vector at an instant of time are →a=2ˆi+3ˆjm/sec2 and →p=6ˆi−4ˆjkgm/sec. Then the motion of the particle is
View SolutionA particle of mass m moving with a velocity (3hat(i) + 2hat(j))m//s collides with sationary body of mass M and finally moves with velocity (-2hat(i) + hat(j))m//s . Then :
View SolutionA particle is rotating about a fixed axis with angular acceleration vec alpha = (3hat i +hat j +hat k) rad/s^2 . The tangential acceleration of a point having radius vector ( 2 hat i-hat j -hat k) m from axis of rotation is (in m/s^2 )
View Solution
