A boat can be rowed at 5 m/s on still water. It is used to cross a 200m wide river from the south bank to the north bank. The river current has uniform velocity of 3 m/s due east.
(a) In which direction must it be steered to cross the river perpendicular to current ?
(b) How long will it take to cross the river in a direction perpendicular to the river flow ?
(c) In which direction must the boat be steered to cross the river in minimum time ? How far will it drift ?Solution in Gujrati
(a) Velocity of a boat on still water is its capacity to move on water surface and equals to its velocity relative to water. →vb/w= Velocity of boat relative to water = Velocity of boat on still water
On flowing water, the water carries the boat along with it. Thus velocity →vb of the boat relative to the ground equals to vector sum of →vb/wand→vw. The boat crosses the river with the velocity →vb.
→vb=→vb/w+→vw
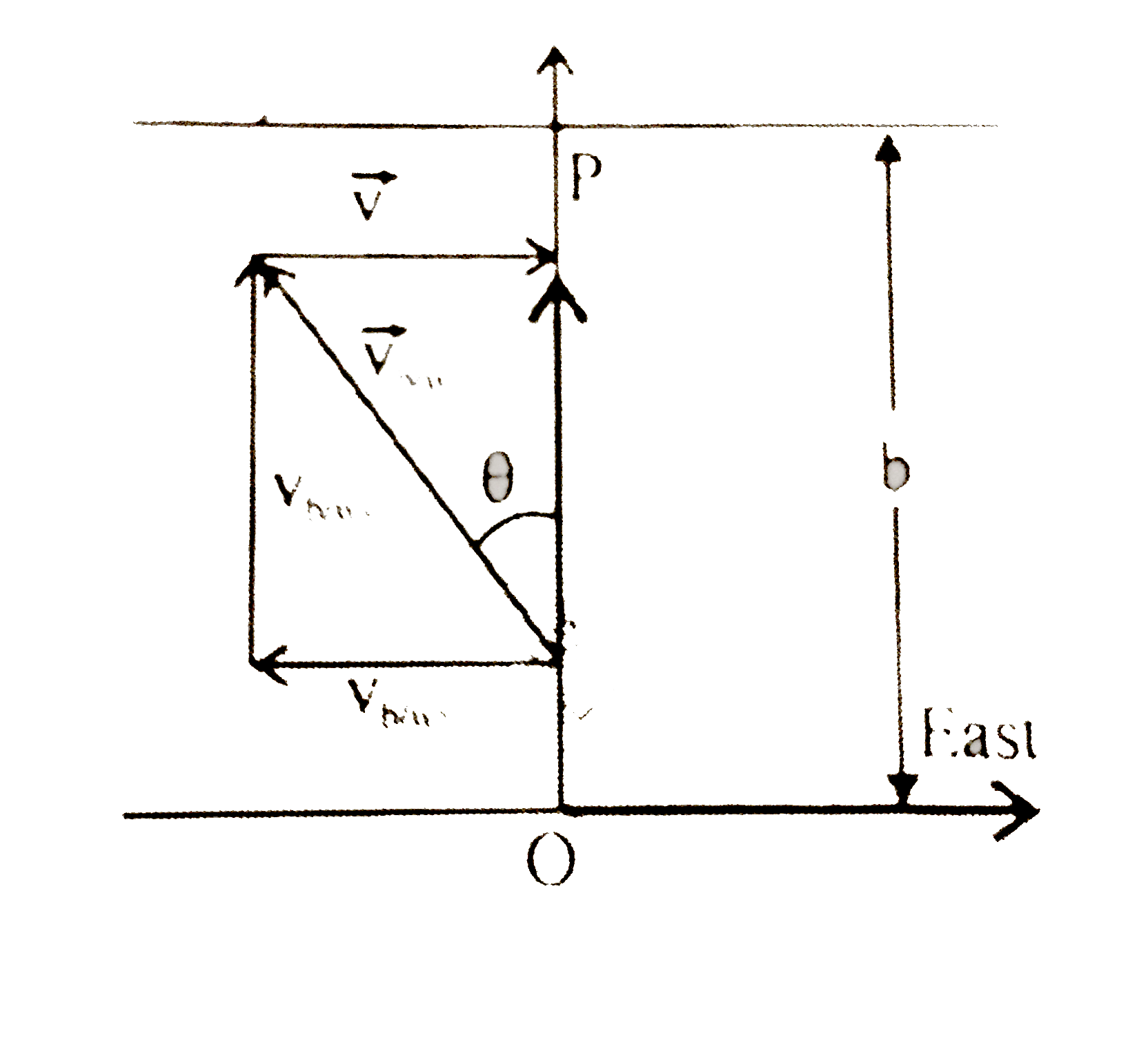
(b) To cross the river perpendicular to current the boat must be steered in a direction so that one of the components of its velocity (→vb/w) relative to water becomes equal and opposite to water flow velocity →vw to neutralize its effect. It is possible only when velocity of boat relative to water is greater than water flow velocity. In the adjoining figure its is shown that the boat starts from the point O and moves along the line OP (y-axis) due north relative to ground with velocity →vb. To achieve this it is steered at an angle θ with the y-axis.
vb/wsinθ=vw→5sinθ=3⇒θ=37∘
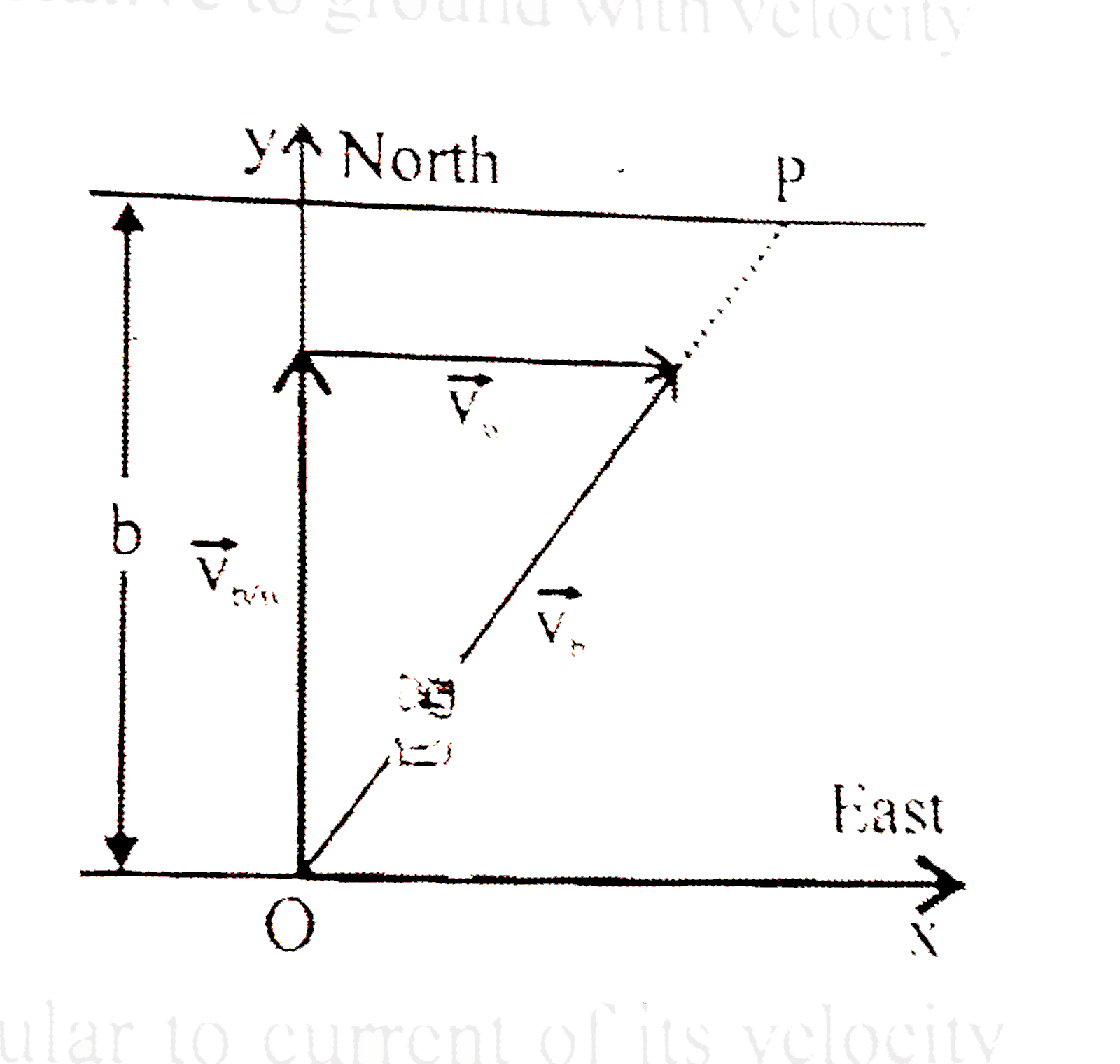
(c) The boat will cover river width b with velocity
vb=vb/wt=vb/wsin37∘=4m/s in time t, which is given by t=b/vb→t=50s
(d) To cross the river in minimum time, the component perpendicular to current of its velocity relative to ground must be kept to maximum value. It is achieved by steering the boat always perpendicular to current as shown in the adjoning figure. The boat starts from O at the south bank and reaches point P on the north bank. Time t taken by the boat is given by
t=b/vb/w→t=40s
Drift is the displacement along the river current measured from the starting point. Thus, it is given by the following equation. We denote it by xd
xd=vbxt
Substituting vbx=vw=3m/s, from the figure, we have
xd=120m
Topper's Solved these Questions
- KINEMATICS-2DBOOK - ALLEN CHAPTER - KINEMATICS-2DEXERCISE - Exercise (S-1)
30 Videos
- KINEMATICS-2DBOOK - ALLEN CHAPTER - KINEMATICS-2DEXERCISE - Exercise (S-2)
14 Videos
- GRAVITATIONBOOK - ALLEN CHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
- MOTION IN A PALNEBOOK - ALLEN CHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE
28 Videos
Similar Questions
The vertical height y and horizontal distance x of a projectile on a certain planet are given by x=(3t)m,y=(4t−6t2) m where t is in seconds, Find the speed of projection (in m/s).
View SolutionA particle travels so that its acceleration is given by →a=5costˆi−3sintˆj. If the particle is located at (−3,2) at time t=0 and is moving with a velocity given by (−3ˆi+2ˆj). Find
(i) The velocity [→v=∫→a.dt] at time t and
(ii) The position vector [→r=∫→v.dt] of the particle at time t(t>0).
View SolutionAssertion : The difference in the value of acceleration due to gravity at pole and equator is proportional to square of angular velocity of earth.
Reason : the value of acceleration due to gravity is minimum at the equator and maximum at the pole.
View SolutionA particle thrown over a triangle from one end of a horizontal base falls on the other end of the base after grazing the vertex. If αandβ are the base angles of triangle and angle of projection is θ, then prove that (tanθ=tanα+tanβ)
View SolutionA man who can swin at the rate of 2 (km)/hr) (in still river) crosses a river to a point exactly opposite on the other bank by swimming in a direction of 120^@ to the flow of the water in the river. The velocity of the water current in (km)/(hr) is
View SolutionThe speed of river current close to banks is nearly zero. The current speed increases linearly from the banks to become maximum (= V_(0)) in the middle of the river. A boat has speed ‘u’ in still water. It starts from one bank and crosses the river. Its velocity relative to water is always kept perpendicular to the current. Find the distance through which the boat will get carried away by the current (along the direction of flow) while it crosses the river. Width of the river is l.
View SolutionThe river 500 m wide is flowing with a current of 4kph. A boat starts from one bank of the river wishes to cross the river at right angle to stream direction. Boatman can row the boat at 8 kph. In which direction he should row the boat. What time he ''ll take to cross the river ?
View SolutionBoat moves with velocity 5 m/s on still water. It is steered perpendicular to the river current.
(a) Will it reach point B or somewhere else on the other bank ?
(b) How long will it take to cross the river?
(c) How far down stream, will it reach the other bank ?
(d) Does it take minimum time in this way ?
View SolutionA boat had to cross a river. It crosses the river by making an angle of 60∘ with the bank of the river due to the stream of the river and travel a distance of 600 m to reach the another side of the river. What is the width of the river ?
View SolutionA swimmer crosses the river along the line making an angle of 45^(@) with the direction of flow. Velocity of the river is 5 m/s. Swimmer takes 6 seconds to cross the river of width 60 m. The velocity of the swimmer with respect to water will be :
View SolutionA 400 m wide river is flowing from west to east at velocity 3m/s. A boat starts from one bank and it crosses the river along the shortest path. When it reaches the middle of river, wind starts blowing horizontally along north-east at a velocity √2 m/s . Due to this, the direction of rowing of boat has to be changed for the boat to move along the shortest path. If the velocity of boat in still water with no wind blowing is 5 m/s, then choose the correct option(s).
View SolutionVelocity of boat in still water is 5m/s. It crossed river of 60 m wide, with 127∘ angle always to the river flow. If the velocity of the river is 3.5m/s the drift obtained by boat in the river on reaching opposite bank is (tan37∘=3/4)
View SolutionThe speed of a boat in still water is 5km / h. The boat crosses the 1km wide river horizontally in 15min. What is the current of the river?
View SolutionVelocity of a boat in still water is 5km*h^(-1) . It takes 15 min to cross a river along the width. The river is 1km wide. Find the velocity of current.
View SolutionA river is 150 m wide and has a current of 1 m*s^(-1) . A boat is moving directly across the river at a speed of 2 m*s^(-1) . How long will the boat take to cross the river ?
View SolutionA 200m - wide river flows due east at a uniform speed of 2.0 m//s . A boat with a speed of 8.0 m/s relative to the water leaves the south bank pointed in a direction 30^@ west of north . What are the (a) magnitude and (b) direction of the boat's velocity relative to the ground ? ( c) how long does the boat take to cross the river ?
View Solution
