The dimension of torque are Solution in Gujrati
[ML3L−3]
[ML−1T−1]
[ML2T−2]
[ML−2]
The correct Answer is:C
Torque →τ=→r×→F
Dimensional for torque →t=[L1][M1L1T−2]
=[M1L2T−2]
Topper's Solved these Questions
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-I ERRORS
18 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-I MEASUREMENT
4 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-I UNIT
12 Videos
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Question
1 Videos
- SEMICONDUCTORSBOOK - ALLEN CHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)
50 Videos
Similar Questions
A quantity is represented by X=maLbTc. The percentage error in measurement of M,LandTarealpha %, beta %andgamma% respectively. The percentage erro in X would be
View SolutionIn a vernier calliper, N divisions of vernier scale coincide with (N-1) divisions of main scale in which 1 division represents 1 mm. The least count of the instrument in cm, should be
View SolutionWhich of the following system of units is not based on units of mass, length and time alone
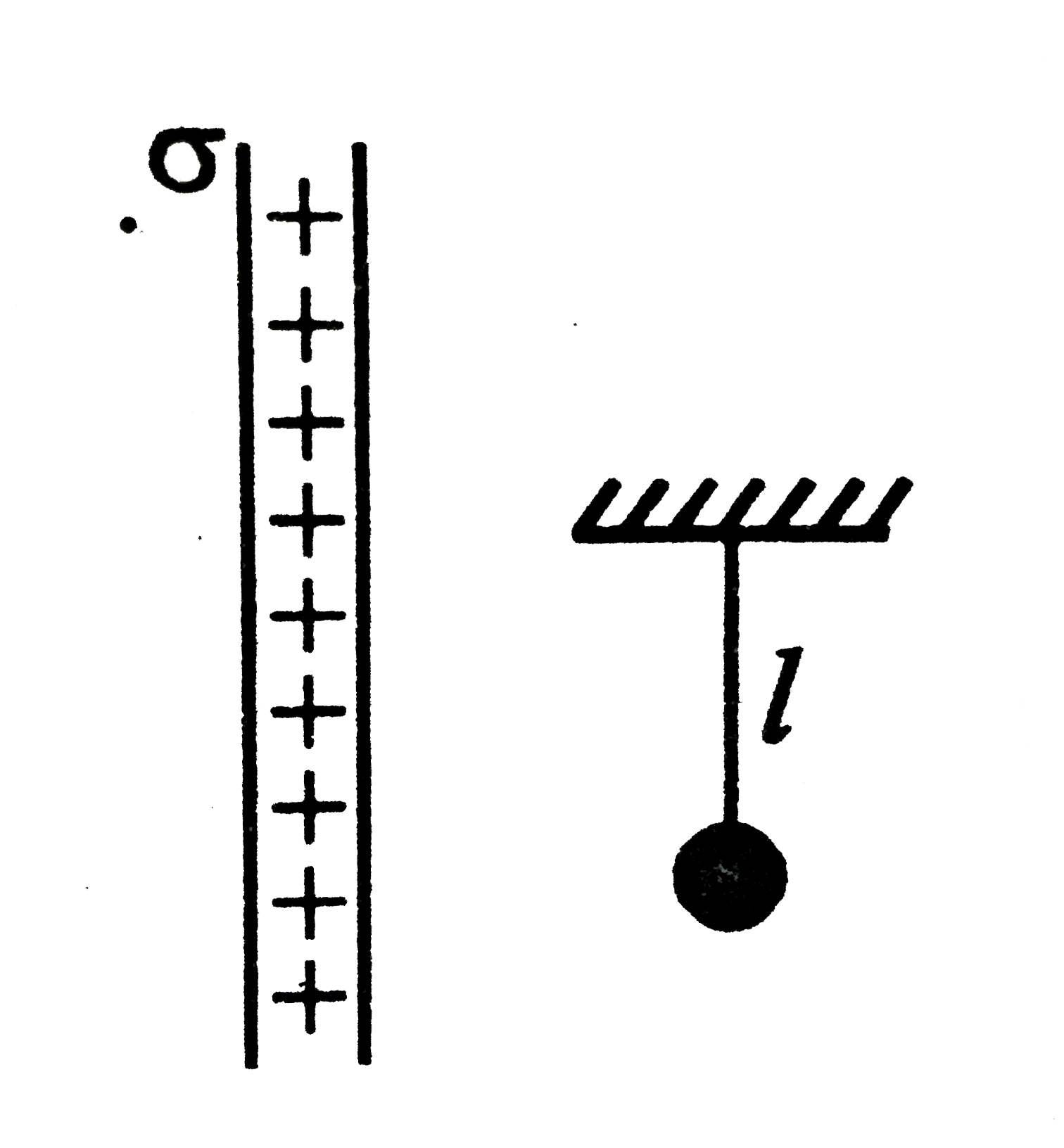
View SolutionA simple pendulum of length l and bob mass m is hanging in front of a large nonconducting sheet having surface charge density σ. If suddenly a charge +q is given to the bob & it is released from the position shown in figure. Find the maximum angle through which the string is deflected from vertical .
 .
.
View SolutionAt absolute zero temperature Si acts as ……..
View SolutionThe dimension of torque
View SolutionThe dimensions of torque are :
View SolutionThe dimensions of torque are :
View SolutionThe dimensions of torque are same as that of
View SolutionThe dimensions of torque are the same as that of
View SolutionThe dimension of torque is
View SolutionThe dimension of torque is
View SolutionWrite down the dimension of torque.
View SolutionThe dimensions of power divided by torque is equal to the dimensions of angular ........
View SolutionDerive the dimension of torque.
View SolutionThe dimension for Torque is
View Solution
