If E is the electric field intensity ε0 is the permitivity of free space, then find dimensions of quantity ε0E2. Solution in Gujrati
[MLT−1]
[ML2T−2]
[ML−1T−2]
[ML2T−1]
The correct Answer is:C
12∈0E2 = Energy density = EnergyVolume=[M1L−1T−2]
Topper's Solved these Questions
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-II AIPMT (MAINS) 2010
1 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-II AIIMS 2010
1 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-II AIPMT 2009
1 Videos
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Question
1 Videos
- SEMICONDUCTORSBOOK - ALLEN CHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)
50 Videos
Similar Questions
A student meaures te distance traversed in free fall of a body, initially at rest in a given time. He uses this data to estimate g, the acceleration due to gravity. If the maximum percentage errors in measurement of the distance and the time are e1 and e2 respectively, the percentage error in the estimation of g is-
View SolutionDimension of magnetic permeability is :
View SolutionIf the dimensions of a physical quantity are given by MaLbTc. Then the physical quantity will be:
View SolutionA simple pendulum of length l and bob mass m is hanging in front of a large nonconducting sheet having surface charge density σ. If suddenly a charge +q is given to the bob & it is released from the position shown in figure. Find the maximum angle through which the string is deflected from vertical .
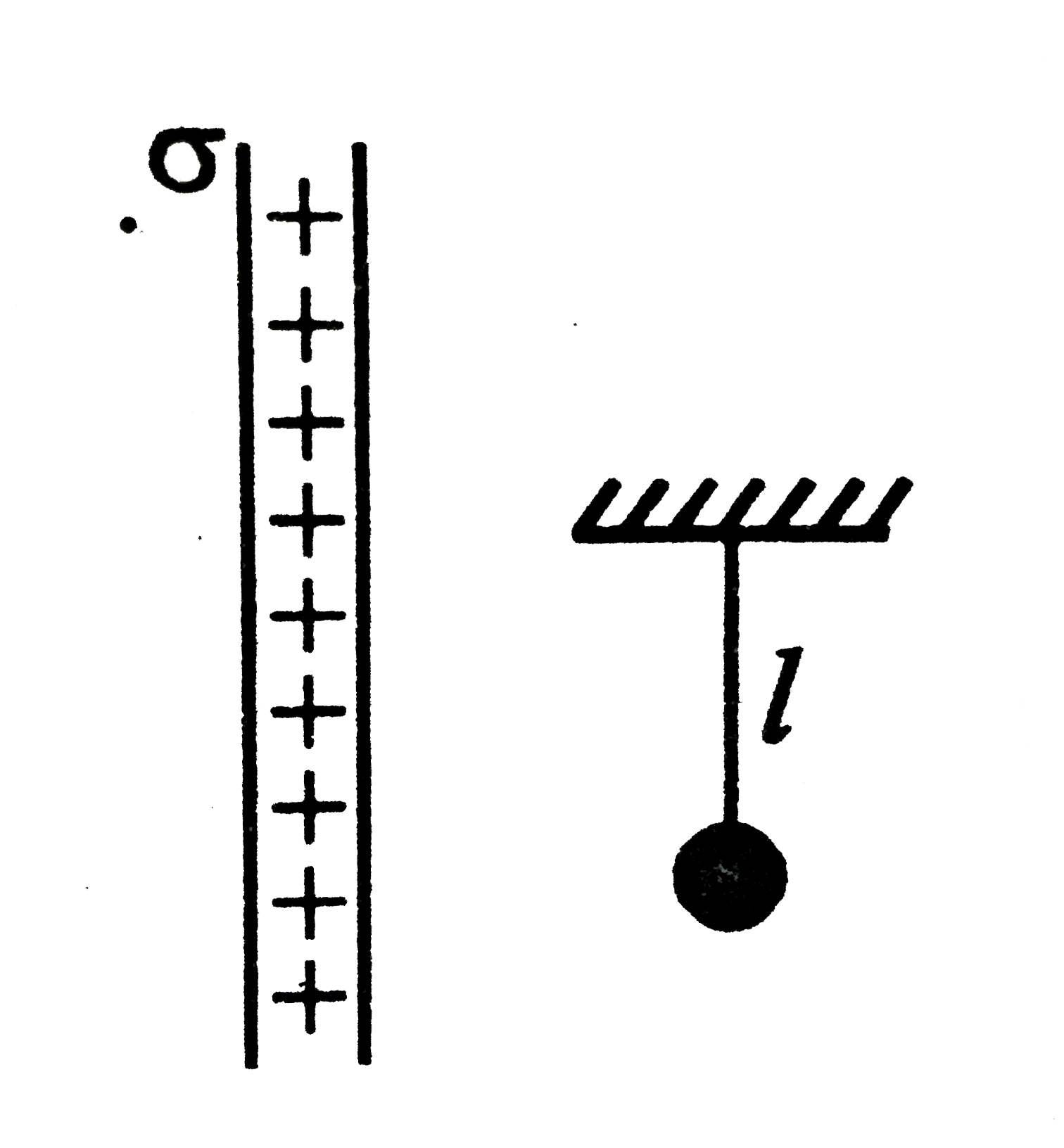 .
.
View SolutionAt absolute zero temperature Si acts as ……..
View SolutionThe dimension of (12)ε0E2 (ε0 : permittivity of free space, E electric field
View SolutionIf L has the dimensions of length, V that of potential and epsilon_(0) is the permittivity of free space then quantity epsilon_(0) LV has the dimensions of
View SolutionThe dimensions of 12ε0E2(ε0= permittivity of free space, E= electric field) is
View Solutionepsilon_(0)E^(2) has the dimensions of ( epsilon_(0)= permittivity of free space, E= electric field) Here k= Boltzmann consant T= absolute temperature R= universal gas constant.
View SolutionFind the dimension of the quantity (1)/(4pi epsilon_(0))(e^(2))/(hc) , the letters have their usual meaning , epsilon_(0) is the permitivity of free space, h, the Planck's constant and c, the velocity of light in free space.
View Solution12ε0E2 की विमा लिखिए (जहाँ ε0 मुक्त स्थाक की वैघुतशीलता तथा E वैघुत क्षेत्र की तीव्रता हैं।
View SolutionThe dimensions of 12∈0E2, where ∈0 is permittivity of free space and E is electric field, is :-
View SolutionThe dimensions of (1)/(2)epsilon_(0)E^(2) (epsilon_(0)= permitivity of free space and E = electric field) are :
View SolutionIf L has the dimensions of length, V that of potential and ε0 is the permittivity of free space then quantity ε0LV have the dimensions of :
View SolutionThe unit of permitivity of free space epsilon_(0) is
View SolutionThe dimension of (1)/(2) epsilon_(0)E^(2) . Where epsilon_(0) is permittivity of free space and E is electric field is
View Solution
