Suppose refractive indes is given as , were A and B are constants and is wavelenght then the dimension of B are same as that of Solution in Gujrati
wavelength
pressure
area
volume
The correct Answer is:C
Refractive index,
Since is dimensionless, hence is dimensionless.
Dimension of B will have the dimension of .
Hence B has a dimension of area.
Topper's Solved these Questions
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-IV
7 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-II AIIMS 2016
2 Videos
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Question
1 Videos
- SEMICONDUCTORSBOOK - ALLEN CHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)
50 Videos
Similar Questions
Assertion : Gravitational forces do not depend on the medium between the masses.
Reason : Mediating particle responsible for gravitational force is graviton.
View SolutionThe dimensional formula of Polarization P is ______ .
View SolutionA simple pendulum of length l and bob mass m is hanging in front of a large nonconducting sheet having surface charge density σ. If suddenly a charge +q is given to the bob & it is released from the position shown in figure. Find the maximum angle through which the string is deflected from vertical .
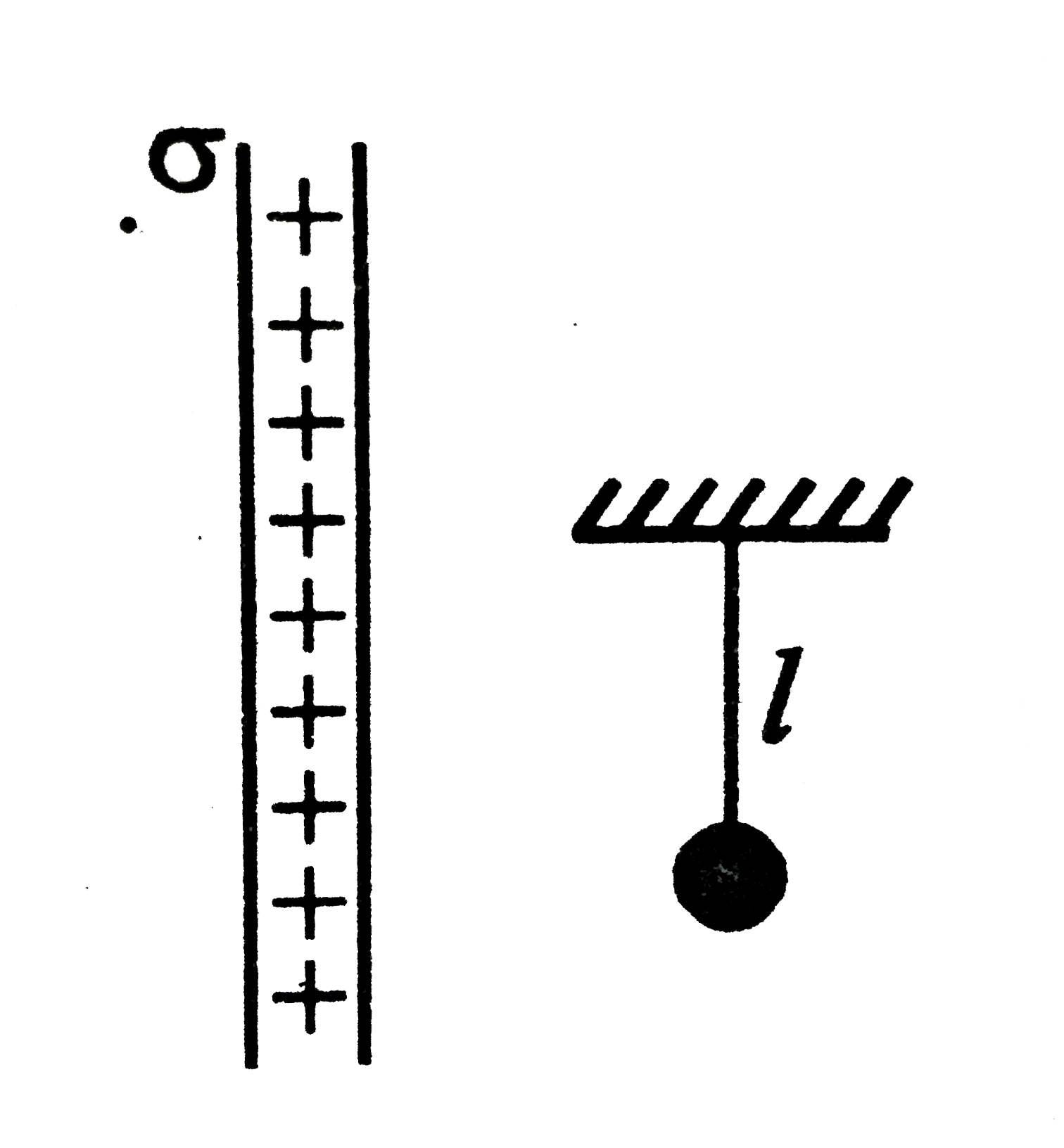 .
.
View SolutionAt absolute zero temperature Si acts as ……..
View SolutionThe position vectors of three points are 2a−b+3c,a−2b+λc and μa−5b where a,b,c are non-coplanar vectors. The points are collinear when (A) λ=−2,μ=94 (B) λ=−94,μ=2 (C) λ=94,μ=−2 (D) none of these
View SolutionLet a,b,c are sides and R is the circumradius of a triangle ABC such that R(b+c)=a sqrt(bc) if B+C=(lambda pi)/(mu),lambda,mu in N and are coprime then lambda+mu is
View SolutionRefractive index mu is given as mu=A+B/lambda^2, where A and B are constants and lambda is wavelength, then dimensions of B are same as that of
View SolutionThe refractive index mu of a medium is found to vary with wavelength lambda as mu = A +(B)/(lambda^2). What are the dimensions of A and B?
View Solutionμ=A+Bλ+Cλ2 si dimensionally correct. The dimensions of A,B and C respectively are (μ,A,B,C are constant) where λ is wave length of wave
View SolutionFind the values of λ ad μ if the points A(−1,4,−2),B(λ,μ,1) and C(0,2,−1) are collinear.
View SolutionIf the points A(−1,3,λ),B(−2,0,1)andC(−4,μ,−3) are collinear, then the values of λ and μ are
View SolutionThe refractive index of a medium is given by mu=A+(B)/(lambda^(2)) where A and B are constants and lambda is the wavelength of light. Then the dimensions of B are the same as that of
View SolutionThe refractive index ( mu ) of glass is related to the wavelength (lambda) of incident light as mu = A +(B)/(lambda^(2)) , where A and B are constants. Find out the dimensions of A and B.
View SolutionHow does refractive index (mu) of a material vary with respect to wavelenght (lambda) . (A and B are constants).
View SolutionSuppose refractive index μ is given as μ=A+Bγ2 where A and B are constants and γ is wavelength, then dimensions of B are same as that of
View Solutionमाना कि अपवर्तनांक । समीकरण μ=A+B/λ2 द्वारा दिया जाता है जहाँ A एवं B नियतांक है तरंगदैर्ध्य λ की विमा समान होगी
View Solution
