Assertion: If x and y are the distances along x and y axes respectively then the dimensions of
d3ydx3 is M0L−2T0
Reason: Dimensions of ∫baydx is M0L2T0Solution in Gujrati
If both Assertion & Reason are True & Reason is a correct explanation of the Assertion.
If both Assertion & Reason are True but Reason is not a correct explanation of the Assertion.
If Assertion is True but the Reason is False.
If both Assertion & Reason are false.
Step by step video & image solution for Assertion: If x and y are the distances along x and y axes respectively then the dimensions of (d^(3)y)/(dx^(3)) is M^(0)L^(-2)T^(0) Reason: Dimensions of int_(a)^(b) ydx is M^(0)L^(2)T^(0) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-III
20 Videos
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Question
1 Videos
- SEMICONDUCTORSBOOK - ALLEN CHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)
50 Videos
Similar Questions
In a particular system the units of length, mass and time are chose to be 10 cm, 10 g and 0.1 s respectively. The unit of force in this system will be equal to :-
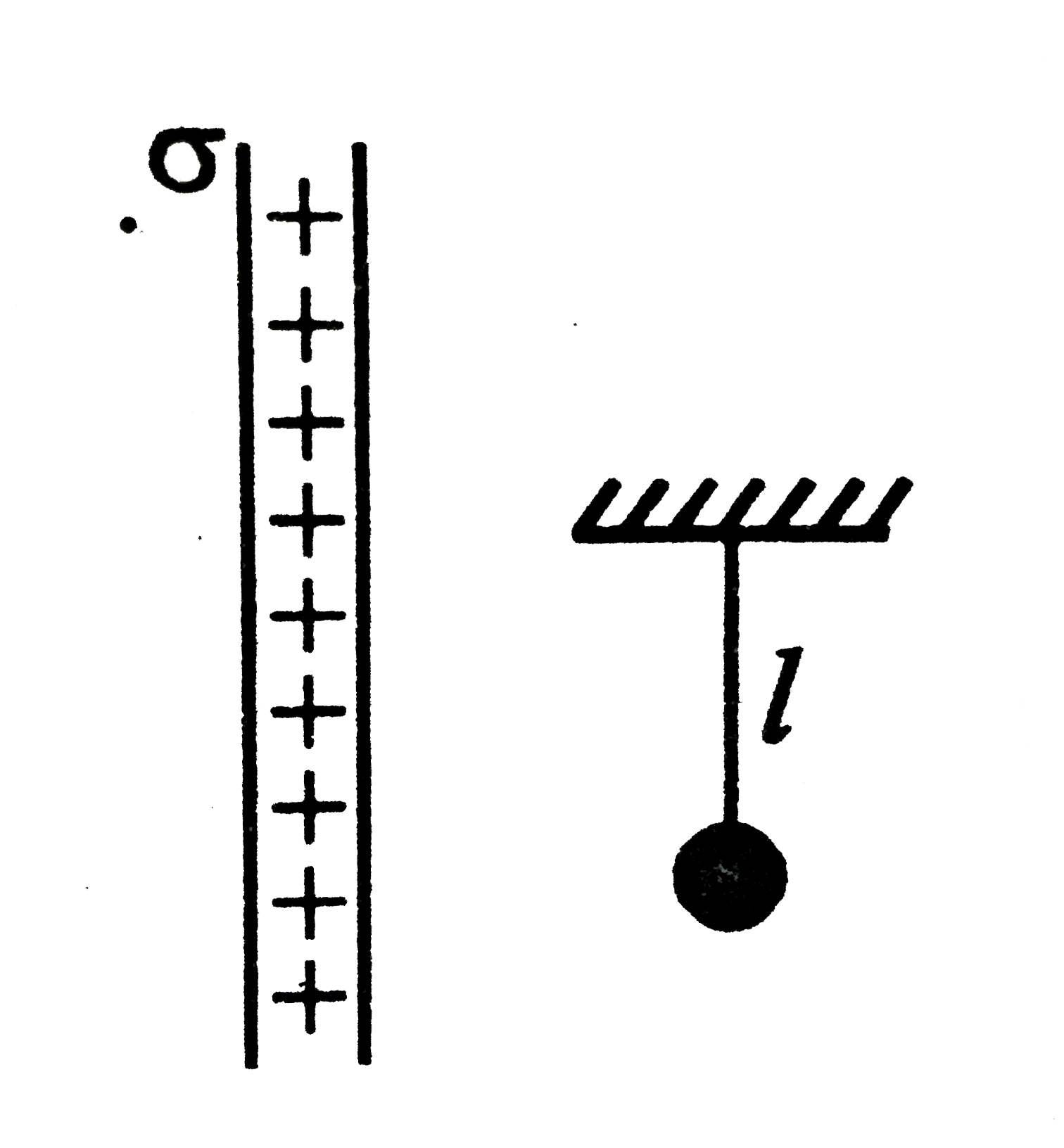
View SolutionA simple pendulum of length l and bob mass m is hanging in front of a large nonconducting sheet having surface charge density σ. If suddenly a charge +q is given to the bob & it is released from the position shown in figure. Find the maximum angle through which the string is deflected from vertical .
 .
.
View SolutionAt absolute zero temperature Si acts as ……..
View SolutionDimension of sqrt((in_(0))/(mu_(0))) are (A) [M L^(2) T^(-3) A^(-2)] (B) [M^(-1) L^(-2) T^(3) A^(2)] (C) [M^(2)L^(2)T^(-3) A^(2)] (D) [M^(-1) L^(2) T^(3) A^(2)]
View SolutionThe physical quanitity the dimensions [M^(-2)L^(-3)T^(0)A^(2)] is
View SolutionAssertion: In y = A sin (omega t - kx),(omega t - kx) is dimensionless. Reason: Because dimension of omega = [M^(0)L^(0)T] .
View SolutionStatement-I : If x and y are the distance along x and y axes respectively then the dimensions of d3ydx3 is M0L−2T∘
Statement-II : Dimensions of ∫baydx is M0L2T∘
View SolutionA.Spring constant1.[M1L2T−2]B.Pascal2.[M0L0T−1]CHertz3.[M1L0T−2]D.Joule4.[M1L−1T−2]
View SolutionIf A = B +(C)/(D+E) the dimensions of B and C are [M^(0)L T^(-1)] and [M^(0)LT^(0)] , respectively . Find the dimensions of A, D and E.
View SolutionDimensions ofε0 are
M−1L−3T4A2
M0L−3T3A3
M−1L−3T3A
M−1L−3TA2
View SolutionPreface: Expressions y = Asin (omegat - kx ) , ( omegat - kx ) Is quarterly. Reason: because the dimension [ M^(0)L^(0)T ] Is
View SolutionIf ∫y0e−t2dt+∫x20sin2tdt=0, then dydx at x=y=1 is
View Solutionकथन: यदि x तथा y क्रमशः x तथा y-अक्षों के अनुदिश दूरियाँ हो, तो d3ydx3 की विमा M0L−2T0 होगी।
कारण: ∫baydx की विमा M0L2T0 है-
View SolutionShow that the diagonals of the parallelogram whose sides are lx+my+n=0,lx+my+n′=0,mx+ly+n=0 and mx+ly+n′=0 include an angle π/2 .
View SolutionDimension of √∈0μ0 are (A) [ML2T−3A−2] (B) [M−1L−2T3A2] (C) [M2L2T−3A2] (D) [M−1L2T3A2]
View SolutionFind the derivative dydx of the following implicit functions :
(a) ∫y0e−t2dt+∫x30sin2tdt=0
(b) ∫y0etdt+∫x0sintdt=0
(c) ∫xπ/2√3−2sin2zdz+∫y0costdt=0
View Solution
