Calculate the value of x in the following equation :-
(i)
(ii)
(iii)
(iv)Solution in Gujrati
The correct Answer is:(i) 2,3,
(ii) 4.5
(iii) 7,8
(iv) 7,9
Step by step video & image solution for Calculate the value of x in the following equation :- (i)x^(2)-5x+6=0 (ii)x^(2)-9x+20=0 (iii) x^(2)-15x+56=0 (iv)x^(2)-16x+63=0 by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (VECTORS)
62 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Units, Dimensions & Measurements)
37 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Graphs))
28 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLEN CHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
State whether the following relations are true or false
(1)−−→AB=−−→BA
(2)−−→AB=−−−→BA
(3)∣∣∣−−→AB∣∣∣=∣∣∣−−→BA∣∣∣
(4)∣∣∣−−→AB∣∣∣=∣∣∣−−−→AB∣∣∣
(5)ˆj=ˆk
(6)∣∣ˆj∣∣=∣∣ˆk∣∣
View SolutionWhich one of the following is not a fundamental quantity ?
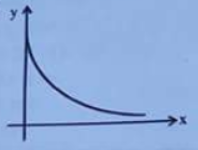
View SolutionWhich of the following equation is best representation of following graph's?
View SolutionAssertion: The driver in a vechicle moving with a constant speed on a straight road is in a non-inertial frame of referance.
Reason: A reference frame in which Newton's laws of motion are applicable is non-inertial.
View SolutionA rod with rectangular cross section oscillates about a horizontal axis passing through one of its ends and it behaves like a seconds pendulum, its length will be
View Solutionx^(2)+15x+56=0
View SolutionA(4,0),B(-4,0) are two points.The locus of P which moves such that |PA-PB|=6 is (i)9x^(2)-7y^(2)+63=0 (ii) 9x^(2)+7y^(2)-63=0( iii) 9x^(2)+7y^(2)+63=0 (iv) 9x^(2)-7y^(2)-63=0
View SolutionCalculate the value of x in the following equation :- (i) x^(2)-5x+6=0 (ii) x^(2)-9x+20=0 (iii) x^(2)-15x+56=0 (iv) x^(2)-16x+63=0
View SolutionI.x2+9x+20=0 II.Y2=16
View Solutionनिम्नलिखित समीकरणों में से प्रत्येक को हल कीजिए:
(i) x2+3=0 (ii) x2+3x+5=0
(iii) x2−x+2=0 (iv) √2x2+x+√2=0
(v) x2+x+1√2=0
View SolutionSolve the following quadratic equations :
(i)9x2−8x+2=0
(ii) √7x2+x+√7=0
(iii)2√3x2−√2x+3√3=0
View SolutionThe equation whose roots are 0 ,0,2,2,−2,−2 is
A) x6+8x4−16x2=0
B)x6−4x4−16x2=0
C) x6−8x4+16x2=0
D) x6+4x4+16x2=0
View SolutionDisuss that nature of roots of
(i) −x2+3x+1=0 (ii) 4x2=0 (iii) 9x2+5x=0
View SolutionFind the value of the polynomial 4x2−5x+3, at
(i) x=0
(ii) x=−1
(iii) x=2
(iv) x=12
View SolutionFind the value of the polynomial 4x2−5x+3, at
(i) x=0
(ii) x=−1
(iii) x=2
(iv) x=12
View SolutionFind the value of the polynomial 4x2−5x+3, at
(i) x=0
(ii) x=−1
(iii) x=2
(iv) x=12
View Solution
