The pressure exerted by an electromagnetic wave of intensity l (watt/m2) on a nonreflecting surface is [c is the velocity of light]
lc
lc2
l/c
l/c2
The correct Answer is:C
To solve the problem of finding the pressure exerted by an electromagnetic wave of intensity I on a non-reflecting surface, we can follow these steps:
Step 1: Understand the Relationship Between Intensity and Energy
The intensity I of an electromagnetic wave is defined as the power per unit area. Mathematically, it can be expressed as:
I=PA
where P is the power and A is the area.
Step 2: Relate Intensity to Momentum
The momentum p of a photon can be expressed in terms of its energy E as:
p=Ec
where c is the speed of light. For an electromagnetic wave, the energy E can be related to intensity I over an area A:
E=I⋅A⋅t
where t is the time duration.
Step 3: Calculate Momentum Per Unit Area
To find the momentum per unit area, we can substitute E into the momentum equation:
Momentum per unit area=EcA=I⋅A⋅tcA=I⋅tc
Since we are interested in the pressure, we will consider the momentum change per unit time.
Step 4: Consider the Non-Reflecting Surface
For a non-reflecting surface, the photons do not reflect back. Therefore, the change in momentum Δp when the wave strikes the surface is equal to the initial momentum:
Δp=I⋅tc
The final momentum is zero since the photons are absorbed.
Step 5: Relate Change in Momentum to Force
The force F exerted on the surface can be defined as the rate of change of momentum:
F=ΔpΔt=Ic
where Δt is the time interval over which the momentum change occurs.
Step 6: Define Pressure
Pressure P is defined as force per unit area:
P=FA
Substituting the expression for force:
P=Ic
Final Result
Thus, the pressure exerted by an electromagnetic wave of intensity I on a non-reflecting surface is given by:
P=Ic
The intensity I of an electromagnetic wave is defined as the power per unit area. Mathematically, it can be expressed as:
I=PA
where P is the power and A is the area.
The momentum p of a photon can be expressed in terms of its energy E as:
p=Ec
where c is the speed of light. For an electromagnetic wave, the energy E can be related to intensity I over an area A:
E=I⋅A⋅t
where t is the time duration.
To find the momentum per unit area, we can substitute E into the momentum equation:
Momentum per unit area=EcA=I⋅A⋅tcA=I⋅tc
Since we are interested in the pressure, we will consider the momentum change per unit time.
For a non-reflecting surface, the photons do not reflect back. Therefore, the change in momentum Δp when the wave strikes the surface is equal to the initial momentum:
Δp=I⋅tc
The final momentum is zero since the photons are absorbed.
The force F exerted on the surface can be defined as the rate of change of momentum:
F=ΔpΔt=Ic
where Δt is the time interval over which the momentum change occurs.
Pressure P is defined as force per unit area:
P=FA
Substituting the expression for force:
P=Ic
Thus, the pressure exerted by an electromagnetic wave of intensity I on a non-reflecting surface is given by:
P=Ic
Similar Questions
Knowledge Check
An electromagnetic wave of intensity I falls on a surface kept in vacuum and exerts radiation pressure p on it.Which of the following are true ?
ARadiation pressure is I/c if the wave is totally absorbed.BRadiation pressure is I/c if the wave is totally reflected.CRadiation pressure is 2 I/c if the wave is totally reflected.DRadiation pressure is in the range I/c<p<2I/c for real surfaces.
What is the force exerted by a photon of intensity 1.4kWm−2 if it falls on a perfect absorber of radius 2 m ?
View SolutionAn electromagnetic wave of intensity I falls on a surface kept in vacuum and exerts radiation pressure p on it.Which of the following are true ?
View SolutionAn electromagnetic wave of intensity I falls on a surface kept in vacuum and exerts radiation pressure p on it.Which of the following are true ?
View SolutionA plane electromagnetic wave of wave intensity 6W/m2 strikes a small mirror of area 40cm(2), held perpendicular to the approaching wave. The momentum transferred by the wave to the mirror each second will be
View SolutionIn the figure shown a converging lens and a diverging lens of focal lengths 20cm and -5cm respectively are separated by a distance such that a paralel beam of light of intensity 100 wa/m2 incident on the converging lens comes out as parallel beam from the diverging lens. Find the intensity of the outgoing beam in wa/m2
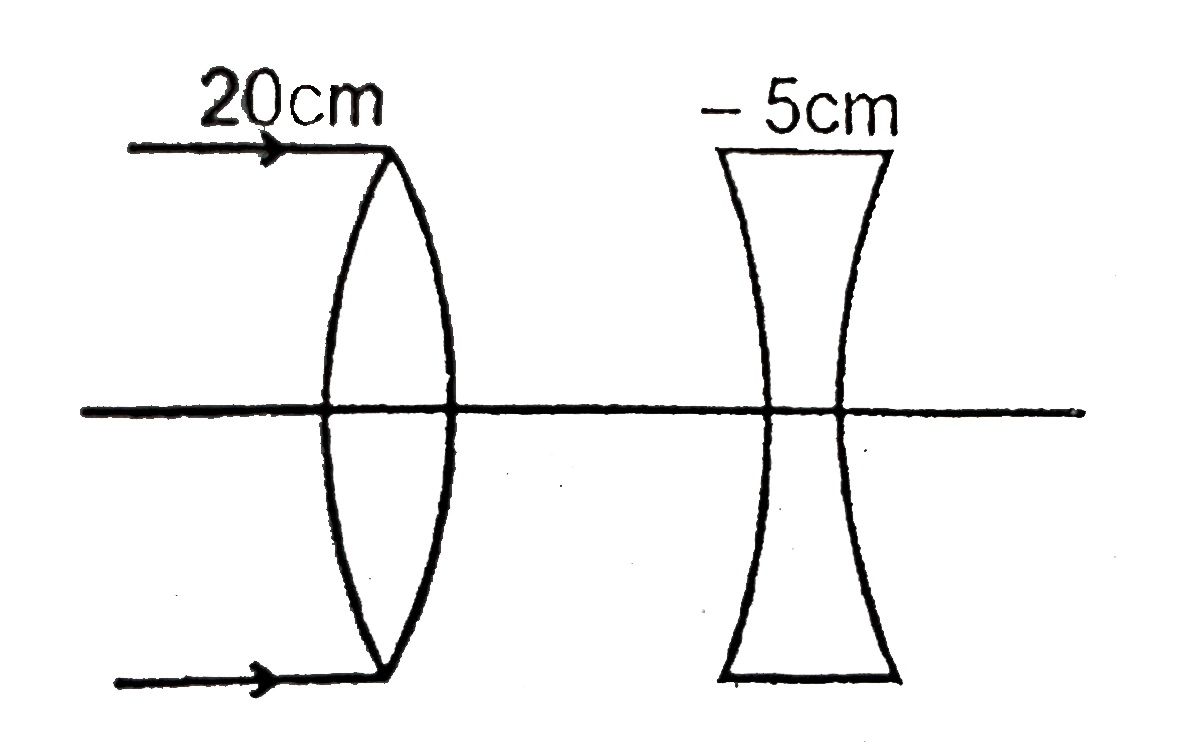
View SolutionThe electric vector vibration of an electromagnetic wave is given by E=(50NC−1)sinω(t−xc), The intensity of the wave is
View SolutionA plane electromagnetic wave of intensity of 10W/m2 strikes a small mmirror of area 20cm2, held perpendicular to the approcaching wave. The radiation froce on the mirror will be:
View Solution
