Electrolyte∧.∞(Scm2mol−1)KCl149.9KNO3145.0HCl426.2NaOAc91.0NaCl126.5
Calculate ∧∞HOAc using appropriate molar conductance of the electrolytes listed above at infinite dilution in H2O at 25∘C
517.2
552.7
390.7
217.5
The correct Answer is:C
To calculate the molar conductance of acetic acid (HOAc) at infinite dilution (Λ∞HOAc), we will use the molar conductance values of the given electrolytes at infinite dilution. The molar conductance of a weak electrolyte like acetic acid can be expressed in terms of its ions.
1. Identify the Molar Conductance of Given Electrolytes:
- KCl: Λ∞KCl=149.9S cm2mol−1
- KNO₃: Λ∞KNO3=145.0S cm2mol−1
- HCl: Λ∞HCl=426.2S cm2mol−1
- NaOAc: Λ∞NaOAc=91.0S cm2mol−1
- NaCl: Λ∞NaCl=126.5S cm2mol−1
2. Write the Molar Conductance Equations:
- For KCl:
Λ∞KCl=Λ∞K++Λ∞Cl−
- For KNO₃:
Λ∞KNO3=Λ∞K++Λ∞NO−3
- For HCl:
Λ∞HCl=Λ∞H++Λ∞Cl−
- For NaOAc:
Λ∞NaOAc=Λ∞Na++Λ∞OAc−
- For NaCl:
Λ∞NaCl=Λ∞Na++Λ∞Cl−
3. Express Λ∞HOAc:
- The molar conductance of acetic acid can be expressed as:
Λ∞HOAc=Λ∞H++Λ∞OAc−
4. Substitute Known Values:
- From the equations, we can isolate Λ∞H+ and Λ∞OAc−:
- From HCl:
Λ∞H+=Λ∞HCl−Λ∞Cl−=426.2−Λ∞Cl−
- From NaCl:
Λ∞Na++Λ∞Cl−=126.5
- From NaOAc:
Λ∞Na++Λ∞OAc−=91.0
5. Solve for Λ∞OAc−:
- Now, we can substitute Λ∞Na+ from the NaCl equation into the NaOAc equation:
(126.5−Λ∞Cl−)+Λ∞OAc−=91.0
- Rearranging gives:
Λ∞OAc−=91.0−(126.5−Λ∞Cl−)
- Simplifying yields:
Λ∞OAc−=Λ∞Cl−−35.5
6. Substituting Back:
- Substitute Λ∞OAc− back into the equation for Λ∞HOAc:
Λ∞HOAc=Λ∞H++Λ∞OAc−
- We can now express Λ∞HOAc in terms of known values.
7. Final Calculation:
- After substituting and simplifying the equations, we find:
Λ∞HOAc=390.7S cm2mol−1
Final Answer:
Λ∞HOAc=390.7S cm2mol−1
- KCl: Λ∞KCl=149.9S cm2mol−1
- KNO₃: Λ∞KNO3=145.0S cm2mol−1
- HCl: Λ∞HCl=426.2S cm2mol−1
- NaOAc: Λ∞NaOAc=91.0S cm2mol−1
- NaCl: Λ∞NaCl=126.5S cm2mol−1
- For KCl:
Λ∞KCl=Λ∞K++Λ∞Cl−
- For KNO₃:
Λ∞KNO3=Λ∞K++Λ∞NO−3
- For HCl:
Λ∞HCl=Λ∞H++Λ∞Cl−
- For NaOAc:
Λ∞NaOAc=Λ∞Na++Λ∞OAc−
- For NaCl:
Λ∞NaCl=Λ∞Na++Λ∞Cl−
- The molar conductance of acetic acid can be expressed as:
Λ∞HOAc=Λ∞H++Λ∞OAc−
- From the equations, we can isolate Λ∞H+ and Λ∞OAc−:
- From HCl:
Λ∞H+=Λ∞HCl−Λ∞Cl−=426.2−Λ∞Cl−
- From NaCl:
Λ∞Na++Λ∞Cl−=126.5
- From NaOAc:
Λ∞Na++Λ∞OAc−=91.0
- Now, we can substitute Λ∞Na+ from the NaCl equation into the NaOAc equation:
(126.5−Λ∞Cl−)+Λ∞OAc−=91.0
- Rearranging gives:
Λ∞OAc−=91.0−(126.5−Λ∞Cl−)
- Simplifying yields:
Λ∞OAc−=Λ∞Cl−−35.5
- Substitute Λ∞OAc− back into the equation for Λ∞HOAc:
Λ∞HOAc=Λ∞H++Λ∞OAc−
- We can now express Λ∞HOAc in terms of known values.
- After substituting and simplifying the equations, we find:
Λ∞HOAc=390.7S cm2mol−1
Λ∞HOAc=390.7S cm2mol−1
Similar Questions

Calculate (A∘HOAC) using appropriate molar conductances of the electrolytes listed above at infinite dilution in H2O at 25∘C .
View Solution
Calculate, molar conductance of acetic acid using appropriate molar conductances of the electrolytes listed above at infinite dilution in H2O at 25∘C :
View SolutionFor strong electrolytes the values of molar conductivities at infinite dilution are given below
(Electrolyte,∧∘m(Sm2mol−1),(BaCl2,280×10−4),(NaCl,126.5×10−4),<br><br>(NaOH,48×10−4)
The molar conductance at infinite dilution for Ba(OH)2 is
View Solution1.0 molal aqueous solution of an electrolyte X3Y2 is 25% ionized. The boiling point of the solution is (Kb for H2O=0.52Kkg/mol):
View Solution0.1 molal aqueous solution of an electrolyte AB3 is 90% ionised. The boiling point of the solution at 1 atm is (Kb(H2O)=0.52kg mol−1)
View Solution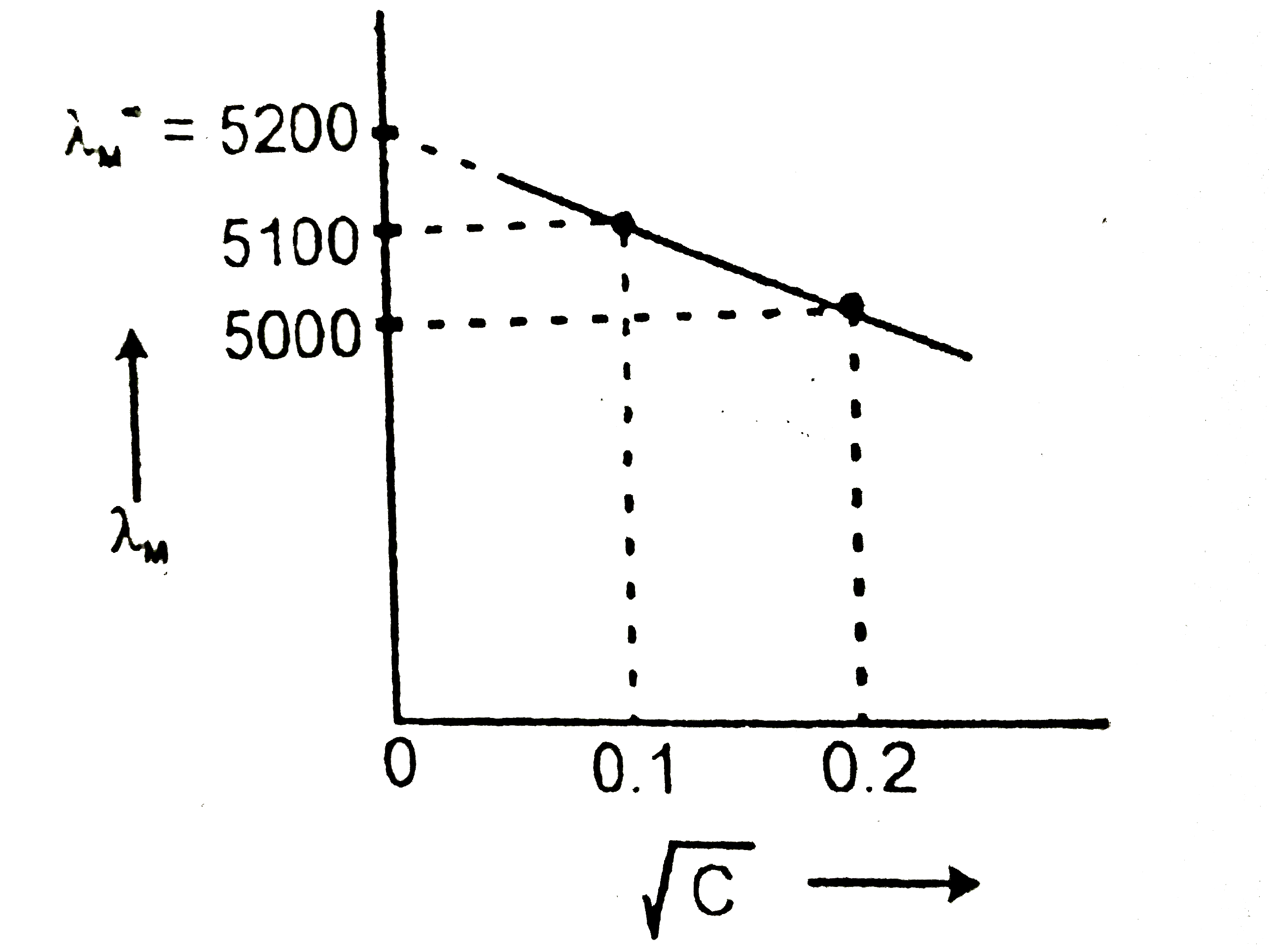
At 0.04 M concentration the molar conductivity of a solution of a electrolyte is 5000Ω−1cm2mol−1 while at 0.01 M concentration the value is 5100Ω−1cm2mol−1 making necessary assumption (taking it as strong electrolyte) find the molar conductivity at infinite dilution and also determine the degree of dissociation of strong electrolyte at 0.04M.
View SolutionAt 0.04 M concentration, the molar conductivity of solution of an electrolyte is 5000Ω−1cm2mol−1 while at 0.01 M concentration the value is 5100Ω−1cm2mol−1. Making necessary assumption (Taking it as strong electrolyte) find the molar conductivity at infinite dilution and write percentage dissociation of strong electrolyte at 0.04 M.
View SolutionThe conductance (G) is
View SolutionThe conductivity of 0.001 M acetic is 4×10−5S/cm. Calculate the dissociation constant of acetic acid, if molar conductivity at infinite dilution for acetic is 390 S cm2/mol.
View SolutionThe molar ionic conductance at infinite dilution of K+ and SO2−4
are 73.5 and 160 Scm2mol−1 respectively.The molar
conductance of solution of K2SO4 at infinite dilution will be
View Solution
