On the same path, the source and observer are moving such a way that the distance between these two increases with the time. The speeds of source and observer are same and equal to 10ms−1 with respect to the ground while no wind in blowing. The apparent frequency received by observer is 1950Hz then the original frequency must be (the speed of sound in present medium is 340m/s
2068 Hz
2100 Hz
1903 Hz
602 Hz
The correct Answer is:A
To solve the problem, we need to determine the original frequency of the source when the observer is moving away from it. We will use the Doppler effect formula for sound when both the source and the observer are moving away from each other.
1. Identify the Given Values:
- Speed of the source (Vs) = 10 m/s
- Speed of the observer (Vo) = 10 m/s
- Apparent frequency (f') = 1950 Hz
- Speed of sound in air (v) = 340 m/s
2. Understand the Doppler Effect Formula:
When both the source and observer are moving away from each other, the apparent frequency (f') can be calculated using the formula:
f′=v−Vov+Vsf
where:
- f′ = apparent frequency
- f = original frequency
- Vo = speed of the observer
- Vs = speed of the source
- v = speed of sound
3. Substitute the Known Values:
Since both the source and observer are moving away, we substitute the values into the formula:
1950=340−10340+10f
4. Simplify the Equation:
Calculate the numerator and denominator:
1950=330350f
5. Cross-Multiply to Solve for f:
1950⋅350=330f
682500=330f
6. Isolate f:
f=682500330
7. Calculate the Original Frequency:
f≈2068.18 Hz
Rounding to the nearest whole number, we find:
f≈2068 Hz
Final Answer:
The original frequency of the source is approximately 2068 Hz.
- Speed of the source (Vs) = 10 m/s
- Speed of the observer (Vo) = 10 m/s
- Apparent frequency (f') = 1950 Hz
- Speed of sound in air (v) = 340 m/s
When both the source and observer are moving away from each other, the apparent frequency (f') can be calculated using the formula:
f′=v−Vov+Vsf
where:
- f′ = apparent frequency
- f = original frequency
- Vo = speed of the observer
- Vs = speed of the source
- v = speed of sound
Since both the source and observer are moving away, we substitute the values into the formula:
1950=340−10340+10f
Calculate the numerator and denominator:
1950=330350f
1950⋅350=330f
682500=330f
f=682500330
f≈2068.18 Hz
Rounding to the nearest whole number, we find:
f≈2068 Hz
The original frequency of the source is approximately 2068 Hz.
Similar Questions
A source and a detector move away from each other, each with a speed of 10ms−1 with respect to the ground with no wind. If the detector detects a frequency 1950 Hz of the sound coming from the source, what is the original frequency of the source? Speed of sound in air =340ms−1.
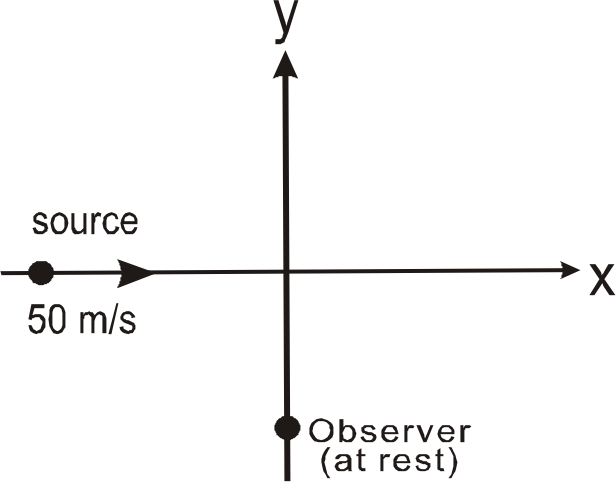
View SolutionA sound source moving with speed 50 m/s along x-axis and observer at rest on y-axis.If the frequency observed by observer when source crosses the origin is 96 Hz, then the original frequency of source is :(speed of sound is given medium is 200 m/s)
View Solution
Knowledge Check
At what speed should a source of sound move so that observer finds the apparent frequency equal to half of the original frequency ?
Av2B2vCv/4Dv
A source and a detector move away from each other, each with a speed of 10 m/s with respect to ground with no wind. If the detector detects a frequency 1650 Hz of the sound coming from the source, what is the original frequency of the source? (speed of sound = 340 m/s
View SolutionA source of sound and an observer are approaching each other with the same speed, which is equal to (110) times the speed of sound. The apparent relative change in frequency of source is
View SolutionShow that when the speed of the source and the observer are small compared to the speed of sound in the medium, the change in frequency becomes independent of the fact whether the source is moving or the observer.
View Solutionif the speed of a sound source is equal to the speed fo sound and the source is moving away from the observer, then the ratio of apparent frequency to the original frequency is
View SolutionSource and observer start moving simulatneously along x and y-axis respectively. The speed of source is twice the speed of observer V0. If the ratio of observer frequency to the frequency of the source is 0.75, find the velocity of sound.
View SolutionSource and observer start moving simulatneously along x and y-axis respectively. The speed of source is twice the speed of observer V0. If the ratio of observer frequency to the frequency of the source is 0.75, find the velocity of sound.
View SolutionA sound source has frequency f. Source and observer both have same speed.For the apparent frequency observed by observer match the following.

View Solution
