Two circular loops having same radius [ R = 10 cm] and same current 72A are placed along same axis as shown. If distance between their centre is 10 cm. find net magnetic field at of point P.
![Two circular loops having same radius [ R = 10 cm] and same current (7)/(2)A are placed along same axis as shown. If distance between their centre is 10 cm. find net magnetic field at of point P.](https://d10lpgp6xz60nq.cloudfront.net/physics_images/RES_AIIMS_ENT_26_MAY_19_S1_E01_015_Q01.png)
50μ0√5T
28μ0√5T
56μ0√5T
56μ0√3T
The correct Answer is:C
At point P, magnetic field will be in same direction by both coils.
B=B1+B2 and B1=B2
B=μ0ir22(r2+n2)3/2×2
B=μ0ir2(r2+n2)3/2
B=μ0×72×(.1)2[(0.1)2+(0.05)2]3/2=56μ0√5T
Similar Questions
A circular loop of radius 0.3 cm lies parallel to amuch bigger circular loop of radius 20 cm. The centre of the small loop is on the axis of the bigger loop. The distance between their centres is 15 cm. If a current of 2.0 A flows through the smaller loop, then the flux linked with bigger loop is
View SolutionA circular loop of radius 0.3 cm lies parallel to amuch bigger circular loop of radius 20 cm. The centre of the small loop is on the axis of the bigger loop. The distance between their centres is 15 cm. If a current of 2.0 A flows through the smaller loop, then the flux linked with bigger loop is
View Solution
Knowledge Check
A circular loop of radius 3 cm is having a current of 12.5 A. The magnitude of magnetic field at a distance of 4 cm on its axis is
A5.65×10−5TB5.27×10−5TC6.54×10−5TD9.20×10−5T
A circular loop of radius 4.0 cm is placed in a horizontal plane and carries an electric current of 5.0 A in the clockwise direction as seen from above. Find the magnetic field (a) at a point 3.0 cm above the centre of the loop (b) at a point 3.0 cm below the centre of the loop.
View SolutionTwo parallel chords of a circle , 12 cm and 16 cm long are on the same of the centre. The distance between them is 2 cm. Find the radius of the circle.
View SolutionA circular loop of radius a, carrying a current I, is placed in a tow dimensional magnetic field. The centre of the loop coincides with the centre of the filed The strenght of the magnetic field at the pariphery of the loop is B. find the magnetic force on the wire.

View SolutionTwo short magnets of magnetic moment 1000Am2 are placed as shown at the corners of a square of side 10cm. The net magnetic induction at P is
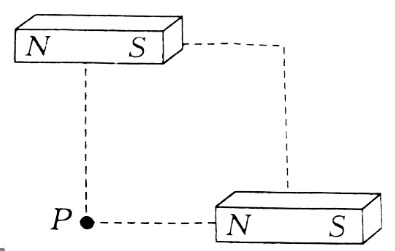
View SolutionA circular coil of 100 turns has a radius of 10 cm and carries a current of 5 A. Determine the magnetic field at the centre of the coil and at a point on the axis of the coil at a distance of 5 cm from its centre.
View SolutionA long solenoid having n = 200 turns per metre has a circular cross-section of radius a1=1cm. A circular conducting loop of radius a2=4cm and resistance R=5(Ω) encircles the solenoid such that the centre of circular loop coincides with the midpoint of the axial line of the solenoid and they have the same axis as shown in Fig.
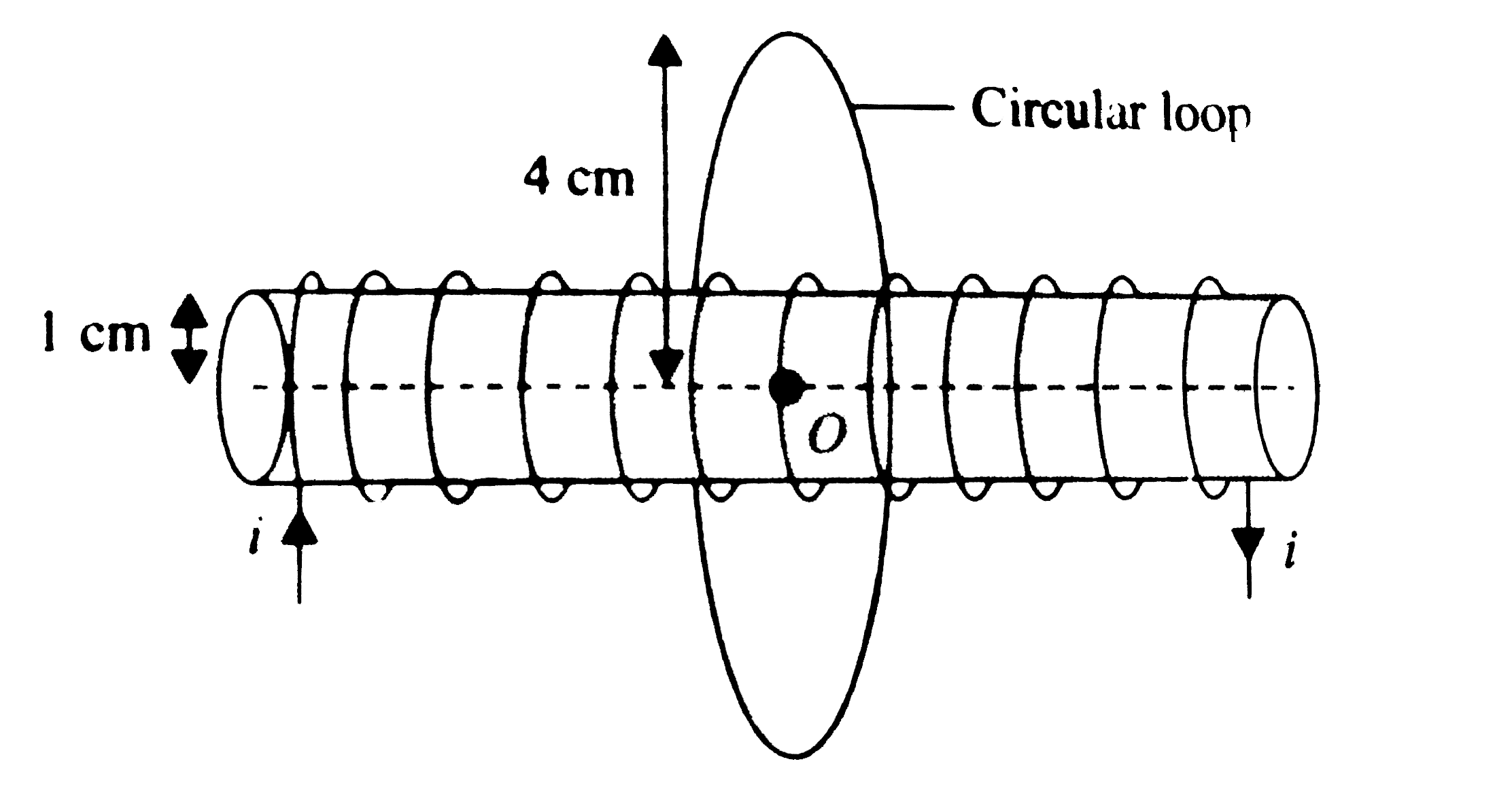
A current 't' in the solenoid results in magnetic field along its axis with magnitude B=(μ)ni at points well inside the solenoid on its axis. We can neglect the insignificant field outside the solenoid. This results in a magnetic flux (ϕ)B through the circular loop. If the current in the winding of solenoid is changed, it will also change the magnetic field B=(μ)0ni and hence also the magnetic flux through the circular loop. Obvisouly, it will result in an induced emf or induced electric field in the circular loop and an induced current will appear in the loop. Let current in the winding of solenoid be reduced at a rate of 75A/sec.
Magentic flux through the loop due to external magnetic field will be
I is the current in the loop
a1 is the radius of solendoid and a1=1cm (given)
a2 is the radius of circular loop and a2=4cm (given)
i is hte current in the solenoid
View SolutionA long solenoid having n = 200 turns per metre has a circular cross-section of radius a1=1cm. A circular conducting loop of radius a2=4cm and resistance R=5(Ω) encircles the solenoid such that the centre of circular loop coincides with the midpoint of the axial line of the solenoid and they have the same axis as shown in Fig.

A current 't' in the solenoid results in magnetic field along its axis with magnitude B=(μ)ni at points well inside the solenoid on its axis. We can neglect the insignificant field outside the solenoid. This results in a magnetic flux (ϕ)B through the circular loop. If the current in the winding of solenoid is changed, it will also change the magnetic field B=(μ)0ni and hence also the magnetic flux through the circular loop. Obvisouly, it will result in an induced emf or induced electric field in the circular loop and an induced current will appear in the loop. Let current in the winding of solenoid be reduced at a rate of 75A/sec.
When the current in the solenoid becomes zero so that external magnetic field for the loop stops changing, current in the loop will follow a differenctial equation given by [You may use an approximation that field at all points in the area of loop is the same as at the centre
View Solution
