What is the gravitaional force acting on 2 kg mass ? [Take g = 10ms^(-2)]
The correct Answer is:20 N
Gravitational force , mg =2×10=20N
Topper's Solved these Questions
- AMAZING AIRBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - AMAZING AIREXERCISE - Very short Answer Type Questions
20 Videos
- AMAZING AIRBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - AMAZING AIREXERCISE - Short Answer Type Questions
29 Videos
- ENVIRONMENT- AN ETERNAL GIFT TO MANKINDBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - ENVIRONMENT- AN ETERNAL GIFT TO MANKINDEXERCISE - Crossword
1 Videos
Similar Questions
Knowledge Check
A force of 100N is applied on a block of mass 3 kg as shown in the figure-2.164. The coefficient of friction between the surface and the block is 0.25.The frictional force acting on the block is: (Take g=10ms2)
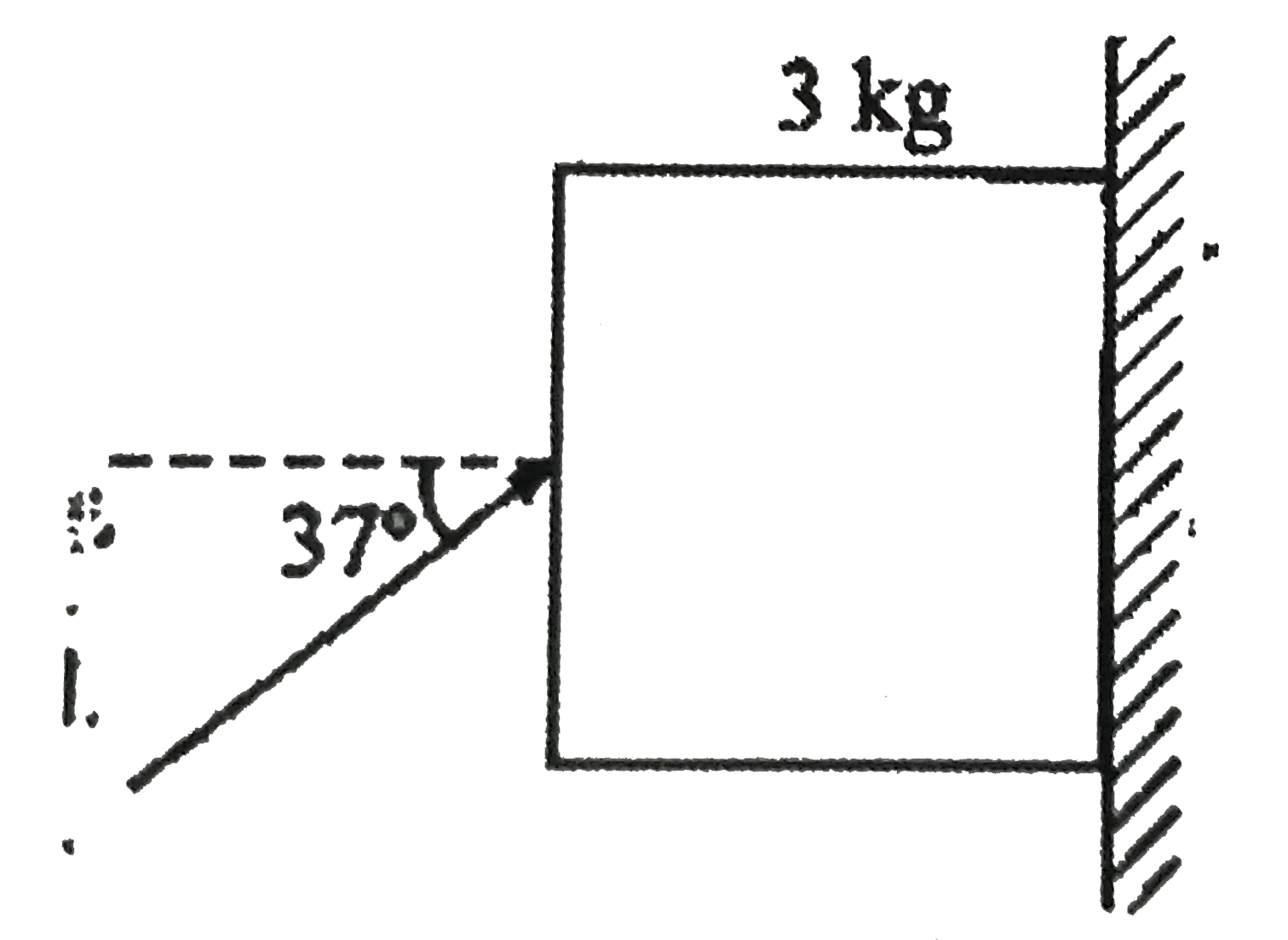 A15 N downwardsB25 N upwardsC20 N downwardsD30 N upwards
A15 N downwardsB25 N upwardsC20 N downwardsD30 N upwardsA body of mass 2 kg is placed on a horizontal surface having coefficient of kinetic friction 0.4 and limiting coefficient of static friction 0.5. If a horizontal force 2.5 N is applied on the body, the frictional force acting on the body will be (Take, g=10ms2)
A8 NB10 NC20 ND2.5 NA block of mass 20 kg is pushed with a horizontal force of 90N. If the coefficient of static and kinetic friction are 0.4 and 0.3, the frictional force acting on the block is (g=10ms−2)
A90NB80NC60ND30N
A body of mass 25 kg, is placed on a horizontal surface whose coefficient of friction 0.25. Find the frictional force action on it. Take (g=10ms−2)
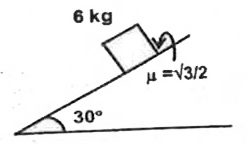
View SolutionA block of mass 6 kg is kept on rough surface as shown in figure. Find acceleration and friction force acting on the block. (Take g=10m/s2)
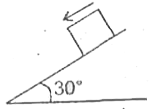
View SolutionA block of mass 10 kg is released on rough incline plane. Block start descending with acceleration 2m/s2. Kinetic friction force acting on block is (take g=10m/s2)
View SolutionA block of mass 20kg is pushed with a horizontal force of 90N. It the coefficient of static and kinetic friction are 0.4 and 0.3, the frictional force acting on the block is (g=10ms−2) .
View SolutionA simple pendulum having a bob of mass 100 g is oscillating with a maximum angular displacement of 3∘. The maximum restoring force acting on the bob is (take g=10ms−2)
View Solution
