State Dalton's law of partial pressures . Derive the same from the kinetic gas equation .
Step-by-Step Solution:
Step 1: State Dalton's Law of Partial Pressures
Dalton's Law of Partial Pressures states that in a mixture of non-reacting gases, the total pressure exerted by the mixture is equal to the sum of the partial pressures of each individual gas. Mathematically, this can be expressed as:
Ptotal=PA+PB+PC+…
where PA,PB,PC,… are the partial pressures of the individual gases in the mixture.
Step 2: Understand the Kinetic Gas Equation
The kinetic gas equation relates the pressure (P), volume (V), number of moles (n), and temperature (T) of an ideal gas. It can be expressed as:
P=13mnc2V
where:
- m is the mass of the gas,
- n is the number of molecules,
- c is the average speed of the gas molecules,
- V is the volume of the gas.
Step 3: Apply the Kinetic Gas Equation to a Mixture of Gases
For a mixture of gases, we can consider two gases, A and B, with their respective pressures PA and PB. According to the kinetic gas equation, we can write:
PA=13mAnAc2AV
PB=13mBnBc2BV
Step 4: Derive the Total Pressure
The total pressure Ptotal of the gas mixture can be expressed as:
Ptotal=PA+PB
Substituting the expressions for PA and PB:
Ptotal=13mAnAc2AV+13mBnBc2BV
Step 5: Generalize for More Gases
This can be generalized for any number of gases in the mixture:
Ptotal=∑iPi
where Pi is the partial pressure of each gas in the mixture.
Step 6: Conclusion
Thus, from the kinetic gas equation, we can derive that the total pressure of a mixture of non-reacting gases is indeed the sum of the partial pressures of each gas, which is consistent with Dalton's Law of Partial Pressures.
---
Dalton's Law of Partial Pressures states that in a mixture of non-reacting gases, the total pressure exerted by the mixture is equal to the sum of the partial pressures of each individual gas. Mathematically, this can be expressed as:
Ptotal=PA+PB+PC+…
where PA,PB,PC,… are the partial pressures of the individual gases in the mixture.
The kinetic gas equation relates the pressure (P), volume (V), number of moles (n), and temperature (T) of an ideal gas. It can be expressed as:
P=13mnc2V
where:
- m is the mass of the gas,
- n is the number of molecules,
- c is the average speed of the gas molecules,
- V is the volume of the gas.
For a mixture of gases, we can consider two gases, A and B, with their respective pressures PA and PB. According to the kinetic gas equation, we can write:
PA=13mAnAc2AV
PB=13mBnBc2BV
The total pressure Ptotal of the gas mixture can be expressed as:
Ptotal=PA+PB
Substituting the expressions for PA and PB:
Ptotal=13mAnAc2AV+13mBnBc2BV
This can be generalized for any number of gases in the mixture:
Ptotal=∑iPi
where Pi is the partial pressure of each gas in the mixture.
Thus, from the kinetic gas equation, we can derive that the total pressure of a mixture of non-reacting gases is indeed the sum of the partial pressures of each gas, which is consistent with Dalton's Law of Partial Pressures.
Similar Questions
What is kinetic gas equation ?
View SolutionDalton's law of partial pressures is not applicable to
View SolutionDalton's law of partial pressure are applicable to
View SolutionOut of three states of matter, only the gases have most of the physical properties common. They have neither definite shapes nor definite volume. In addition to this, the gases obey different gas laws such as Boyles's law. Charles law, Dalton's law of partial pressures etc. Based upon these gas laws, ideal gas equation PV=nRT has been derived.
Answer the following questions on the basis of above paragraph.
(i) what is Dalton's law of partial pressures ?
(ii) Derive ideal gas equation on the basis of all gas laws.
View SolutionWhat is the use of Dalton's law of partial pressure ?
View SolutionDerive the perfect gas equation.
View SolutionState and explain Daltan's law of partial pressures. How is the law helpful in calculating the pressures of the gases which are collected over water ?
View SolutionBased on the given diagram, which of the following statements regarding the homogenous solution of two volatile liquids are correct? (1) Plots AD and BC show that Raoult's law is obeyed for the solution in which B is a solvent and A is the solute and as well as for that in which A is solvent and B is solute. (2) Plot CD shows that Dalton's law of partial pressures is obeyed by the binary solution of components A and B. (3) EF + EG = EH; and AC and BD correspond to the vapour pressure of the pure solvents A and B respectively.
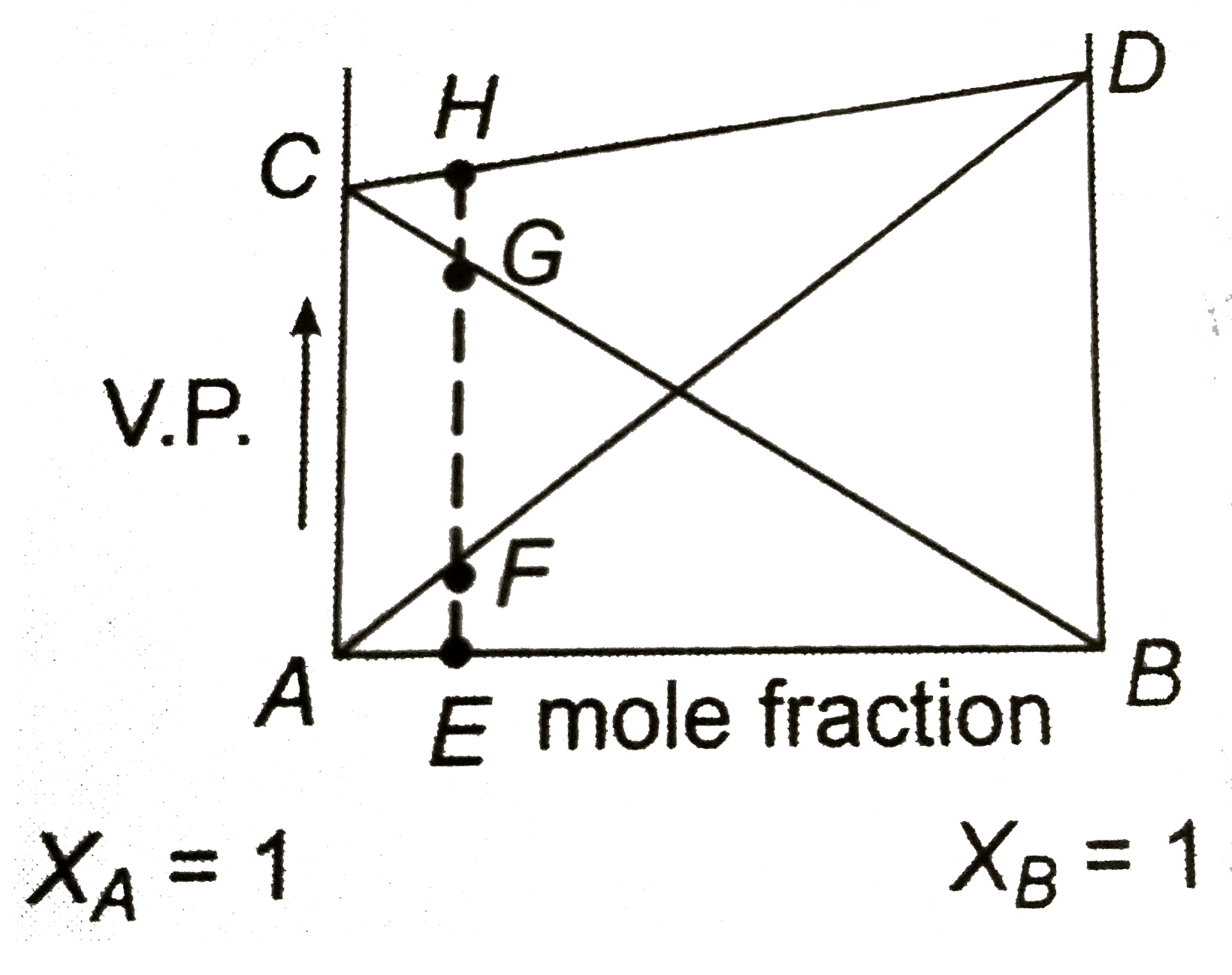
View SolutionState the following : Gas equation
View SolutionThe partial pressure of a dry gas is
View Solution
