The correct statement (s) for cubic close packed (ccp) three dimensional structure is (are)
The number of the neighbours of an atom present in the topmost layer is 12
The efficiency of atom packing is
The number of octahedral and tetrahedral voids per atom are 1 and 2 respectively
The unit cell edge length is times the radius of the atom
The correct Answer is:B, C, D
To solve the question regarding the correct statements for the cubic close packed (CCP) three-dimensional structure, we will analyze each statement provided in the question.
1. Statement 1: The number of neighbors of the atom present in the topmost layer is 12.
- Analysis: This statement is incorrect. The coordination number of an atom in the topmost layer of a cubic close-packed structure (also known as face-centered cubic, FCC) is not 12 because there are no atoms above it. The coordination number refers to the number of nearest neighbors surrounding an atom. In the case of an atom in the topmost layer, it is surrounded by 4 atoms in the same layer and 4 atoms in the layer below, giving a total of 8 neighbors.
- Conclusion: This statement is false.
2. Statement 2: The efficiency of atom packing is 74%.
- Analysis: This statement is correct. The packing efficiency of a cubic close-packed structure is indeed 74%. This can be calculated using the formula for packing fraction:
Packing Fraction=Z×Volume of sphereVolume of cube
For FCC, Z=4 (the number of atoms per unit cell), and the packing fraction comes out to be 74%.
- Conclusion: This statement is true.
3. Statement 3: The number of octahedral and tetrahedral voids per atom is 1 and 2.
- Analysis: This statement is also correct. In a cubic close-packed structure, there is 1 octahedral void for each atom and 2 tetrahedral voids for each atom. This is derived from the fact that the number of octahedral voids equals the number of atoms per unit cell (Z), and the number of tetrahedral voids is twice the number of atoms per unit cell.
- Conclusion: This statement is true.
4. Statement 4: The unit cell edge length is 2√2 times the radius of the atom.
- Analysis: This statement is correct. In an FCC lattice, the relationship between the edge length (a) and the atomic radius (R) can be derived from the geometry of the face diagonal. The face diagonal is equal to a√2 and is also equal to 4R (since it contains 3 atomic radii). Therefore, we can derive:
a√2=4R⟹a=4R√2=2√2R
- Conclusion: This statement is true.
Final Conclusion:
The correct statements regarding the cubic close packed (CCP) structure are:
- Statement 2: True
- Statement 3: True
- Statement 4: True
Summary of Correct Statements:
- Statement 2, Statement 3, and Statement 4 are correct.
- Analysis: This statement is incorrect. The coordination number of an atom in the topmost layer of a cubic close-packed structure (also known as face-centered cubic, FCC) is not 12 because there are no atoms above it. The coordination number refers to the number of nearest neighbors surrounding an atom. In the case of an atom in the topmost layer, it is surrounded by 4 atoms in the same layer and 4 atoms in the layer below, giving a total of 8 neighbors.
- Conclusion: This statement is false.
- Analysis: This statement is correct. The packing efficiency of a cubic close-packed structure is indeed 74%. This can be calculated using the formula for packing fraction:
Packing Fraction=Z×Volume of sphereVolume of cube
For FCC, Z=4 (the number of atoms per unit cell), and the packing fraction comes out to be 74%.
- Conclusion: This statement is true.
- Analysis: This statement is also correct. In a cubic close-packed structure, there is 1 octahedral void for each atom and 2 tetrahedral voids for each atom. This is derived from the fact that the number of octahedral voids equals the number of atoms per unit cell (Z), and the number of tetrahedral voids is twice the number of atoms per unit cell.
- Conclusion: This statement is true.
- Analysis: This statement is correct. In an FCC lattice, the relationship between the edge length (a) and the atomic radius (R) can be derived from the geometry of the face diagonal. The face diagonal is equal to a√2 and is also equal to 4R (since it contains 3 atomic radii). Therefore, we can derive:
a√2=4R⟹a=4R√2=2√2R
- Conclusion: This statement is true.
The correct statements regarding the cubic close packed (CCP) structure are:
- Statement 2: True
- Statement 3: True
- Statement 4: True
- Statement 2, Statement 3, and Statement 4 are correct.
Similar Questions
Knowledge Check
In the cubic close packing, the unit cell has
A4 tetrahedral voids each of which is shared by four adjacent unit cellsB4 tetrahedral voids within the unit cellC8 tetrahedral voids cach of which is shared by four adjacent unit cellsD8 tetrahedral voids within the unit cells
Select the correct statement(s) related to hexagonal close packing of identical spheres in three dimensions :
View SolutionPercentage of free space in cubic close packed struchure and in body centred structure are respectively.
View SolutionPacking refers to the arrangement of constituent units in such a way that the forces of attraction among the constituent particles is the maximum and the contituents occupy the maximum available space. In two dimensions, there are hexagonal close packing and cubic close packing. In three dimentions, there are hexagonal, cubic as well as body centred close packings.
The empty space left in ccp packing is:
View SolutionSelect the correct statement(s) about three-dimensional hcp system.
View SolutionPacking refers to the arrangement of constituent units in such a way that the forces of attraction among the constituent particles is the maximum and the contituents occupy the maximum available space. In two dimensions, there are hexagonal close packing and cubic close packing. In three dimentions, there are hexagonal, cubic as well as body centred close packings.
A certain oxide of a metal M crystallises in such a way that O2− ions occupy hcp arrangement following ABAB... pattern. The metal ions however occupy 2/3 rd of the octahedral voids. The formula of the compound is :
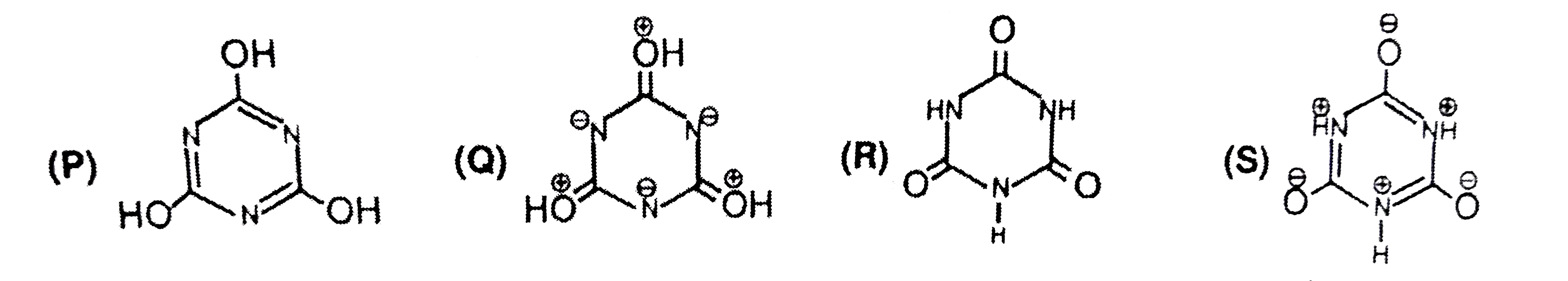
View SolutionThe correct statement(s) concerning the structure P,Q,R & S is/are
View SolutionVolume occupied for a cubic close packed lattice of sphere is
View Solution
