The transition of electron in H atom that will emit maximum energy is
n3→n2
n4→n3
n5→n4
n6→n5
The correct Answer is:A
To determine the transition of an electron in a hydrogen atom that will emit maximum energy, we can follow these steps:
Step 1: Understand Energy Levels in Hydrogen Atom
In a hydrogen atom, electrons occupy specific energy levels, denoted by the principal quantum number n. The energy levels are quantized and can be represented as:
- n=1 (ground state)
- n=2
- n=3
- n=4
- n=5
- n=6
Step 2: Identify the Formula for Energy Difference
The energy associated with each level can be calculated using the formula:
En=−13.6eVn2
To find the energy emitted during a transition from a higher energy level ni to a lower energy level nf, we use:
ΔE=Ef−Ei=−13.6n2f+13.6n2i
This can be simplified to:
ΔE=13.6⎛⎝1n2f−1n2i⎞⎠
Step 3: Calculate Energy Differences for Given Transitions
We need to calculate the energy differences for the possible transitions:
1. Transition from n=3 to n=2:
ΔE3→2=13.6(122−132)=13.6(14−19)=13.6(9−436)=13.6(536)≈1.89eV
2. Transition from n=4 to n=3:
ΔE4→3=13.6(132−142)=13.6(19−116)=13.6(16−9144)=13.6(7144)≈0.66eV
3. Transition from n=5 to n=4:
ΔE5→4=13.6(142−152)=13.6(116−125)=13.6(25−16400)=13.6(9400)≈0.31eV
4. Transition from n=6 to n=5:
ΔE6→5=13.6(152−162)=13.6(125−136)=13.6(36−25900)=13.6(11900)≈0.17eV
Step 4: Compare Energy Differences
Now, we compare the energy differences calculated:
- ΔE3→2≈1.89eV
- ΔE4→3≈0.66eV
- ΔE5→4≈0.31eV
- ΔE6→5≈0.17eV
Conclusion
The transition that emits the maximum energy is from n=3 to n=2.
Final Answer
The transition of electron in H atom that will emit maximum energy is from n=3 to n=2.
---
In a hydrogen atom, electrons occupy specific energy levels, denoted by the principal quantum number n. The energy levels are quantized and can be represented as:
- n=1 (ground state)
- n=2
- n=3
- n=4
- n=5
- n=6
The energy associated with each level can be calculated using the formula:
En=−13.6eVn2
To find the energy emitted during a transition from a higher energy level ni to a lower energy level nf, we use:
ΔE=Ef−Ei=−13.6n2f+13.6n2i
This can be simplified to:
ΔE=13.6⎛⎝1n2f−1n2i⎞⎠
We need to calculate the energy differences for the possible transitions:
1. Transition from n=3 to n=2:
ΔE3→2=13.6(122−132)=13.6(14−19)=13.6(9−436)=13.6(536)≈1.89eV
ΔE4→3=13.6(132−142)=13.6(19−116)=13.6(16−9144)=13.6(7144)≈0.66eV
ΔE5→4=13.6(142−152)=13.6(116−125)=13.6(25−16400)=13.6(9400)≈0.31eV
ΔE6→5=13.6(152−162)=13.6(125−136)=13.6(36−25900)=13.6(11900)≈0.17eV
Now, we compare the energy differences calculated:
- ΔE3→2≈1.89eV
- ΔE4→3≈0.66eV
- ΔE5→4≈0.31eV
- ΔE6→5≈0.17eV
The transition that emits the maximum energy is from n=3 to n=2.
The transition of electron in H atom that will emit maximum energy is from n=3 to n=2.
Similar Questions
Knowledge Check
The ground state energy of an atom is −13.6eV. The photon emitted during the transition of electron from n=3 to n=1 state, is incidenet on a photosensitive material of unknown work function. The photoelectrons are emitted from the materials with a maximum kinetic energy of 9 eV. the threshold wavelength of the material used is
A0.9×10−7mB4×10−7mC0.47×10−7mD9×10−7m
Which of the following electronic transition in hydrogen atom will emit largest amount of energy?
View SolutionWhich of the following transition will emit maximum energy in hydrogen atom ?
View SolutionWhat will be the kinetic energy and total energy of an electron in H atom if the atom emit a photon of wavelength 4860Å ?
View SolutionIn Bohr Atomic model regarding transition of electron for hydrogen like atom following observation were noted.
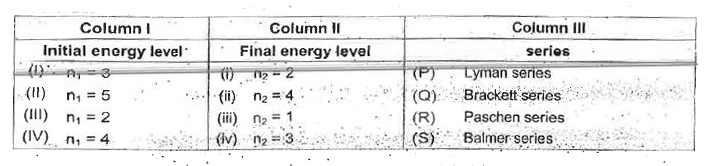
Transition of electron to corresponding to lowest energy released is
View SolutionCalculate the ratio of the frequencies of the radiation emitted due to transition of the electron in a hydrogen atom from its (i) second permitted energy level to the first level and (ii) highest permitted energy level to the second permitted level .
View SolutionConsidering no electronic repulsion in Helium atom what will be Bohr's energy for both the electrons ? Bohr's energy of ground state of H− atom is −13.6eV
View SolutionIf the wavelength of photon emitted due to transition of electron from third orbit to first orbit in a hydrogen atom is λ then the wavelength of photon emitted due to transition of electron from fourth orbit to second orbit will be
View Solution