Which electronic transition in a hydrogen atom releases the greatest amount of energy?
n = 1 to n = 2
n = 2 to n = 4
n = 3 to n = 6
n=∞ to n = 1
The correct Answer is:A
To determine which electronic transition in a hydrogen atom releases the greatest amount of energy, we can follow these steps:
Step 1: Understand the Energy Levels
In a hydrogen atom, the energy levels are quantized and can be described by the formula:
En=−13.6eVn2
where En is the energy of the level, and n is the principal quantum number (n = 1, 2, 3,...).
Step 2: Energy and Quantum Number Relationship
From the formula, we can see that energy is inversely proportional to the square of the principal quantum number n. This means:
- As n increases, the energy becomes less negative (increases).
- The lower the value of n, the more negative the energy, indicating a higher energy state when transitioning to a lower level.
Step 3: Identify the Transition with Maximum Energy Release
To find the transition that releases the greatest amount of energy, we need to look for the transition from a higher energy level (higher n) to a lower energy level (lower n). The transition that releases the most energy occurs when an electron falls from the highest energy level (infinity) to the lowest energy level (n = 1).
Step 4: Calculate the Energy for the Transition
The transition from n=∞ to n=1 can be calculated as follows:
- Energy at n=∞:
E∞=0eV
- Energy at n=1:
E1=−13.6eV
The energy released during the transition from n=∞ to n=1 is:
ΔE=E1−E∞=−13.6eV−0eV=−13.6eV
This indicates that 13.6 eV of energy is released.
Conclusion
The electronic transition in a hydrogen atom that releases the greatest amount of energy is from n=∞ to n=1.
---
In a hydrogen atom, the energy levels are quantized and can be described by the formula:
En=−13.6eVn2
where En is the energy of the level, and n is the principal quantum number (n = 1, 2, 3,...).
From the formula, we can see that energy is inversely proportional to the square of the principal quantum number n. This means:
- As n increases, the energy becomes less negative (increases).
- The lower the value of n, the more negative the energy, indicating a higher energy state when transitioning to a lower level.
To find the transition that releases the greatest amount of energy, we need to look for the transition from a higher energy level (higher n) to a lower energy level (lower n). The transition that releases the most energy occurs when an electron falls from the highest energy level (infinity) to the lowest energy level (n = 1).
The transition from n=∞ to n=1 can be calculated as follows:
- Energy at n=∞:
E∞=0eV
- Energy at n=1:
E1=−13.6eV
ΔE=E1−E∞=−13.6eV−0eV=−13.6eV
This indicates that 13.6 eV of energy is released.
The electronic transition in a hydrogen atom that releases the greatest amount of energy is from n=∞ to n=1.
Similar Questions
Knowledge Check
Which of the following electron transitions will require the largest amount of energy in a hydrogen atom?
AFrom n= 1 to n = 2BFrom n = 2 to n = 3CFrom n=∞ to n =1DFrom n =3 to n = 5.
The figure shows an energy level diagram for the hydrogen atom. Several transitions are marked as I, II, III,_____. The diagram is only indicative and not be scale.
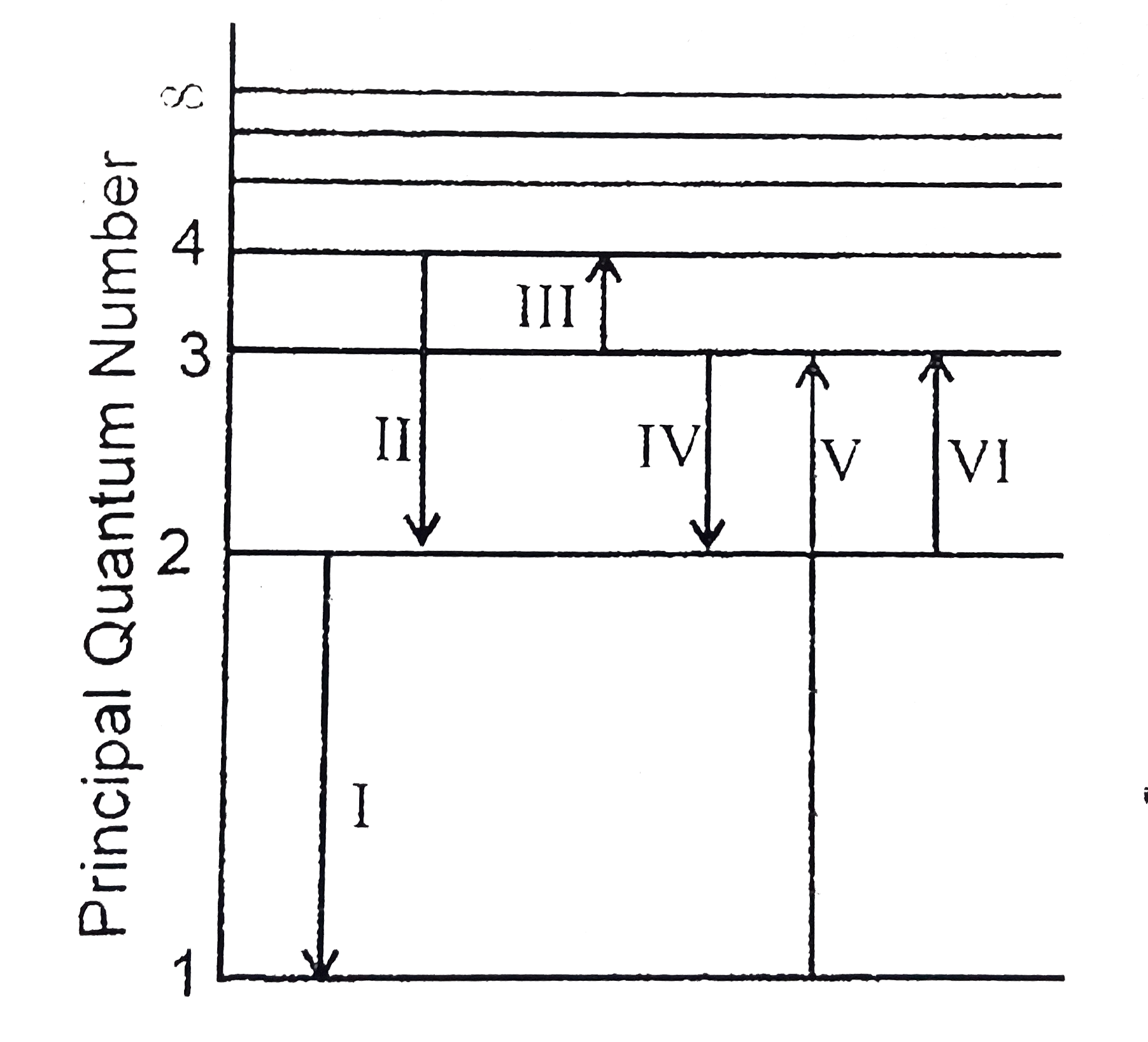
Which transition will occur when a hydrogen atom is irradiated with radiation of wavelength 103nm.
View SolutionThe figure shows an energy level diagram for the hydrogen atom. Several transitions are marked as I, II, III,_____. The diagram is only indicative and not be scale.

Which transition will occur when a hydrogen atom is irradiated with radiation of wavelength 103nm.
View SolutionIf the electron of a hydrogen atom is present in the first orbit. The total energy of the electrons is
View SolutionThe electron in a hydrogen atom at rest makes a transition from n=2 energy state to the n=1 ground state.
find the energy (eV) of the emitted photon.
View SolutionUsing Bohr's model , calculate the wavelength of the radiation emitted when an electron in a hydrogen atom makes a transition from the fourth energy level to the second energy level.
View SolutionIf potential energy of an electron in a hydrogen atom in first excited state is taken to be zero kinetic energy (in eV) of an electron in ground state will be
View SolutionThe electron in a hydrogen atom at rest makes a transition from n=2 energy state to the n=1 ground state.
Assuming that all of the energy corresponding to transition from n=2→n=1 is carried off by the photon. By setting the momentum of the system (atom + photon) equal to zero after the emission and assuming that the recoil energy of the atom is smaller compared with the n=2→n=1 energy level separation , find the energy of the recoiling hydrogen atom.
View Solution
