The approximate temperature at which 1 mol L−1 of a sample of pure ideal gas exhibits a pressure of 101.325 k Pa is
12.2 K
122 K
244 K
300 K
The correct Answer is:A
To find the approximate temperature at which 1 mol L−1 of a sample of pure ideal gas exhibits a pressure of 101.325 kPa, we can use the ideal gas equation:
Ideal Gas Equation:
PV=nRT
Where:
- P = pressure (in atm)
- V = volume (in liters)
- n = number of moles
- R = universal gas constant
- T = temperature (in Kelvin)
Step 1: Convert Pressure to atm
The pressure given is 101.325 kPa. We need to convert this to atm:
\( 1 \text{ atm} = 101.325 \text{ kPa} \)
Thus,
\( P = \frac{101.325 \text{ kPa}}{101.325 \text{ kPa/atm}} = 1 \text{ atm} \)
Step 2: Identify the Volume and Number of Moles
The volume \( V \) is given as 1 L, and the number of moles \( n \) is given as 1 mol.
Step 3: Use the Ideal Gas Constant
The value of the universal gas constant \( R \) is:
\( R = 0.0821 \text{ atm L mol}^{-1} \text{ K}^{-1} \)
Step 4: Rearrange the Ideal Gas Equation to Solve for Temperature
We can rearrange the ideal gas equation to solve for temperature \( T \):
\( T = \frac{PV}{nR} \)
Step 5: Substitute the Values into the Equation
Now, substituting the values we have:
\( T = \frac{(1 \text{ atm})(1 \text{ L})}{(1 \text{ mol})(0.0821 \text{ atm L mol}^{-1} \text{ K}^{-1})} \)
Step 6: Calculate the Temperature
Calculating the above expression:
\( T = \frac{1}{0.0821} \)
\( T \approx 12.2 \text{ K} \)
Final Answer
The approximate temperature at which 1 mol L−1 of a sample of pure ideal gas exhibits a pressure of 101.325 kPa is approximately 12.2 K.
---
PV=nRT
- P = pressure (in atm)
- V = volume (in liters)
- n = number of moles
- R = universal gas constant
- T = temperature (in Kelvin)
The pressure given is 101.325 kPa. We need to convert this to atm:
\( 1 \text{ atm} = 101.325 \text{ kPa} \)
Thus,
\( P = \frac{101.325 \text{ kPa}}{101.325 \text{ kPa/atm}} = 1 \text{ atm} \)
The volume \( V \) is given as 1 L, and the number of moles \( n \) is given as 1 mol.
The value of the universal gas constant \( R \) is:
\( R = 0.0821 \text{ atm L mol}^{-1} \text{ K}^{-1} \)
We can rearrange the ideal gas equation to solve for temperature \( T \):
\( T = \frac{PV}{nR} \)
Now, substituting the values we have:
\( T = \frac{(1 \text{ atm})(1 \text{ L})}{(1 \text{ mol})(0.0821 \text{ atm L mol}^{-1} \text{ K}^{-1})} \)
Calculating the above expression:
\( T = \frac{1}{0.0821} \)
\( T \approx 12.2 \text{ K} \)
The approximate temperature at which 1 mol L−1 of a sample of pure ideal gas exhibits a pressure of 101.325 kPa is approximately 12.2 K.
Similar Questions
Under what conditions will a pure sample of an ideal gas not only exhibit a pressure of 1atm but also a concentration of 1mollitre−1
[R=0.082 iltre atm mol−1K−1]View SolutionTemperature of 1mol of a gas is increased by 1∘ at constant pressure. The work done is
View SolutionTemperature of 1mol of a gas is increased by 1∘ at constant pressure. The work done is
View Solution50 cal of heat is required to raise the temperature of 1 mole of an ideal gas from 20∘C to 25∘C, while the pressure of the gas is kept constant. The amount of heat required to raise the temperature of the same has through same temperature range at constant volume is ( R = 2 cal/mol/K)
View SolutionTwo moles of an ideal gas are allowed to expand from a volume of 10dm3 to 2 m3 at 300 K against a pressure of 101.325 kPa. Calcualte the work done.
View SolutionThe volume of 1 mol of a gas at standard temperature and pressure is .
View SolutionContainer A holds an ideal gas at a pressure 1×105 Pa and at 300 K. Container B whose volume is 4 times the volume of A has the same ideal gas at 400 K and at a pressure of 5×105
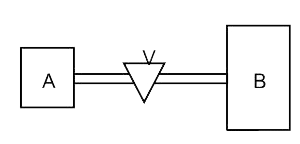
Before the valve V is opened, the ratio of number of moles of gas in A and B is :View SolutionThe internal pressure loss of 1mol of van der Waals gas over an ideal gas is equal to
View SolutionA gas is allowed to expand at constant temperature from a volume of 1.0L to 10.0L against an external pressure of 0.50 atm. If the gas absorbs 250J of heat from the surroundings, what are the values of q and ΔE ? (Given 1Latm=101J)
View SolutionA gas cylinder having a volume of 25.0 L contains a mixtue of butane CH3(CH2)2CH3 and isobutane (CH3)3CH in the ratio of 3 : 1 by moles. If the pressure inside the cylinder is 6.78×106 pa and the temperature is 298 K, calculate the number of molecular of each gas assuming ideal gas behaviour. (1 atm = 101325 Pa)
View Solution

