In man the sperms released from the testis take the following route to reach the urethra
Vasa efferentia, Bidder canal, uriniferous tubules and nephrostome
Vasa efferntia, epididymis, vasa deferens and urethra.
Vasa efferntia, Bidder's canal nephrostome
Vasa efferntia, collecting tubules and bidder canals.
The correct Answer is:B
To determine the route that sperm takes from the testes to the urethra in males, we can follow these steps:
1. Sperm Production: Sperm is produced in the seminiferous tubules located within the testes.
2. Vasa Efferentia: After sperm is produced, it moves from the seminiferous tubules into a network of small ducts called the vasa efferentia.
3. Epididymis: The vasa efferentia then transport the sperm to the epididymis, which is a coiled tube where sperm matures and is stored.
4. Vas Deferens: From the epididymis, the matured sperm travels into the vas deferens, which is a muscular tube that transports sperm from the epididymis to the ejaculatory duct.
5. Ejaculatory Duct: The vas deferens joins with the seminal vesicle to form the ejaculatory duct, where sperm mixes with seminal fluid to form semen.
6. Urethra: Finally, the semen is expelled through the urethra during ejaculation.
Final Route:
Thus, the complete route of sperm from the testes to the urethra is:
- Vasa Efferentia → Epididymis → Vas Deferens → Urethra
Answer:
The correct option is B. Vasa Efferentia, Epididymis, Vas Deferens, and Urethra.
---
Thus, the complete route of sperm from the testes to the urethra is:
- Vasa Efferentia → Epididymis → Vas Deferens → Urethra
The correct option is B. Vasa Efferentia, Epididymis, Vas Deferens, and Urethra.
Topper's Solved these Questions
Similar Questions
A balloon starts rising from the ground with a constant acceleration of 1.25m/s2. After 8 s, a stone is released from the balloon. Find the time taken by the stone to reach the ground. (Take g=10m/s2)
View SolutionA stone is dropped from a certain heitht which can reach the ground in 5s. It is stopped aftre 3s of its fall and then it is again released. The total time taken by the stone to reach the ground will be .
View Solution
Knowledge Check
The height of a tower is h meter, A body is thrown from the top of tower vertically upwards with some speed, it takes t1 seconds to reach the ground. Another body thrown from the top of tower with same speed downwards and takes t2 seconds to reach the ground. If third body, released from same place takes 't' second to reach the ground, then
At=t1+t22Bt=t1t2C2t=1t1+1t2Dt=√(t1t2)
A balloon from rest accelerates uniformly upward with 'a' ms−2, for t seconds of time. A stone is released from the balloon. Now, read the following statements to pick the right ones.
(a) The stone's initial velocity is zero, relative to balloon
(b) The stone's initial velocity is nonzero, relative to earth
( c) The time taken to reach the ground from the balloon's frame of reference is inversely proportional to √(a+g)
(d) The time take to reach the ground from earth's frame of reference is directly proportional to √(a+g)
View Solution(A):A metal ball and a wooden ball of same radius are dropped from the same height in vaccum reach the ground same time.
(R ):In vacuum all the bodies dropped from same height take same time to reach the round.
View SolutionIn the figure shown, a particle is released from the position A on a smooth track. When the particle reaches at B, then normal reaction on it by the track. When the particle reaches at B, then normal reaction on it by the track is
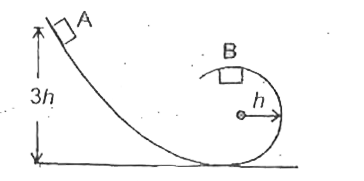
View SolutionList the structures, in their correct sequence, through which the sperms must pass from the time they are produced in the testes to the time they leave the urethra.
View SolutionFrom the top of a tower, a stone is thrown up and it reaches the ground in time t1A second stone is thrown down with the same speed and it reaches the ground in time t2 A third stone is released from rest and it reaches the ground in time The correct relation between t1,t2 and t3 is :
View SolutionA particle is thrown vertically up from the top of a building of height 20m with initial velocity u. A second particle is released from the same point 1 second later. If both particles reach the ground at the same instant ,3u=m/s. [Takeg=10m/s2]
View Solution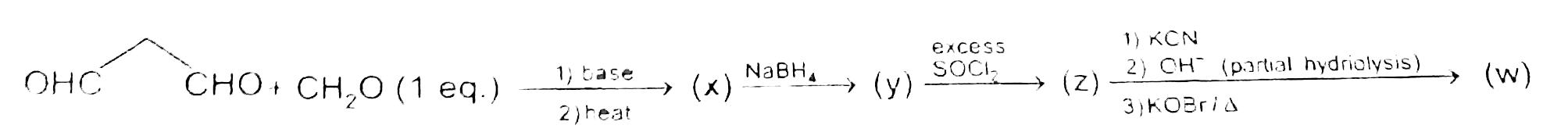
Synthesis of propellane takes placed by the following route.
Q. The product (w) is:
View Solution
