Question

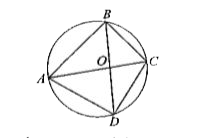
In a circle with centre O, AC and BD are two chords. AC and BD meet at E when produced. If AB is the diameter and $\angle AEB={68}^{\circ }$, then the measure of $\angle DOC$ is:

Options

A. `30^@`
B. `44^@`
C. `32^@`
D. `22^@`

Explanation

To solve the problem, we need to find the measure of angle DOC in the given circle with center O, where AB is the diameter and angle AEB is 68 degrees.

1. Identify the Given Information:
- We have a circle with center O.
- AB is the diameter of the circle.
- AC and BD are two chords that intersect at point E when extended.
- Angle AEB = 68 degrees.

2. Use the Property of Angles in a Circle:
- According to the properties of circles, when two chords intersect, the angle formed at the intersection point (angle AEB in this case) has a specific relationship with the angle formed at the center (angle DOC).
- The relationship can be expressed as:
\( \text{Angle DOC} = 180^\circ - 2 \times \text{Angle AEB} \)

3. Substitute the Known Value:
- We know that angle AEB = 68 degrees. We can substitute this value into the equation:
\( \text{Angle DOC} = 180^\circ - 2 \times 68^\circ \)

4. Calculate the Value:
- First, calculate \(2 \times 68^\circ\):
\( 2 \times 68^\circ = 136^\circ \)
- Now, substitute this back into the equation for angle DOC:
\( \text{Angle DOC} = 180^\circ - 136^\circ = 44^\circ \)

5. Final Answer:
- Therefore, the measure of angle DOC is:
\( \text{Angle DOC} = 44^\circ \)