In a triangle PQR, P is the largest angle and . Further the incircle of the triangle touches the sides PQ, QR and RP at N, L and M respectively, such that the lengths of PN, QL and RM are consecutive even integers. Then possible length(s) of the side(s) of the triangle is (are) Solution in Bengali
16
18
24
22

Let
Adding we get,
. So,
Now,
or
or
So, sides are 22, 20, 18
Topper's Solved these Questions
Similar Questions
The pair of lines joining the origin to the points of intersection of the curves
ax2+2hxy+by2+2gx=0 and
a'x2+2h'xy+b'y2+2g'x=0
will be at right angles to one another , if
View SolutionIf a triangle ABC, the incircle touches the sides BC, CA and AB respectively at D, E and F. If the radius of incircle is 4 units and if BD, CE and AF are consecutive integers, Find the lengths of sides of the triangle ABC.
View SolutionThe sides of a right-angled triangle are integers. The length of one of the sides is 12. The largest possible radius of the incircle of such a triangle is-
View SolutionΔPQR कि भुजाओ PR और QR पर क्रमशः बिंदु S और T इस प्रकार स्थित है कि ∠P=∠RTS है दर्शाइए कि ΔRPQ~ΔRTS है
View SolutionΔPQR में P वृहत्तम कोण है। तथा cosp=13 इसके अतिरिक्त त्रिभुज का अन्तु:वृत्त भुजाओं PQ,QR तथा RP को क्रमशः N,L तथा M पर इस तरह स्पर्श करता है। कि PN, QL तथा RM की लम्बाईयाँ क्रमागत सम्पूर्ण संख्याऍं है। तक त्रिभुज की भुजा (भुजाओं ) की सम्भावित लम्बाइयाँ क्या है।
View SolutionIn a triangle PQR ,P is the largest angle and cos P = 13 . further the triangle toches the side PQ. QR and RP at N , L and M respectively , such that the lengths of PN , QL , and RM are consecutive even integers. Then possible length(s) of the side(s) of the triangle is(are)
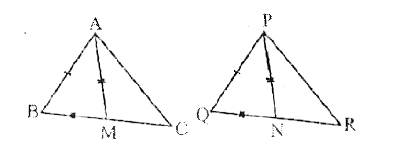
View SolutionTwo sides AB and BC and median AM of one triangle ABC are respectively equal to sides PQ and QR and median PN of Δ PQR. Show that
ΔABM≅ΔPQN
(ii) ΔABC≅ΔPQR
View Solutionत्रिभुज PQR, में P बृहत्तम कोण है तथा cosP=13 इसके अतिरिक्त त्रिभुज का अन्तःवृत्त भुजाओं PQ, QR तथा RP को क्रमशः N, L तथा M पर इस तरह स्पर्श करता है कि PN, QL तथा RM की लम्बाईयाँ क्रमागत सम पूर्णसंख्याएँ है तब त्रिभुज की भुजा (भुजाओं) की संभावित लम्बाई (लम्बाईयाँ) है (है) : (i) 16 (ii) 18 (iii) 24 (iv) 22
View SolutionS is any point on side QR of a ΔPQR. Show that PQ+QR+RP>2PS .
View SolutionP is triangle angle in triangle PQR. And cos P = (1)/(3) . In addition, the inner sides of the triangle touch PQ, QR and RP on N, L and M respectively. That the lengths of PN, QL and RM are consecutive even numbers, then the possible length of the side of the triangle is.
View Solutionत्रिभुज PQR, में P बृहत्तम कोण है तथा cosP=13 इसके अतिरिक्त त्रिभुज का अन्तःवृत्त भुजाओं PQ, QR तथा RP को क्रमशः N, L तथा M पर इस तरह स्पर्श करता है कि PN, QL तथा RM की लम्बाईयाँ क्रमागत सम पूर्णसंख्याएँ है तब त्रिभुज की भुजा (भुजाओं) की संभावित लम्बाई (लम्बाईयाँ) है (है) : (i) 16 (ii) 18 (iii) 24 (iv) 22
View SolutionΔPQR ਦੀਆਂ ਭੁਜਾਵਾਂ PR ਅਤੇ QR ਉਤੇ ਕ੍ਰਮਵਾਰ ਬਿੰਦੂ S ਅਤੇ T ਇਸ ਤਰ੍ਹਾਂ ਸਥਿਤ ਹਨ ਕਿ ∠P=∠RTS ਹੈ।ਦਿਖਾਉ ਕਿ ΔRPQ~ΔRTS ਹੈ।
View Solutionਤ੍ਰਿਭੁਜ ABC ਦੀਆਂ ਦੋ ਭੁਜਾਵਾਂ AB,BC ਅਤੇ ਮੱਧਿਕਾ AM ਕ੍ਰਮਵਾਰ ਦੂਸਰੀ ਤ੍ਰਿਭੁਜ PQR ਦੀਆਂ ਭੁਜਾਵਾਂ PQ ਅਤੇ QR ਅਤੇ ਮੱਧਿਕਾ PN ਦੇ ਬਰਾਬਰ ਹਨ (ਵੇਖੋ ਚਿੱਤਰ 7.40)। ਸਿੱਧ ਕਰੋ ਕਿ △ABC≅△PQR
View SolutionIn the figure triangle PQR Prove that if S is any one point on the side QR, PQ+QR+RP gt 2PS
View SolutionPQR is an equilateral triangle. MN is drawn parallel to QR such that M is on PQ and N is on PR. If PN = 6 cm, then the length of MN is
View SolutionPQR is an isosceles triangle with sides PQ=PR=45cmand QR=72cm. PN is a median to base QR. What will be the length (in cm.) of PN?
View Solution
