If" f, is a continuous function with ∫x0f(t)dt→∞ as ∣x↦∞then show that every line y=mx intersects the curve y2+∫x0f(t)dt=2Solution in Bengali
Step by step video & image solution for If" f, is a continuous function with int_0^x f(t) dt->oo as |x|->oothen show that every line y = mx intersects the curve y^2 + int_0^x f(t) dt = 2 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The least value of a∈R for which 4ax2+1x≥1, for all x>0, is
View SolutionThe points with position vectors →x+→y,→x−→yand→x+λ→y are collinear for all real values of λ.
View SolutionLet f be a non-negative function defined on the interval .[0,1].If ∫x0√1−(f'(t))2.dt=∫x0f(t).dt, 0≤x≤1 and f(0)=0,then
View SolutionIf f, is a continuous function with int_(0)^(x)f(t)dt rarr oo as |x|->oo then show that every line y=mx intersects the curve y^(2)+int_(0)^(x)f(t)dt=2
View Solutionयदि 'f' एक संतत् फलन है तथा ∫x0f(t)dt→∞ जब |x|→∞ हो, तो प्रदर्शित कीजिए कि प्रत्येक रेखा y = m x वक्र y2+∫x0f(t)dt=2! को प्रतिच्छेद करती है।
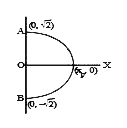
View SolutionIf" f, is a continuous function with ∫x0f(t)dt→∞ as ∣x↦∞then show that every line y=mx intersects the curve y2+∫x0f(t)dt=2
View SolutionLet f:[0,∞)→R be a continuous strictly increasing function, such that f3(x)=∫x0t.f2(t)dt for every x≥0. Then value of f(6) is_______
View Solutionf(x)=∫x0f(t)dt=x+∫1xtf(t)dt, then the value of f(1) is
View SolutionIf" f, is a continuous function with ∫x0f(t)dt→∞ as ∣x↦∞then show that every line y=mx intersects the curve y2+∫x0f(t)dt=2
View SolutionLet f:[0,∞)→R be a continuous strictly increasing function, such that f3(x)=∫x0t⋅f2(t)dt for every x≥0. Then value of f(6) is_______
View SolutionIf f(x)=∫x0{f(t)}−1dtand∫10{f(t)}−1=√2, then
View SolutionIf f(t) is an odd function then ∫x0f(t(dt is
View SolutionIf ∫x0f(t)dt=x+∫lxtf(t)dt, then the value of f(1) is :
View SolutionLet f:(0,∞)→R and F(x)=∫x0f(t)dt.
If F(x2)=x2(1+x), then f(4) equals :
View SolutionIf" f, is a continuous function with ∫x0f(t)dt→∞ as ∣x↦∞then show that every line y=mx intersects the curve y2+∫x0f(t)dt=2
View Solution
