A line L passing through the focus of the parabola y2=4(x−1) intersects the parabola at two distinct points. If m is the slope of the line L,then Solution in Bengali
Step by step video & image solution for A line L passing through the focus of the parabola y^2=4(x-1) intersects the parabola at two distinct points. If m is the slope of the line L , then by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - All Questions
877 Videos
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS BOOK - CENGAGE PUBLICATIONCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type
11 Videos
Similar Questions
The equation esinx−e−sinx−4=0 has
View SolutionLet →a=a1ˆi+a2ˆj+a3ˆk,→b=b1ˆi+b2ˆj+b3ˆk and →c=c1ˆi+c2ˆj+c3ˆk be three non- zero vectors such that →c is a unit vectors perpendicular to both the vectors →a and →b. If the angle between →a and →b is π6 then
∣∣ ∣∣a1a2a3b1b2b3c1c2c3∣∣ ∣∣2 is equal to
View SolutionIf xl+ym=1 is any line passing through the intersection point of the lines xa+yb=1 and xb+ya=1 then prove that 1l+1m=1a+1b
View SolutionStatement 1: The point of intersection of the tangents at three distinct points A,B,andC on the parabola y2=4x can be collinear. Statement 2: If a line L does not intersect the parabola y2=4x, then from every point of the line, two tangents can be drawn to the parabola.
View SolutionA line L passing through the focus of the parabola y2=4(x−1) intersects the parabola at two distinct points. If m is the slope of the line L, then −1<m<1 (b) m<−1 or m>1 m∈R (d) none of these
View SolutionA line L passing through the focus of the parabola (y−2)2=4(x+1) intersects the two distinct point. If m be the slope of the line I,, then
View Solution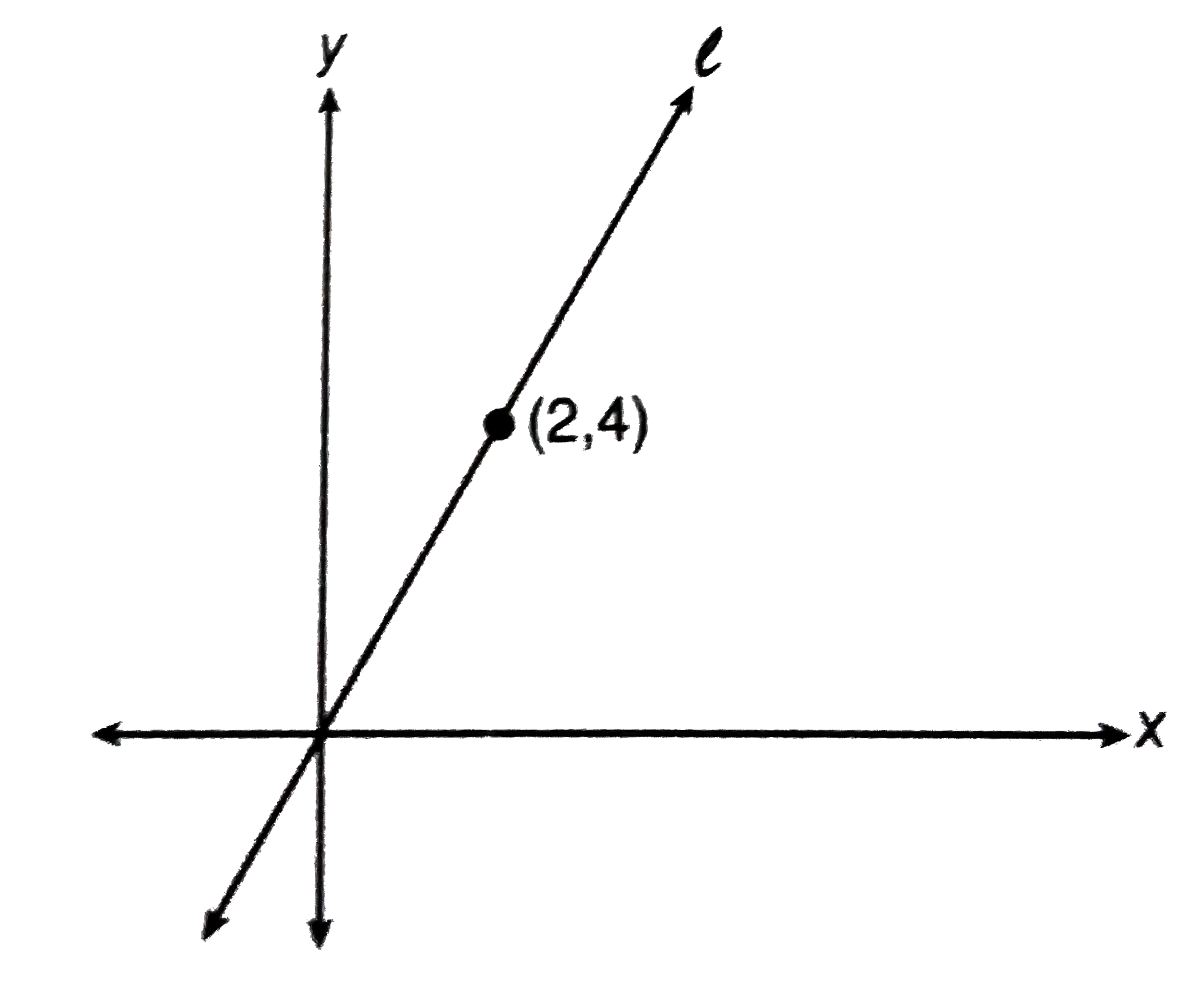
In the accompanying figure. Line l passes through the origin and the point (2, 4). Line m (not shown) is perpendicular to line l at (2, 4). Line m intersects the x-axis at which point?
View SolutionThe graph of line l in the xy-plane passes through the points (2,5)and(4,11). The graph of line m has a slope of -2 and an x-intercept of 2. If point (x, y) is the point of intersection of line l and m, what is the value of y?
View SolutionThe locus of point of intersection of any tangent to the parabola y2=4a(x−2) with a line perpendicular to it and passing through the focus, is
View SolutionIf xl+ym=1 is any line passing through the intersection point of the lines xa+yb=1 and xb+ya=1 then prove that 1l+1m=1a+1b
View SolutionStatement 1: The point of intersection of the tangents at three distinct points A,B,andC on the parabola y2=4x can be collinear. Statement 2: If a line L does not intersect the parabola y2=4x, then from every point of the line, two tangents can be drawn to the parabola.
View SolutionA line L passing through the focus of the parabola y2=4(x−1) intersects the parabola at two distinct points. If m is the slope of the line L, then (a) −1<m<1 (b) m<−1 or m>1 (c) m∈R (d) none of these
View SolutionA line L passing through the focus of the parabola y2=4(x−1) intersects the parabola at two distinct points. If m is the slope of the line L, then −1<m<1 (b) m<−1 or m>1 m∈R (d) none of these
View SolutionA line L passes through the point (1, 1) and (2,0). Another line M which is perpendicular to L passes through the point [12,0]. Then the area of the triangle formed by the lines L, M and y-axis is
View Solutionरेखा y=x−2 परवलय y2=8x को बिन्दुओं A और B पर काटती है। A और B पर परवलय के अभिलम्ब G नी प्रतिच्छेदित होते है। G से गुजरने वाली एक रेखा परवलय को बिन्दु C पर समकोण पर प्रतिच्छेद करती है और A तथा B पर स्पर्श रेखाएँ बिन्दु T पर प्रतिच्छेद करती है।
A और B से गुजरने वाले वृत्त की न्यूनतम त्रिज्या है
View SolutionA line L passing through the focus of the parabola (y−2)2=4(x+1) intersects the two distinct point. If m be the slope of the line I,, then
View Solution
