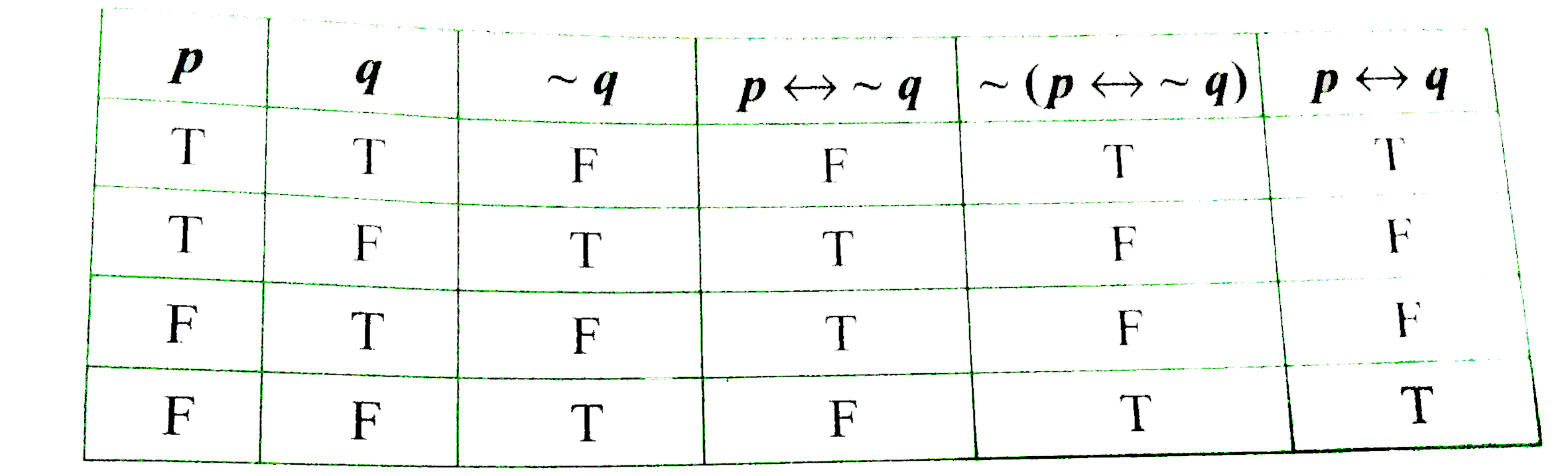
The statement~(p↔~q) isSolution in Bengali
equivalent to p↔q
equivalent to ~p↔q
a tautology
a fallacy
The correct Answer is:A
Topper's Solved these Questions
- MATHMETICAL REASONINGBOOK - CENGAGE PUBLICATIONCHAPTER - MATHMETICAL REASONINGEXERCISE - Single correct answer type
38 Videos
- LOGARITHM AND ITS PROPERTIESBOOK - CENGAGE PUBLICATIONCHAPTER - LOGARITHM AND ITS PROPERTIESEXERCISE - JEE ADVANCED
1 Videos
- MATRICESBOOK - CENGAGE PUBLICATIONCHAPTER - MATRICESEXERCISE - All Questions
509 Videos
Similar Questions
Which of the following is not a statement.
View SolutionLet (x0,y0) be the solution of the following equations: (2x)1n2=(3y)1n3 31nx=21ny The x0 is 16 (b) 13 (c) 12 (d) 6
View SolutionIn which of the following type of matrix inverse does not exist always? a. idempotent b. orthogonal c. involuntary d. none of these
View SolutionStatements -1 : ~(pharr~q) is equivalent to p harr q Statement-2: (~ p harr ~q) is a tautology.
View SolutionThe statement ~(p↔~q) is
View Solutionवक्तब्य I~(p⇔~q),p⇒q के तार्किक समतुल्य है
वक्तब्य II~(p⇔~q) एक पुनरुक्ति है
View SolutionThe statement ~(p↔~q) is -
View SolutionIf p and q are two statements , then which of the following statements is not equivalent to p⇔(p⇒q) ?
View SolutionThe statement ~(p⇔q) is not equivalent to
View SolutionThe statement p⇔q is not equivalent to
View SolutionThe statement (~(p⇔q))∧p is equivalent to
View SolutionThe statement p⇔q is equal to -
View SolutionThe compound statement (p⇔q)∨(p⇔~q) is logically equivalent to
View SolutionStatement-1: ~(p↔~q) is equivalent to (p↔q).
Statement-2: ~(p↔~q) is a tautology.
View SolutionStatement I ~(phArr ~q) ,phArr q K is the logical equivalent. Statement II ~(phArr ~q) There is a repetition.
View SolutionThe statement ~(p↔~q) is
View Solution