Consider the frequency distribution, where A is a positive interger : variance is 160.
xA2A3A4A5A6Af211111.Solution in Bengali
The correct Answer is:C
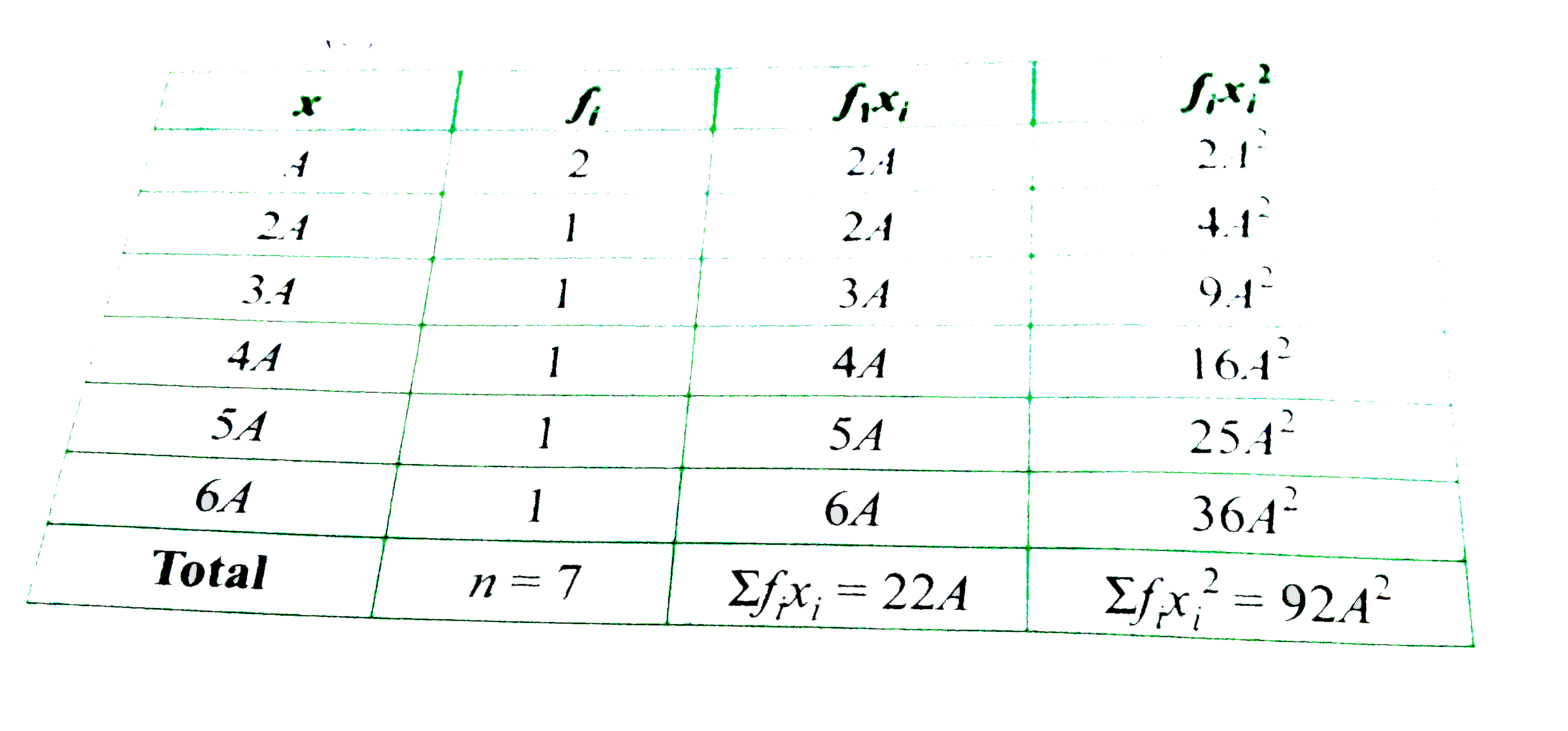
∴σ2=∑fix2in−(∑fixin)2
⇒160=92A27−(22A7)2
⇒160=92A27−484A249
⇒160=(644−484)A249
⇒160=160A249
⇒A2=49
∴A=7
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - STATISTICSEXERCISE - Exercise 11.15videos
STATISTICS
- BOOK - CENGAGE PUBLICATIONCHAPTER - STATISTICSEXERCISE - Exercise 11.26videos
STATISTICS
- BOOK - CENGAGE PUBLICATIONCHAPTER - SOLUTIONS AND PROPERTIES OF TRIANGLE EXERCISE - Comprehension Type6videos
SOLUTIONS AND PROPERTIES OF TRIANGLE
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
Similar Questions
In a class of 100 students there are 70 boys whose average marks in subject are 75. If the average marks of the complete class are 72, then the average marks of the girls
04:05View SolutionIn an experiment with 15 observations of x the following results were available ∑x2=2830∑x=170 one observation that was 20 was found to be wrong and it was replaced by its correct value of 30 Then the corrected variance is (1) 8.33 (2) 78 (3) 188.66 (4) 177.33
02:08View SolutionLet ABC be a triangle in which the line joining the circumecentre and incentre is parallel to base BC of the triangle. Then answer the following questions :
Then range of ∠A is03:27View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
03:16View Solutiondet[[x+a,x+2a,x+3ax+2a,x+3a,x+4ax+4a,x+5a,x+6a]]=0
02:54View SolutionProve that : ∣∣ ∣∣x+ax+2ax+3ax+2ax+3ax+4ax+4ax+5ax+6a∣∣ ∣∣=0
04:09View SolutionConsider the frequency distribution, where A is a positive interger :
xA2A3A4A5A6Af21111102:29View Solutionसिद्ध कीजिए कि ∣∣ ∣∣x+ax+2ax+3ax+2ax+3ax+4ax+4ax+5ax+6a∣∣ ∣∣=0
06:31View Solutionसिद्ध कीजिए कि
sinAsin2A+sin3Asin6A−sin4Asin5A=005:49View Solution∣∣ ∣∣a+b2a+b3a+b2a+b3a+b4a+b4a+b5a+b6a+b∣∣ ∣∣=0
03:04View SolutionGiven, a2+2a+2,3a2+6a+6 and 4a2+5a+4 are in A.P. Find the value or values of a.
03:28View Solutionसिद्ध कीजिए की:
sin2Asin3A−sin4Asin5A+sin3Asin6Acos2Acos3A−cos4Acos5A+cos3Acos6A=tanAtan4A04:34View SolutionProve that:cos2Acos3A−cos2Acos7A+cosAcos10Asin4Asin3A−sin2Asin5A+sin4Asin7A
=cot6Acot5A04:54View SolutionProve that (2a, 4a), (2a, 6a), and (2a +√3a, 5a) are the vertices of an equilateral triangle.
05:06View SolutionConsider the frequency distribution, where A is a positive interger : variance is 160.
xA2A3A4A5A6Af211111.03:32View SolutionIf a is positive integer and the frequency distribution: x: , A, 2a, 3a, 4a, 5a, 6a f: , 2, 1, 1, 1, 1, 1 and variance is 160 then find the value of a.
02:15View Solution

