A parallelepiped S has base points A,B,CandD and upper face points A′,B′,C′,andD' . The parallelepiped is compressed by upper face A'B'C'D' to form a new parallepiped T having upper face points A'',B'',C''andD'' . The volume of parallelepiped T is 90 percent of the volume of parallelepiped S. Prove that the locus of A'' is a plane.Solution in Bengali
S is the parallelepiped with base point A,B,CandD and upper face points A',B',C'andD'. Let its volume ve Vs. By compressing it by upper face A',B',C'andD', a new parallelepiped T is formed whose upper face points are now A'',B'',C''andD''. Let its volume be VT.
Let h be the height of original parallelepiped S. Then
VS=(area of ABCD)×h (i)
Let equation of plane ABCD be ax+by+cz+d=0andA''(α,β,γ).
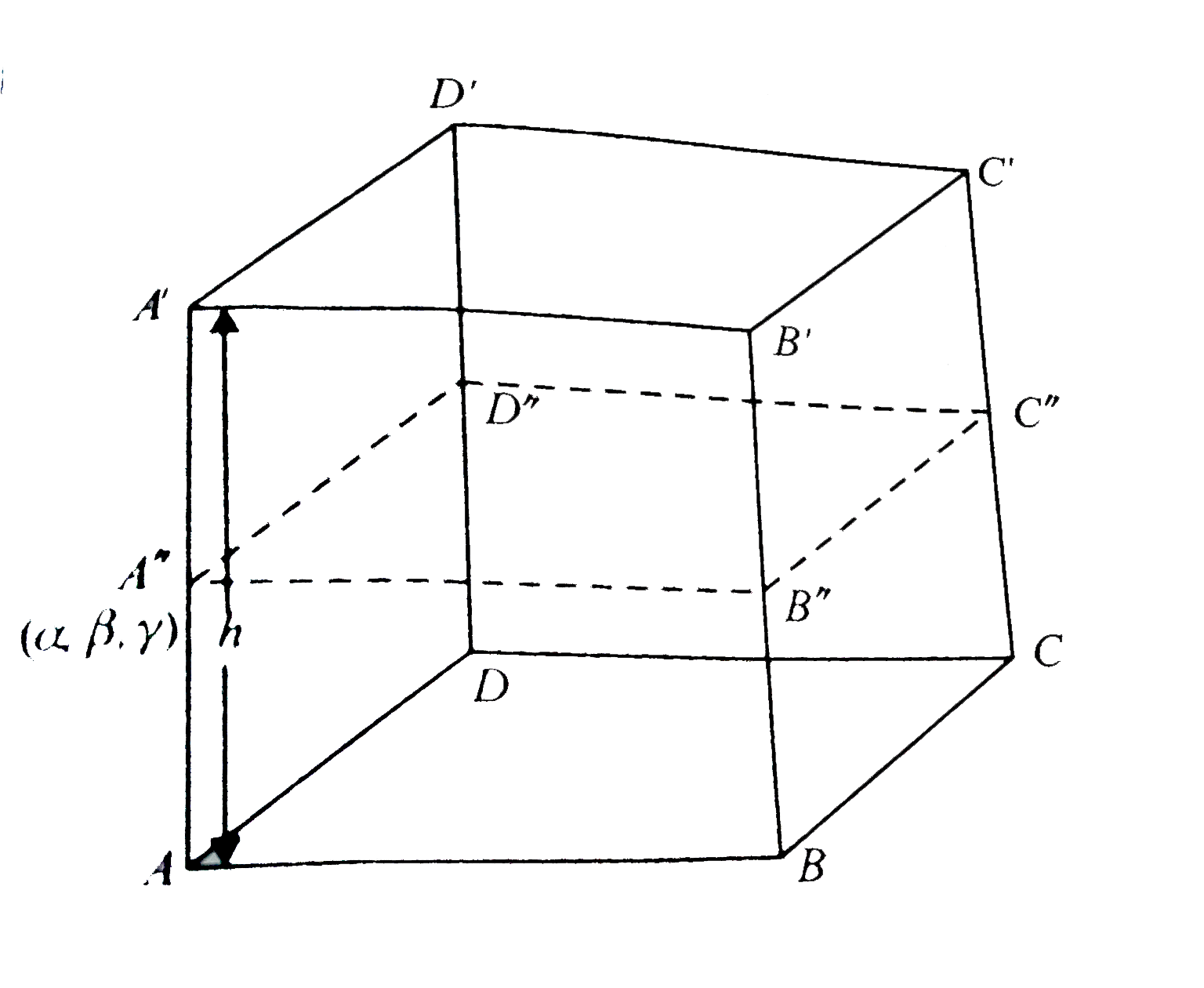
Then the height of the new parallelepiped T is the length of the perpendicular from A'' to ABCD, i.e., aα+η+cγ+d√a2+b2+c2. Therefore
VT=(ar ABCD)×(aα+η+cγ+d)√a2+b2+c2 (ii)
But given that VT=90100Vs (iii)
From (i), (ii) and (iii), we get
aα+η+cγ+d√a2+b2+c2=0.9h
or aα+η+cγ+d−0.9h√a2+b2+c2=0
Therefore, the locus of A''(α,β,γ) is ax+by+cz+d−0.9h√a2+b2+c2=0, which is a plane parallel to ABCD. Hence proved.
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - ARCHIVES SINGLE CORRECT ANSWER TYPE9videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - ARCHIVES MULTIPLE CORRECT ANSWERS TYPE4videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - INTEGER TYPE10videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGE PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - All Questions291videos
THREE DIMENSIONAL GEOMETRY
- BOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Numerical value type)4videos
TRIGONOMETRIC EQUATIONS
Similar Questions
The value of k such that x−41=y−21=z−k2 lies in the plane 2x−4y=z=7 is a. 7 b. -7 c. no real value d. 4
04:13View SolutionTwo lines L1x=5,y3−α=z−2andL2:x=αy−1=z2−α are coplanar. Then α can take value (s) a. 1 b. 2 c. 3 d. 4
04:07View SolutionFind the number of sphere of radius r touching the coordinate axes.
07:43View SolutionFind the angle between the line whose direction cosines are given by l+m+n=0andl2+m2−n2=0.
03:07View SolutionThe number of all possible values of θ, where 0<θ<π, for which the system of equations (y+z)cos3θ=(xyz)sin3θ,xsin3θ=2cos3θy+2sin3θzand(xyz)sin3θ=(y+2z)cos3θ+ysin3θ have a solution (x0,y0,z0) wiith y0z0≠0 is
06:11View SolutionThe condition that one of the straight lines given by the equation ax2+2hxy+by2=0 may coincide with one of those given by the equation a′x2+2h′xy+b′y2=0 is (ab′−a′b)2=4(ha′−h′a)(bh′−b′h) (ab′−a′b)2=(ha′−h′a)(bh′−b′h) (ha′−h′a)2=4(ab′−a′b)(bh′−b′h) (bh′−b′h)2=4(ab′−a′b)(ha′−h′a)
02:18View SolutionIf the normal to the given hyperbola at the point (ct,ct) meets the curve again at (ct′,ct′), then (A) t3t′=1 (B) t3t′=−1 (C) tt′=1 (D) tt′=−1
03:02View SolutionA parallelepiped S has base points A,B,CandD and upper face points A′,B′,C′,andD' . The parallelepiped is compressed by upper face A'B'C'D' to form a new parallepiped T having upper face points A,B,Ca n dD . The volume of parallelepiped T is 90 percent of the volume of parallelepiped S. Prove that the locus of A is a plane.
06:09View SolutionUsing determinants prove that the points (a, b), (a′, b′)and (a−a′, b−b′) are collinear if ab′=a′b.
03:52View Solution|(bc,bcprime+bprime c, bprime c prime),(ca,ca prime+c prime a,c prime a prime),(ab, ab prime+a prime b,a prime b prime)| is equal to
08:47View SolutionIf (ax2+bx+c)y+(a′x2+b′x2+c′)=0 and x is a rational function of y, then prove that (ac′−a′c)2=(ab′−a′b)×(bc′−b′c).
08:13View SolutionThe condition that one of the straight lines given by the equation ax2+2hxy+by2=0 may coincide with one of those given by the equation a′x2+2h′xy+b′y2=0 is (ab′−a′b)2=4(ha′−h′a)(bh′−b′h) (ab′−a′b)2=(ha′−h′a)(bh′−b′h) (ha′−h′a)2=4(ab′−a′b)(bh′−b′h) (bh′−b′h)2=4(ab′−a′b)(ha′−h′a)
07:16View SolutionThe condition that one of the straight lines given by the equation ax2+2hxy+by2=0 may coincide with one of those given by the equation a′x2+2h′xy+b′y2=0 is (ab′−a′b)2=4(ha′−h′a)(bh′−b′h) (ab′−a′b)2=(ha′−h′a)(bh′−b′h) (ha′−h′a)2=4(ab′−a′b)(bh′−b′h) (bh′−b′h)2=4(ab′−a′b)(ha′−h′a)
03:46View SolutionIf the normal to the given hyperbola at the point (ct,ct) meets the curve again at (ct′,ct′), then (A) t3t′=1 (B) t3t′=−1 (C) tt′=1 (D) tt′=−1
06:51View SolutionA parallelepiped S has base points A,B,CandD and upper face points A′,B′,C′,andD' . The parallelepiped is compressed by upper face A'B'C'D' to form a new parallepiped T having upper face points A'',B'',C''and D'' . The volume of parallelepiped T is 90 percent of the volume of parallelepiped S. Prove that the locus of A is a plane.
08:21View SolutionA parallelepiped S has base points A,B,CandD and upper face points A′,B′,C′,andD' . The parallelepiped is compressed by upper face A'B'C'D' to form a new parallepiped T having upper face points A,B,Ca n dD . The volume of parallelepiped T is 90 percent of the volume of parallelepiped S. Prove that the locus of A is a plane.
03:50View Solution

