Let be a continuous onto function satisfying . If then the minimum number of roots of the equation isSolution in Bengali
f(x) is an odd function.
Since the points (-3, 2) and (5, 4) lie on the curve, (3, -2) and (-5, -4) will also lie on the curve.
For minimum number of roots, graph of the continuous function f(x) is as follows.

From the above graph of f(x), it is clear that equation f (x) = 0 has at least three real roots.
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - GETTING STARTED WITH GRAPHSEXERCISE - ILLUSTRATION 1.121videos
GETTING STARTED WITH GRAPHS
- BOOK - CENGAGE PUBLICATIONCHAPTER - GETTING STARTED WITH GRAPHSEXERCISE - ILLUSTRATION 1.131videos
GETTING STARTED WITH GRAPHS
- BOOK - CENGAGE PUBLICATIONCHAPTER - GETTING STARTED WITH GRAPHSEXERCISE - ILLUSTRATION 1.101videos
GETTING STARTED WITH GRAPHS
- BOOK - CENGAGE PUBLICATIONCHAPTER - FUNCTIONSEXERCISE - Comprehension Type7videos
FUNCTIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - GRAPH OF INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Exercises18videos
GRAPH OF INVERSE TRIGONOMETRIC FUNCTIONS
Similar Questions
Let f:R→Randg:R→R be two one-one and onto function such that they are the mirror images of each other about the line y=a.Ifh(x)=f(x)+g(x),thenh(x) is one-one and onto only one-one and not onto only onto but not one-one neither one-one nor onto
04:42View SolutionCheck weather the following function/functions is/are periodic or not? Find the period in case the function is periodic.
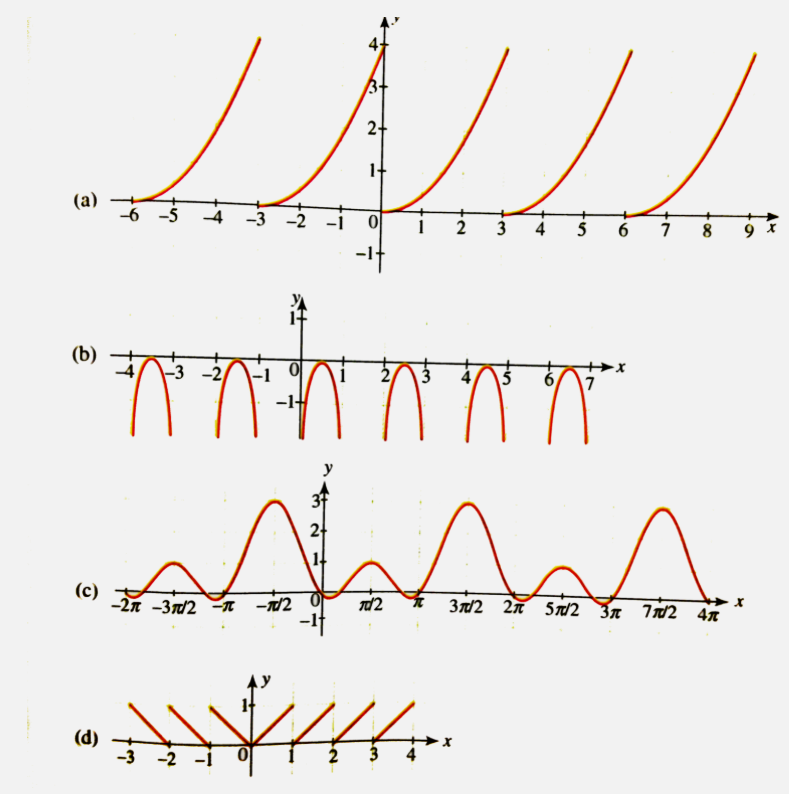 02:36View Solution
02:36View SolutionCheck whether the function has (have) y-symmetry or origin symmetry: f(x)=x2sinx.
05:12View SolutionLet f(x)=x2−2x−1∀x∈R.Letf:(−∞,a]→[b,∞), where a is the largest real number for which f(x) is bijective.
If f:R→R, then range of values of k for which equation f(|x|)=k has 4 distinct real roots isView SolutionDraw the graph of y=tan−1x−cot−1x.
View SolutionLet f:R→R be a continuous onto function satisfying f(x)+f(−x)=0∀x∈R. If f(−3)=2andf(5)=4∈[−5,5], then the minimum number of roots of the equation f(x)=0 is
02:15View SolutionLet f be a function satisfying f(x+y)=f(x) *f(y) for all x,y, in R. If f (1) =3 then sum_(r=1)^(n) f (r) is equal to
View SolutionLet f:R rarr R be a continuous onto function satisfying f(x)+f(-x)=0AA x in R, If f(-3)=2 and f(5)=4 in [-5,5], then the equation f(x)=0 has
06:23View SolutionLet f:R→R be a continuous onto function satisfying f(x)+f(−x)=0,∀x∈R . If f(−3)=2andf(5)=4 in [-5, 5], then what is the minimum number of roots of the equation f(x) = 0?
04:52View SolutionLet a function f:R→R, where R is the set of real nos. satisfying the equation f(x+y)=f(x)+f(y)∀x,y if f(x) is continuous at x = 0, then
05:12View SolutionLet f(x) be a continuous & differentiable function on R satisfying f(−x)=f(x)&f(3+x)=f(3−x)∀x∈R. If f'(1)=−5 then f'(7) =
02:12View SolutionLet f be a continuous function satisfying f(x) f(y)=f(x)+f(y)+f(xy)-2 for all x,y ∈ R and f(2)=5 then limx→4f(x) is
04:14View SolutionLet f:R→R be a continuous onto function satisfying f(x)+f(−x)=0∀x∈R. If f(−3)=2 and f(5)=4 in [−5,5], then the minimum number of roots of the equation f(x)=0 is
03:08View SolutionLet f:R→R be a continuous onto function satisfying f(x)+f(−x)=0,∀x∈R . If f(−3)=2andf(5)=4 in [-5, 5], then what is the minimum number of roots of the equation f(x) = 0?
02:32View SolutionLet a function f: R to R , where R is the set of real nos. satisfying the equation f(x+y) = f(x) + f(y) AA x, y if f(x) is continuous at x = 0, then
04:42View Solutionएक सतत् एवं आच्छादक फलन f:R→R इस प्रकार से है कि f(x)+f(−x)=0,∀x∈R. यदि f(-3)=2 तथा f(5)=4 तो अन्तराल [-5,5], में f(x)=0 का-
03:32View Solution

