Consider a triangle with vertices A(1,2),B(3,1), and C(−3,0). Find the equation of altitude through vertex A the equation of median through vertex A the equation of internal angle bisector of ∠ASolution in Bengali
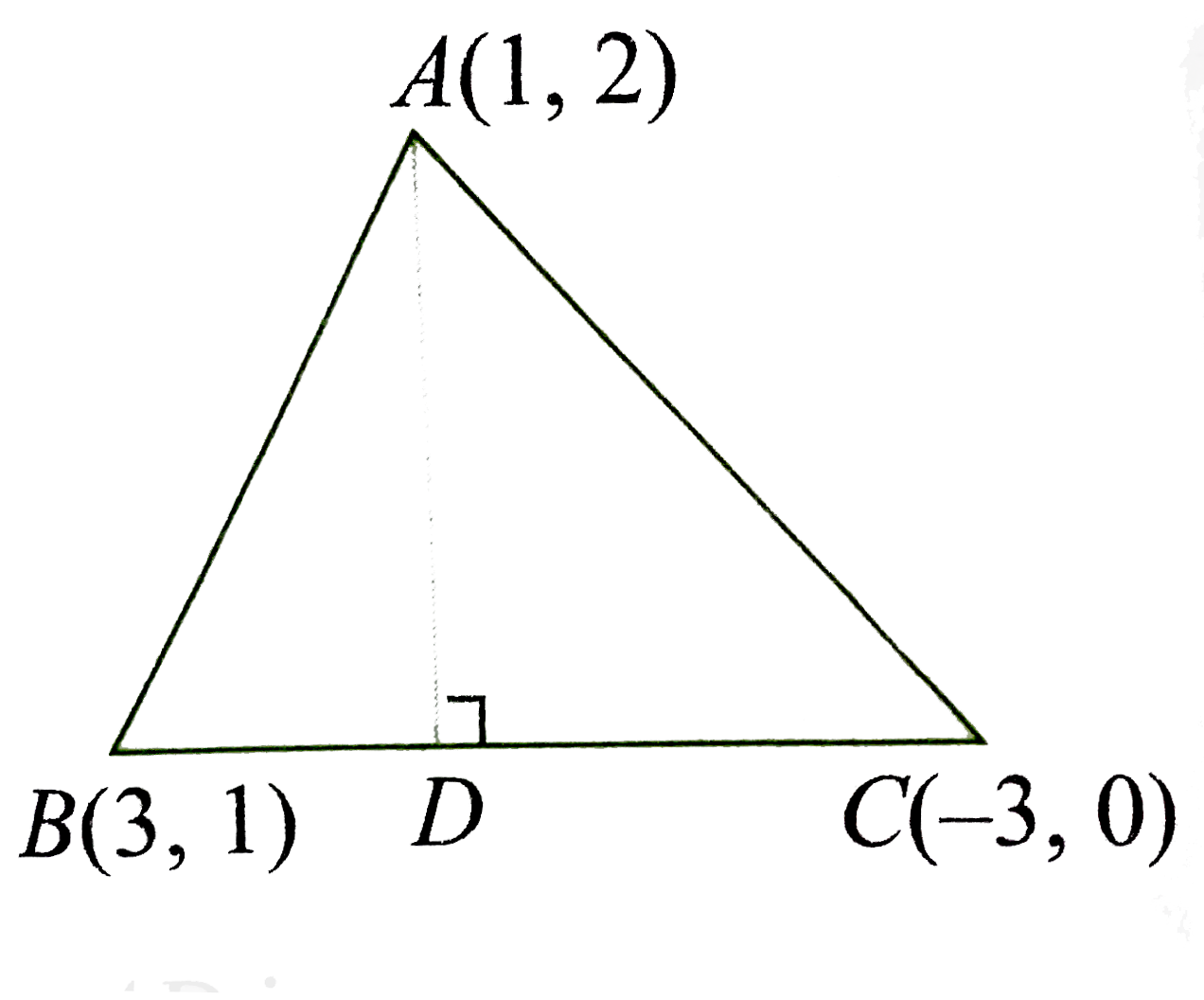
Let the altitude through A be AD.
Now, AD ⊥ BC
Slope of BC =1−03−(−3)=16
therefore, the equation of altitude AD is
y-2 = -6(x-1)
or 6x + y - 8 = 0

(ii) The median through A passes through the midpoint of BC.
Now, the midpoint of BC is E(3-3)/2, (1+0)/2) or E(0,1/2).
Therefore, the equation of
median AE is y−2=2−(1/2)1−0(x−1)
or 3x-2y+1 = 0
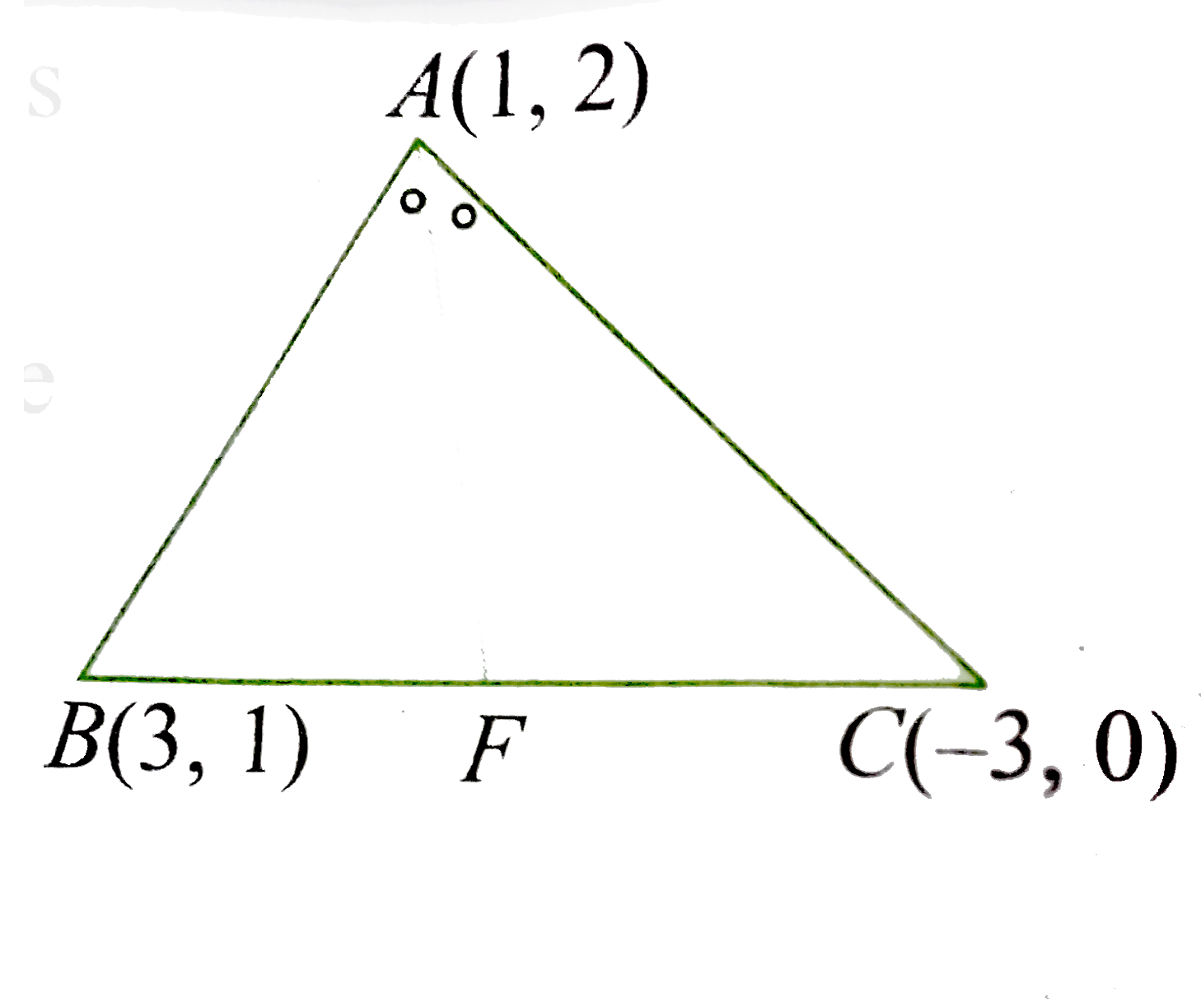
Let the internal bisector of A meets the side BC in F.
Now, the point F divides BC in the ratio
BFCF=ABAC=√5√20=12
Therefore, the coordinates of F are
(2(3)+1(−3)2+1,2(1)+1(0)2+1)=(1,23)
Therefore, the slope of AF is ∞.
Hence, the equation of AF is x-1 = 0.
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXAMPLE12videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - CONCEPT APPLICATION EXERCISE 2.123videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )1videos
THEORY OF EQUATIONS
Similar Questions
Show that the lines 4x+y−9=0,x−2y+3=0,5x−y−6=0 make equal intercepts on any line of slope 2.
06:08View SolutionFind the equation of the right bisector of the line segment joining the points (3,4) and (-1,2).
10:07View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
07:59View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
10:07View SolutionIn the triangle ABC with vertices A (2, 3), B (4, –1) and C (1, 2), find the equation and length of altitude from the vertex A.
View SolutionConsider a triangle with vertices A(1,2),B(3,1), and C(−3,0). Find the equation of altitude through vertex A. the equation of median through vertex A. the equation of internal angle bisector of ∠A.
02:22View SolutionLet ABC be a triangle such that the coordinates of the vertex A are (3,1). Equation of the median through B is 2x+y-3=0 and equation of the angular bisector of C is 7x-4y-1=0. Find the slope of line BC.
03:00View SolutionConsider a triangle with vertices A(1;2) ;B(3;1) and C(-3;0) Find (i) equation of altitude through vertex A (ii) the equation of median through vertex A (iii) the equation of internal angle bisector of ∠A
02:31View SolutionIn triangle ABC if y=x+1 & y=2x+3 are altitude through A and angle bisector of B respectively. If vertex C≡(3,4) then equation of median through C is
06:47View SolutionFind the equation of the median through vertex A of ΔABC if
A=(1,2),B=(−3,4),C=(−1,6)03:06View SolutionThe vertices of a triangle are (2,0) (0,2) (4,6) then the equation of the median through the vertex (2,0) is
10:32View SolutionThe vertices of a triangle are (2,0)(0,2)(4,6) then the equation of the median through the vertex (2,0) is
05:29View SolutionIn ΔABC,ifA=(1,2),B=(3,4)andAC=3,BC=4, then the equation of altitude through the vertex 'C' is
05:25View SolutionConsider a triangle with vertices A(1,2),B(3,1) and C(−3,0). Find the equation of altitude through vertex A.
05:55View SolutionConsider a triangle with vertices A(1,2),B(3,1), and C(−3,0). Find the equation of altitude through vertex A. the equation of median through vertex A. the equation of internal angle bisector of ∠A.
02:05View SolutionIn the triangle ABC with vertices A(2,3),B(4,1)andC(1,2), find the equation and length of altitude from the vertex A.
03:54View Solution
