The equation of straight line passing through (-2,-7) and having an intercept of length 3 between the straight lines : 4x + 3y = 12 , 4x + 3y = 3 are :
(A) 7x + 24y + 182 = 0
(B) 7x + 24y + 18 = 0
(C) x + 2 = 0
(D) x - 2 = 0Solution in Bengali
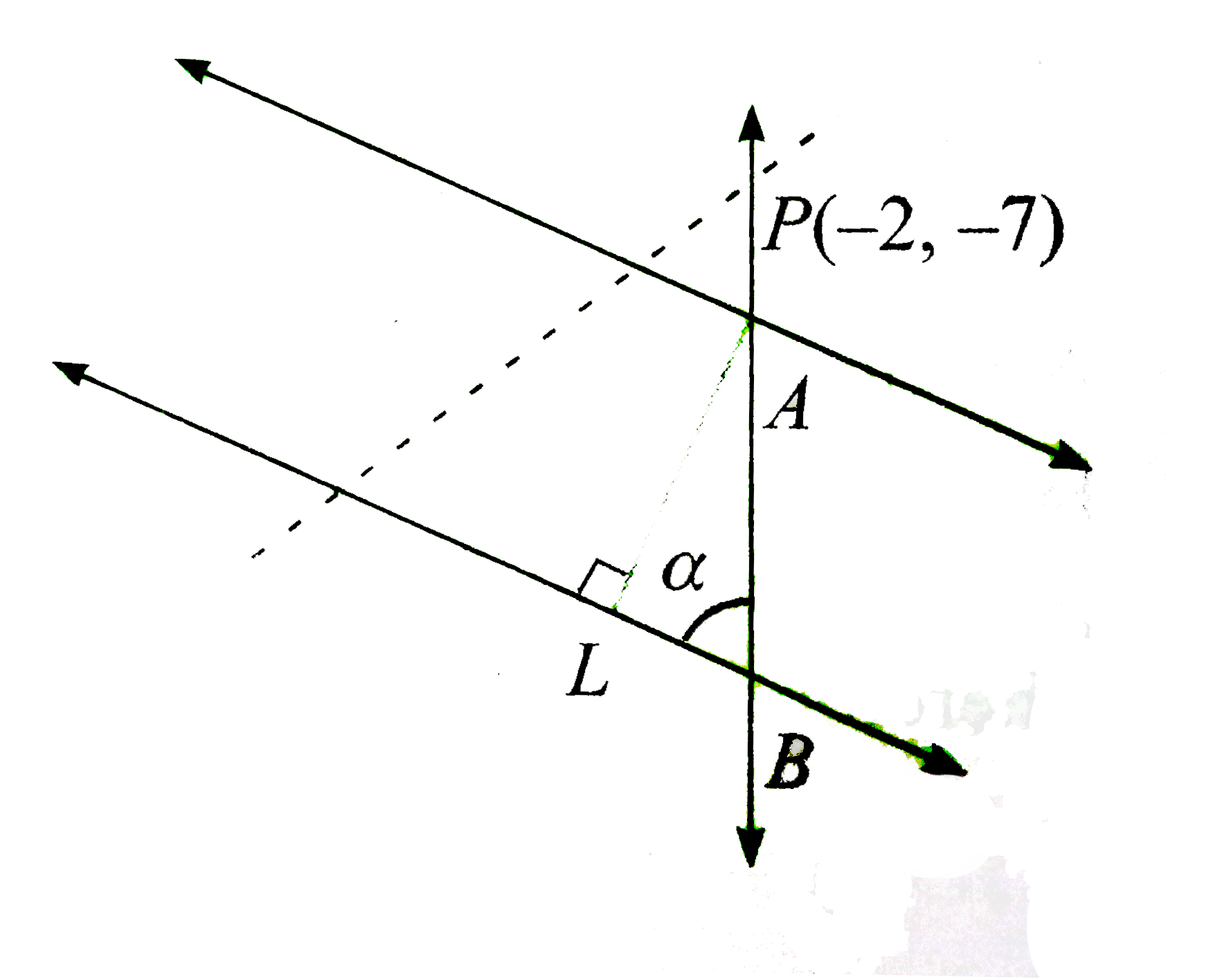
Given lines are
4x+3y−12=0 (1)
and 4x+3y−3=0 (2)
Given AB=3.
The distance between parallel lines (1) and (2),
AL=|−12+3|√42+32=95
FromΔALB, we get
LB2=AB2−AL2
=32−8125=9×1625
∴LB=125
tanα=ALLB=34
Also, tanθ=Slope of line(1)=−43
Let the slope of PB be m. Now,
tanα=34=∣∣∣m−(−4/3)1+m(−4/3)∣∣∣=∣∣∣3m+43−4m∣∣∣
or m=∞orm=−724
Therefore, the equation of the line is
x+2=0 and y+7=−724(x+2)
or x+2=0 and 7x+24y+182=0
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXAMPLE12videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - CONCEPT APPLICATION EXERCISE 2.123videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )1videos
THEORY OF EQUATIONS
Similar Questions
Show that the lines 4x+y−9=0,x−2y+3=0,5x−y−6=0 make equal intercepts on any line of slope 2.
09:48View SolutionFind the equation of the right bisector of the line segment joining the points (3,4) and (-1,2).
03:16View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
01:39View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
View SolutionThe equation of straight line passing through (-2,-7) and having an intercept of length 3 between the straight lines: 4x+3y=12,4x+3y=3 are :(A)7x+24y+182=0 (B) 7x+24y+18=0(C)x+2=0(D)x-2=0
02:33View SolutionA point equidistant from the line 4x+3y+10=0,5x-12y+26=0 and 7x+24y-50=0 is
03:56View SolutionShow that the origin is equidistant from the lines 4x+3y+10=0;5x-12y+26=0 and 7x+24y=50
03:22View SolutionThe point equidistant to the lines 4x+3y+10=0,5x−12y+26=0,7x+24y−50=10 is
05:29View SolutionA point equidistant from the lines 4x+3y+10=0,5x−12y+26=0and7x+24y−50=0 is :
11:36View Solutionरेखाओं 4x + 3y+10 = 0, 5x - 12y+26 = 0 व 7x+24y-50 = 0 से समदूरस्थ बिन्दु है
02:59View SolutionThe straight lines 4x−3y−5=0,x−2y=0,7x+y−40=0 and x+3y+10=0 from
02:59View SolutionA point equidistant from the line 4x+3y+10=0,5x−12y+26=0 and 7x+24y−50=0is
03:04View SolutionThe equation of straight line passing through (-2,-7) and having an intercept of length 3 between the straight lines : 4x + 3y = 12 , 4x + 3y = 3 are : (A) 7x + 24y + 182 = 0 (B) 7x + 24y + 18 = 0 (C) x + 2 = 0 (D) x - 2 = 0
05:32View SolutionThe equation of straight line passing through (-2,-7) and having an intercept of length 3 between the straight lines : 4x + 3y = 12 , 4x + 3y = 3 are : (A) 7x + 24y + 182 = 0 (B) 7x + 24y + 18 = 0 (C) x + 2 = 0 (D) x - 2 = 0
03:51View SolutionThe equation of straight line passing through (-2,-7) and having an intercept of length 3 between the straight lines : 4x + 3y = 12 , 4x + 3y = 3 are : (A) 7x + 24y + 182 = 0 (B) 7x + 24y + 18 = 0 (C) x + 2 = 0 (D) x - 2 = 0
04:09View Solution

