Question

Let the sides of a parallelogram be U=a, U=b,V=a' and V=b', where U=lx+my+n, V=l'x+m'y+n'. Show that the equation of the diagonal through the point of intersection of
$U=a,V=a\text{'}\text{and}U=b,V=b\text{'}\text{is given by}\left|\begin{array}{ccc}U& V& 1\\ a& a\text{'}& 1\\ b& b\text{'}& 1\end{array}\right|=0.$

Explanation

Parallelogram is formed by lines U=a, U=b, V=a' and V=b', where U=lx+my+n, V=l'x+m'y+n'

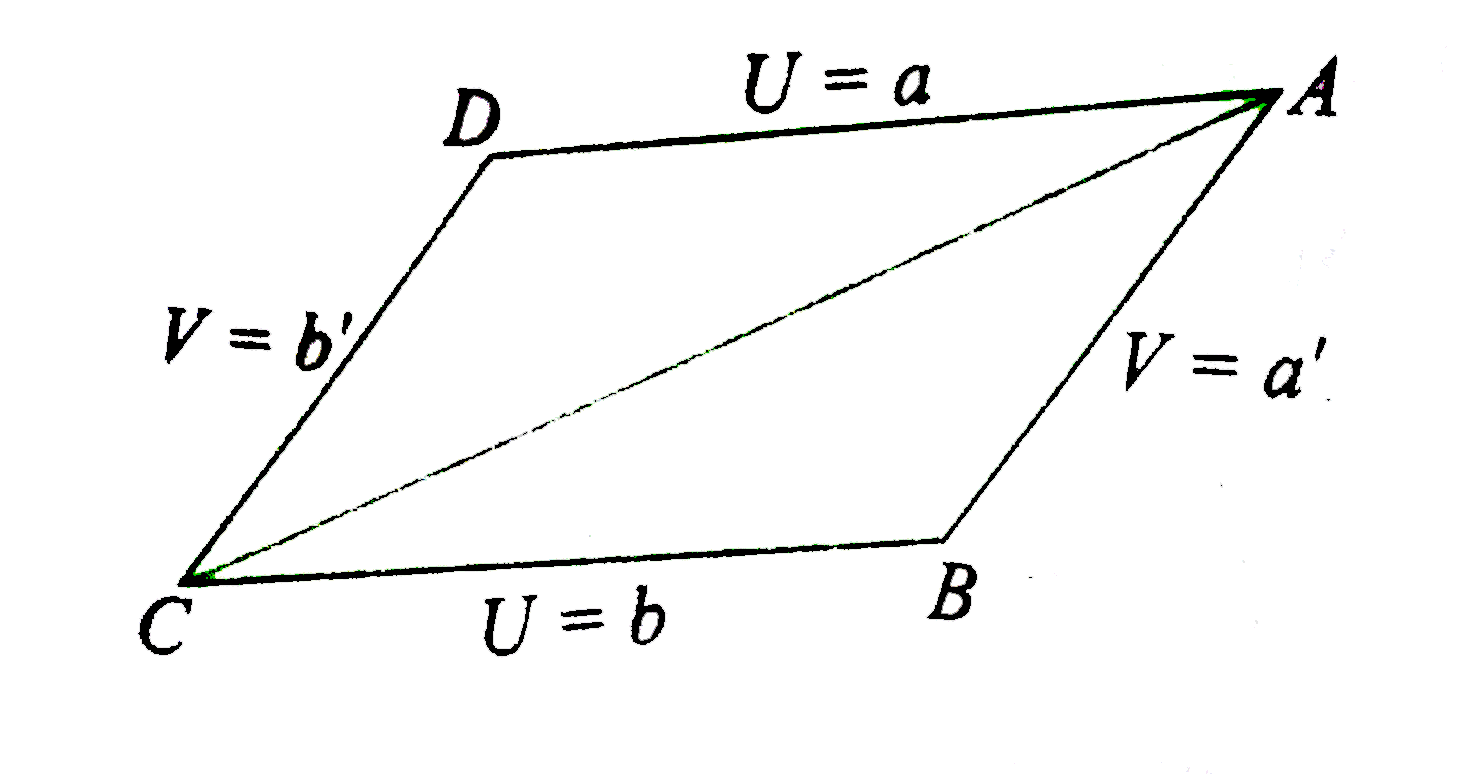
Let the required diagonal be AC.
Since one end A of the diagonal AC passes through the point of intersection of lines U-a=0 and V-a'=0, its equation is given by
`(U-a)+lambda(V-a')=0 " " (1)`
But the other end C of the same diagonal passes through the point of intersection of lines U-b=0 and V-b' = 0.
So, its equation is given by
`(U-b)+mu(V-b') = 0 " " (2)`
Equation (1) and (2) represents the same straight lines.
`therefore 1=(lambda)/(mu) = (a+lambdaa')/(b+mub')`
`rArr lambda=mu = -((a-b)/(a'-b'))`
Putting the value of `lambda` in (1), we get
`(U-a)-((a-b)/(a'-b'))(V-a')=0`
`" or " |{:(U,V,1),(a,a',1),(b,b',1):}| =0`
This is the required equation of diagonal.