In a plane there are two families of lines y=x+r,y=−x+r, where r∈{0,1,2,3,4}. Find the number of squares of diagonals of length 2 formed by the lines Solution in Bengali
The correct Answer is:9
Each family has parallel lines having the distance between them as 1/√2 unit. Both the families are perpendicular to each other. So, to form a square of diagonal 2 units, lines of alternate pair are to be chosen.
Both the families have three such pairs. So, the number of squares possible is 3×3=9.
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - ARCHIVES (JEE MAIN)
9 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - ARCHIVES (JEE ADVANCED)
3 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MATRIX MATCH TYPE)
8 Videos
- STRAIGHT LINEBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )
1 Videos
Similar Questions
The line L given by x5+yb=1 passes through the point (13,32).the line K is parallel to L and has the equation xc+y3=1 then the distance between L and K is
View SolutionThe locus of the orthocentre of the triangle formed by the lines (1+p)x−py+p(1+p)=0,(1+q)x−qy+q(1+q)=0 and y = 0, where p≠⋅q, is (A) a hyperbola (B) a parabola (C) an ellipse (D) a straight line
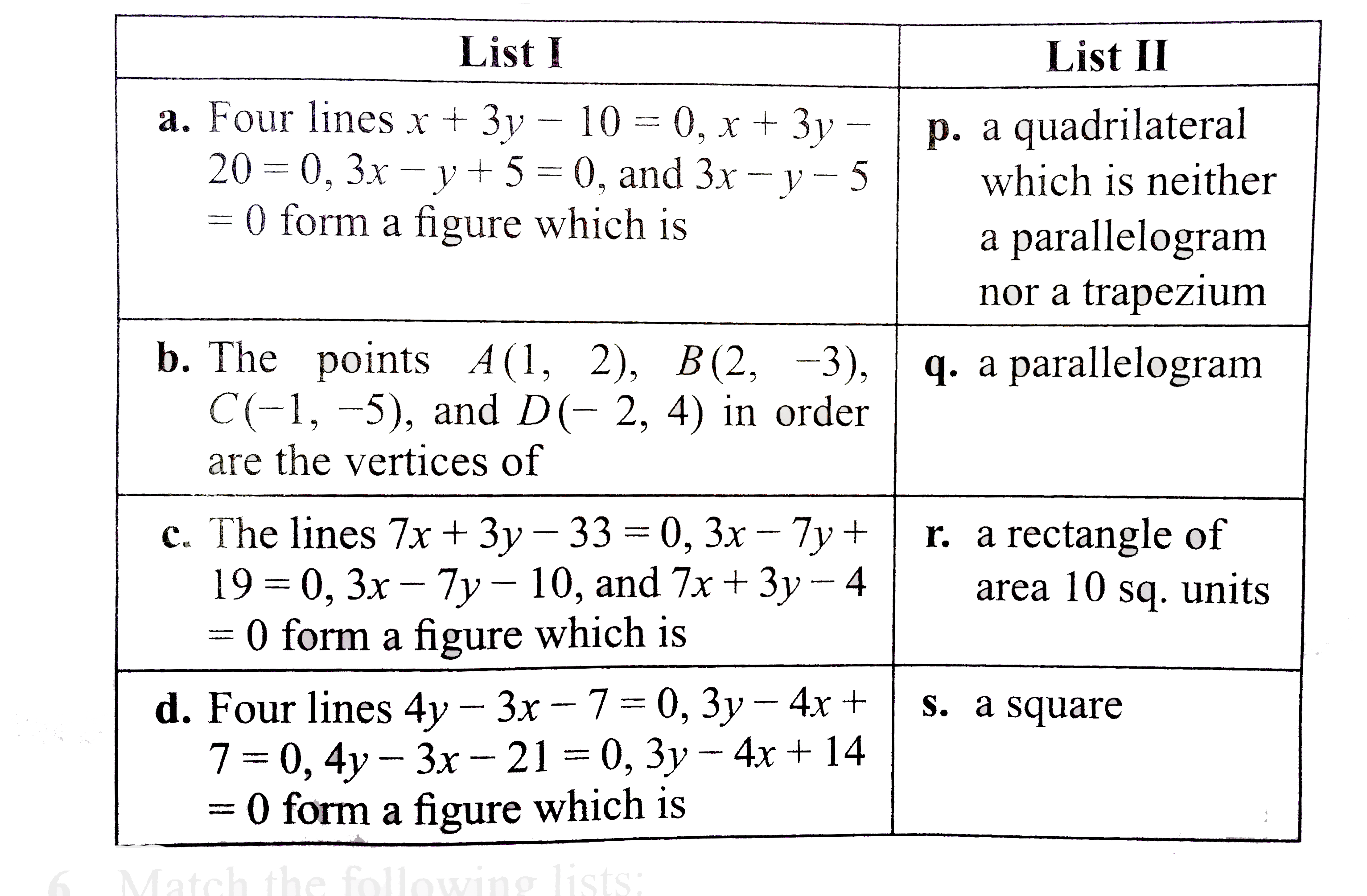
View SolutionMatch the following lists:
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
View SolutionIn a plane there are two families of lines y=x+r,y=-x+r, where r in{0,1,2,3,4}. The number of squares of diagonals of length 2 formed by the lines is:
View SolutionFind the equations of the diagonals of the square formed by the lines x=0,y=0,x=1andy=1.
View SolutionIf x2+y2=R2(whereR>0)andk=y''(1+y'2)3 then find k in terms of R alone.
View SolutionConsider the fourteen lines in the plane given by y=x+r, y=-x+r, where r∈{0,1,2,3,4,5,6}. The number of squares formed by these lines whose diagonals are of length 2, is
View SolutionIf common tangents of x2+y2=r2 and x216+y29=1 forms a square, then the length of diagonal of the square is
View SolutionConsider the fourteen lines in the plane given by y=x+r,y=−x+r, where r∈{0,1,2,3,4,5,6). The number of squares formed by these lines, whose sides are of length √2, is
View SolutionIf the line x+2y=3 cuts a chord of length r unit with the circle x2+y2=r2 then find r2.
View SolutionThe equation of the plane containing the straight line x2=y3=z4 and perpendicular to the plane containing the straight lines x3=y4=z2andx4=y2=z3 is
px+qy+r=0, then p+q+r is
View SolutionIn a plane there are two families of lines y = x+c, y=-x+c where ce{0, 1, 2, 3, 4} the number of squares of diagonals of length 2 units formed by the lines is
View SolutionFind the equations of the diagonals of the square formed by the lines x=0,y=0,x=1 and y=1.
View SolutionIn a plane there are two families of lines y=x+r,y=−x+r, where r∈{0,1,2,3,4}. The number of squares of diagonals of length 2 formed by the lines is:
View Solution
