Find the coordinates of the point which divides the line segments joining the points (6,3) and (−4,5) in the ratio 3:2 (i) internally and (ii) externally.Solution in Bengali
(i) Given points are A (6,3) and B(-4,5). Let poitn P(x,y) divide AB internally in the ratio 3:2

∴(x,y)≡(3(−4)+2(6)3+2,3(5)+2(3)3+2)≡(0,215)
(ii) P(x,y) divides AB externally in the ratio 3:2.
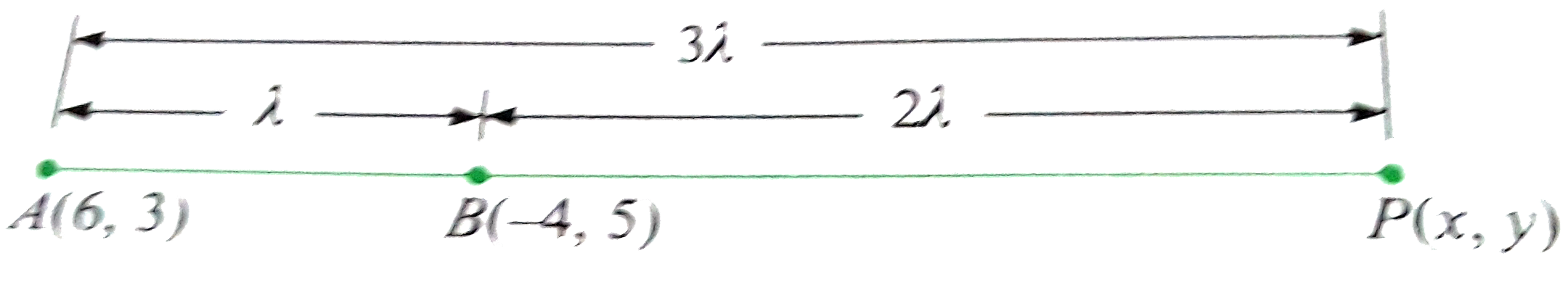
∴(x,y)≡(3(−4)−2(6)3−2,3(5)−2(3)3−2)≡(−24,9)
Alternatively, ABBP=(12) (From the figure)
∴(−4,5)≡(3(6)+1(x)2+1,2(3)+1(y)2+1)≡(12+x3,6+y3)
∴(x,y)≡(−24,9)
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Illustration1.24
1 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Illustration1.25
1 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Illustration1.22
1 Videos
- COORDINATE SYSTEMBOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGE PUBLICATIONCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
Given that A(1,1) and B(2,−3) are two points and D is a point on AB produced such that AD=3AB. Find the coordinates of D.
View SolutionDetermine the ratio in which the line 3x+y−9=0 divides the segment joining the points (1,3) and (2,7).
View SolutionIf A(3√2,√2), B(−3√2,√2),C(−3√2,−√2) and D(3cosθ,2sinθ) are four points . If the area of the quadrilateral ABCD is maximum where θ∈(3π2,2π) then (a) maximum area is 10 sq units (b) θ=7π4 (c) θ=2π−sin−13√85 (d) maximum area is 12 sq units
View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
View SolutionFind the coordinates of the point which divides the line segment joining the points (1,2,3)and (3,4,5)in the ratio 2:3
(i) internally, and (ii) externally.
View SolutionFind the coordinates of the point which divides the line segments joining the points (6,3) and (−4,5) in the ratio 3:2 (i) internally and (ii) externally.
View SolutionFind the coordinates of the point which divides the line segment joining the points (1,-2,3) and (3,4,-5) in the ratio 2:3(1) internally, and (2) externally
View Solution(i) Find the coordinates of the point which divides the line segment joining the points (6;3) and (-4;5) in the ratio 3:2 internally
View SolutionFind the coordinates of a point which divides the line segments joining the points (1;−2;3)&(3;4;−5) in the ratio 2:3 internally and externally
View SolutionFind the coordinates of the points which divides the line segment joining the points (6, 3) and (−4, 5) in the ratio 3 :2 internally.
View SolutionFind the coordinates of the point which divides the line segment joining the points (1,-2,3) and (3,4,-5) in the ratio 2:3 (i) internally and (ii) externally
View SolutionFind the coordinates of the point which divides the line segments joining the points (6,3) and (−4,5) in the ratio 3:2 (i) internally and (ii) externally.
View SolutionFind the coordinates of the point which divides the line segments joining the points (6,3) and (−4,5) in the ratio 3:2 (i) internally and (ii) externally.
View SolutionFind the coordinate of the point which divides the line segment joining the points (3,−2,5) and (3,4,2) in the ratio 2:1
internally
View SolutionFind the coordinate of the point which divides the line segment joining the points (3,−2,5) and (3,4,2) in the ratio 2:1 externally
View Solution
