Question

Solve $\tan x>\cot x,$
where $x\in [0,2\pi ]\stackrel{.}{}$

Explanation

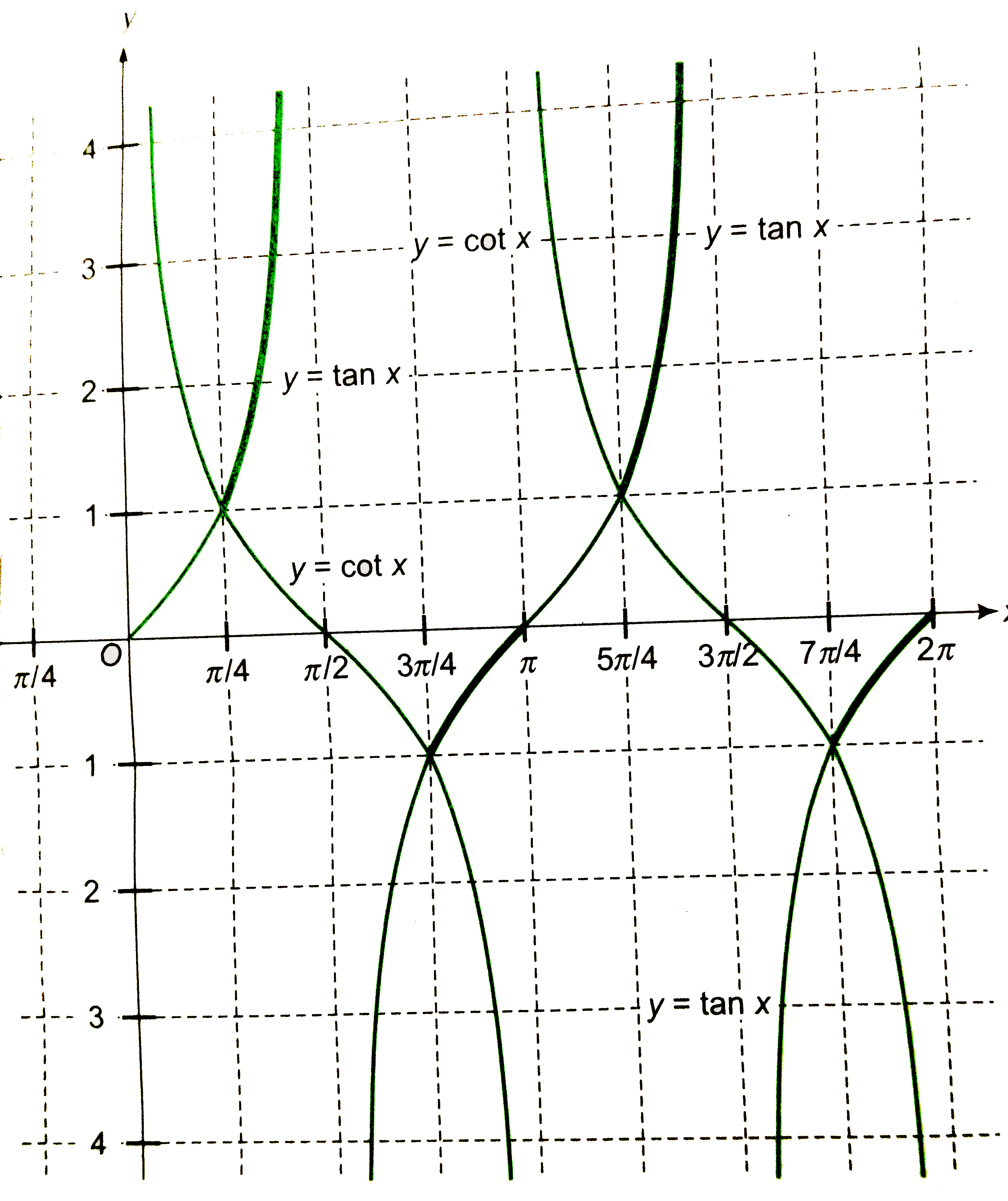
We find the `tanxgecotx`.
Therefore, the value of tan x is more than the value of cot x.
That is, the values of x for which graph of `y=tanx` is above the graph of `y=cot x`.
From the graph, it is clear that
`x in (pi//4,pi//2)uu(3pi//4,pi)uu(5pi//4,3pi//2)uu(7pi//4,2pi)`.