The number of five-digit numbers which are divisible by 3 that can be formed by using the digits 1,2,3,4,5,6,7,8 and 9, when repetition of digits is allowed, is (a)310 (b)4.38 (c)5.38 (d)7.38Solution in Bengali
310
4.38
5.38
7.38
The correct Answer is:A
(a) 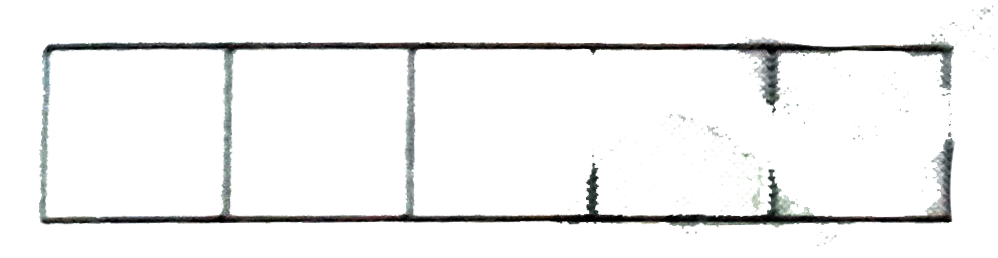
1st blank can be filled in 9 ways
2nd blank can be filled in 9 ways (repetition is allowed)
3rd blank can be filled in 9 ways
4th blank can be filled in 9 ways
Now, we have to fill the 5th blank carefully such that the number is divisible by 3.
Add the 4 numbers in the first 4 blanks.
If their sum is in the form 3n, then fill the last blank by 3, 6 or 9 so that the sum of all digits is divisible by 3.
If their sum is in the form 3n+1, than fill the last bank by 2, 5 or 8.
If their sum is in the form 3n+2, than fill the last blank by 1, 4 or 7.
Therefore, in any case, the last blank can be filled in 3 ways only.
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - PERMUTATION AND COMBINATIONEXERCISE - Multiple Correct Answer2videos
PERMUTATION AND COMBINATION
- BOOK - CENGAGE PUBLICATIONCHAPTER - PERMUTATION AND COMBINATIONEXERCISE - Comprehension8videos
PERMUTATION AND COMBINATION
- BOOK - CENGAGE PUBLICATIONCHAPTER - PARABOLAEXERCISE - Matching Column Type1videos
PARABOLA
- BOOK - CENGAGE PUBLICATIONCHAPTER - PRINCIPLE OF MATHEMATICAL INDUCTIONEXERCISE - Sovled Examples22videos
PRINCIPLE OF MATHEMATICAL INDUCTION
Similar Questions
A contest consists of ranking 10 songs of which 6 are Indian classic and 4 are westorn songs. Number of ways of ranking so that, There are exactly 3 indian classic songs in top 5 is (5!)3.
12:37View SolutionGiven are six 0's, five 1's and four 2's . Consider all possible permutations of all these numbers. [A permutations can have its leading digit 0].
How many permutations have the first 0 preceding the first 1 ?
(a) 15C4×10C5 (b) 15C5×10C4 (c) 15C6×10C5 (d) 15C5×10C502:20View SolutionAB is a chord of y2=4x such that normals at A and B intersect at C(9,6) and the tengent at A and B at point T, find (CT)2/13
01:41View SolutionProve the following by using the principle of mathematical induction for all n∈N:13+23+33+...........+n3=(n(n+1)2)2
03:05View SolutionHow many five digits numbers divisible by 3 can be formed using the digits 0,1,2,3,4,7 and 8 if each digit is to be used atmost.once.
07:04View SolutionWrite all possible 3-digit numbers using the digits 6, 0, 4, when repetition of digits is not allowed repetition of digits is allowed
01:35View SolutionWrite all the possible 4-digit even numbers using the digits 5, 8, 9 and 4 when the repetition is not allowed.
04:32View SolutionThe number of five-digit numbers which are divisible by 3 that can be formed by using the digits 1,2,3,4,5,6,7,8 and 9, when repetition of digits is allowed, is
04:07View SolutionThe number of four-digit numbers that can be formed by using the digits 1,2,3,4,5,6,7,8 and 9 such that the least digit used is 4, when repetition of digits is allowed is
02:57View SolutionA 2 digit number is formed using the digits 2, 3, 5, 8 and 9 without repetition. What is the probability that the digits used are 3 and 9?
04:19View SolutionThe number of 5 digited numbers that can be formed using the digits the digits 0,1,2,3,4,5 that are divisible by 5 when repetition is allowed is
04:03View SolutionFind the number of 4 digit numbers divisible by 5 that can be formed using the digits 1, 2, 3, 4, 5 when repetition of digits is allowed.
06:03View SolutionThe number of four digit numbers that can be formed using the digits 1,2,3,4,5,6,7,8,9 which are divisible by 3, when repetition of digits is allowed any number of times, is
02:50View SolutionThe number of five-digit numbers which are divisible by 3 that can be formed by using the digits 1,2,3,4,5,6,7,8 and 9, when repetition of digits is allowed, is
02:12View SolutionThe number of four-digit numbers that can be formed by using the digits 1,2,3,4,5,6,7,8 and 9 such that the least digit used is 4, when repetition of digits is allowed is
01:47View SolutionGiven the digits 1,2,3 and 8 If repetition of digits is not allowed, find the number of numbers which can be formed from the given digits
03:53View Solution

