Four letters, two 'a' and two 'b' are filled into 16 cells of a matrix as given. It is required that each cell contains atmost one letter and each row or column cannot contain same letters. Then the number of ways the matrix can be filled is
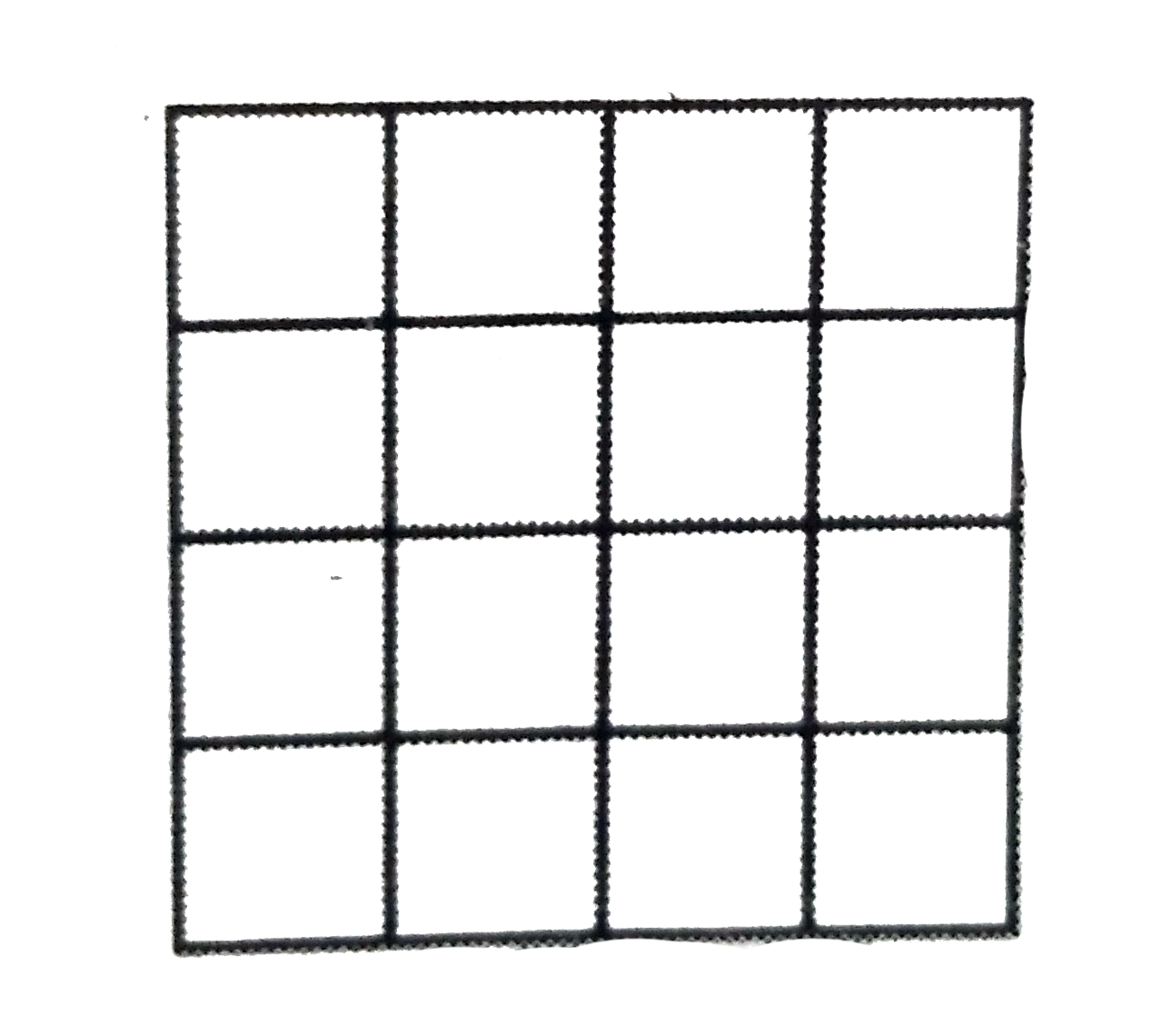
(A) 3600
(B) 5200
(C) 3960
(D) 4120Solution in Bengali
3600
5200
3960
4120
The correct Answer is:C
(c) Selecting two rows for two 'a' in 4C2 ways. Now first 'a' can be put in any row in 4 ways, Second 'a' can be put in other row in 3 ways as no two a' are in same column.
Thus two 'a' can be filled in
4C2.4×3=72 ways.
Similarly two 'b' can be filled in 72 ways.
Thus two 'a' and two 'b' can be filled in 722 ways.
Now, we have no exclude the cases when
(i) both 'a' and 'b' are in same cell i.e., 72 ways (as after fixing 'a' in 72 ways, 'b' can be placed in this position)
(ii) one 'a' and 'b' are in same row or coloumn i.e., 72×16 ways
Required number of ways =722−72−72×16=3960
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - PERMUTATION AND COMBINATIONEXERCISE - Multiple Correct Answer2videos
PERMUTATION AND COMBINATION
- BOOK - CENGAGE PUBLICATIONCHAPTER - PERMUTATION AND COMBINATIONEXERCISE - Comprehension8videos
PERMUTATION AND COMBINATION
- BOOK - CENGAGE PUBLICATIONCHAPTER - PARABOLAEXERCISE - Matching Column Type1videos
PARABOLA
- BOOK - CENGAGE PUBLICATIONCHAPTER - PRINCIPLE OF MATHEMATICAL INDUCTIONEXERCISE - Sovled Examples22videos
PRINCIPLE OF MATHEMATICAL INDUCTION
Similar Questions
A contest consists of ranking 10 songs of which 6 are Indian classic and 4 are westorn songs. Number of ways of ranking so that, There are exactly 3 indian classic songs in top 5 is (5!)3.
03:47View SolutionGiven are six 0's, five 1's and four 2's . Consider all possible permutations of all these numbers. [A permutations can have its leading digit 0].
How many permutations have the first 0 preceding the first 1 ?
(a) 15C4×10C5 (b) 15C5×10C4 (c) 15C6×10C5 (d) 15C5×10C502:11View SolutionAB is a chord of y2=4x such that normals at A and B intersect at C(9,6) and the tengent at A and B at point T, find (CT)2/13
01:31View SolutionProve the following by using the principle of mathematical induction for all n∈N:13+23+33+...........+n3=(n(n+1)2)2
02:45View SolutionIdentify the cell organelle 'A' :-
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/ALN_BIO_C10_E08_018_Q01.png" width="80%"gt02:17View SolutionDetermine the point of symmetry of a regular hexagon.
05:41View SolutionDtermine the images of the following figure about the given line :
02:10View SolutionMatch the following Column A to Column B
 02:17View Solution
02:17View SolutionMatch the following Column A to Column B
 02:10View Solution
02:10View SolutionMatch the following Column A to Column B
 04:23View Solution
04:23View SolutionThe inequation represented by the graph given below is :
01:42View SolutionThe inequation that best describes the graph given below is _________
03:12View SolutionThe inequation that best describes the following graph is _________
04:00View SolutionWhich inequations represent the shaded region in the given figure
05:46View SolutionIn the chemical reactions,
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/JM_10_CHE_P1_E01_009_S01.png" width="80%"gt
the compounds 'A' and 'B' respectively are06:52View SolutionMatch the followings (column I with column II)
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/NCERT_BIO_XI_C03_E01_011_Q01.png" width="80%"gt06:00View Solution

