A triangle ABC vertices A(5,1),B(-1,-7) and C(1,4). Respectively. L be the line mirror passing through C and parallel to AB. A light ray emanating from point A goes along the direction of the internal bisector of angle A, which meets the mirror and BC at E and D, respectively. then the sum of the areas of ΔACEandΔABC is Solution in Bengali
17 sq. units
18 sq. units
503 sq. units
20 sq. units
The correct Answer is:C
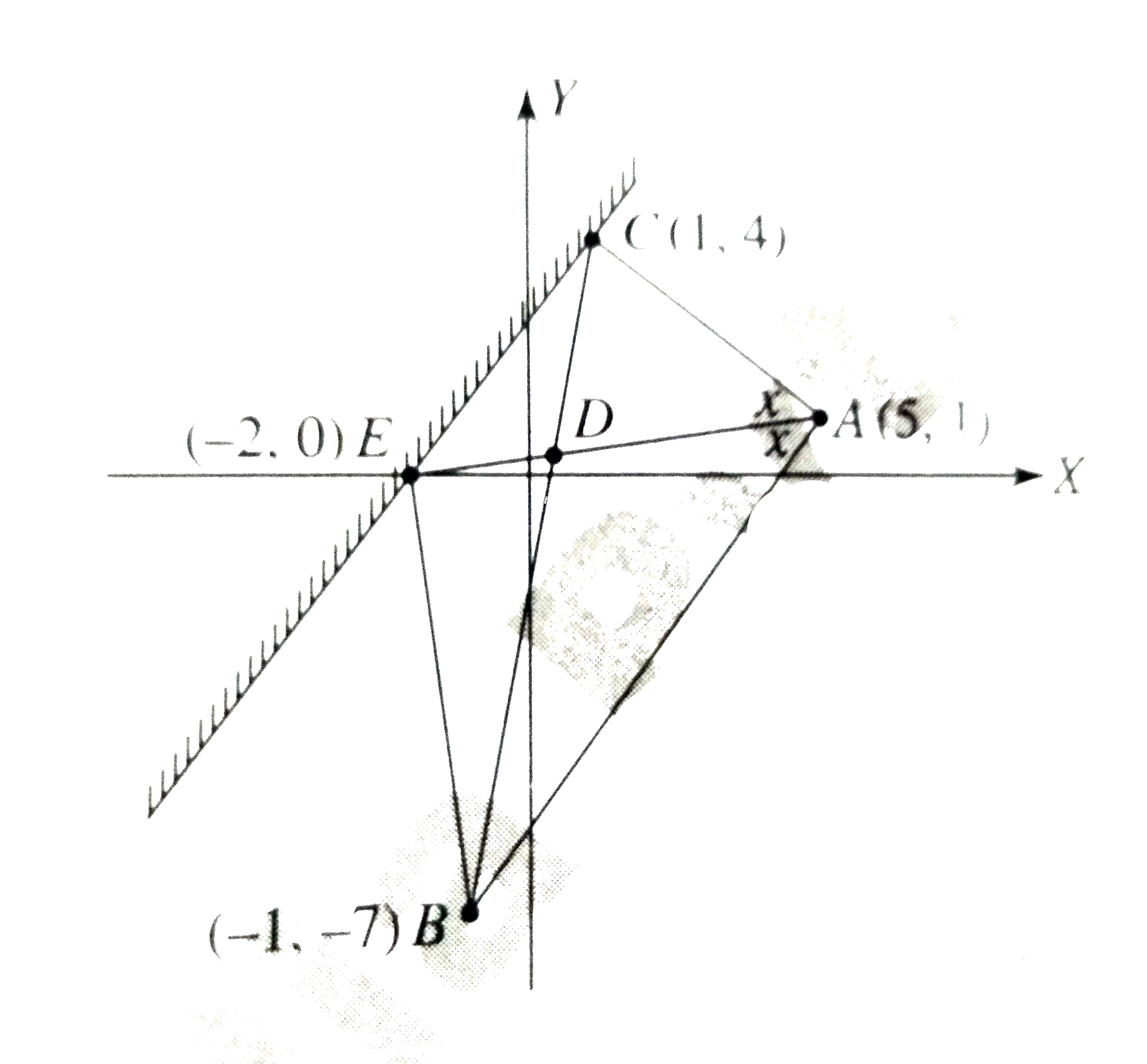
ADDE=BDDC=ABAC
∴ Point D(13,13) and E(−2,0).
Area of ΔADC+ Area of ΔBDE
=253+253
=503 sq. units
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSTEMEXERCISE - Comprehension Type4videos
COORDINATE SYSTEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type2videos
COORDINATE SYSTEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - All Questions238videos
CONTINUITY AND DIFFERENTIABILITY
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - JEE Main6videos
COORDINATE SYSYEM
Similar Questions
A(x1,y1),B(x2,y2),C(x3,y3) are three vertices of a triangle ABC, lx+my+n=0 is an equation of line L. If L intersects the sides BC,CA and AB of a triangle ABC at P,Q,R respectively, then BPPC×CQQA×ARRB is equal to
01:13View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
06:37View SolutionFind x where f(x)=max{√x(2−x),2−x} is non-differentiable.
03:17View SolutionThe lines p(p2+1)x−y+q=0 and (p2+1)2x+(p2+1)y+2q=0 are perpendicular to a common line for
06:58View SolutionThe vertices of a triangle are A(−1,−7),B(5,1)andC(1,4). If the internal angle bisector of ∠B meets the side AC in D, then find the length AD.
05:39View SolutionThe vertices of a triangle are A(-1,-7),B(5,1) and C(1,4). The equation of the bisector of the angle ABC
04:12View SolutionIn a triangle ABC,angle bisector of angle B meets side AC at point D.Does the reflection of point A falls on line BC,if BD is taken as the mirror?
04:09View SolutionA triangle ABC has vertices A(5, 1), B (-1, -7) and C(1, 4) respectively. L be the line mirror passing through C and parallel to AB and a light ray eliminating from point A goes along the direction of internal bisector of the angle A, which meets the mirror and BC at E, D respectively. If sum of the areas of triangle ACE and triangle ABE is K sq units then(2K)/5-6 is
10:55View SolutionIn a triangle ABC, D and E are points on AB, AC respectively such that DE is parallel to BC. Suppose BE, CD intersect at O. If the areas of the triangles ADE and ODE are 3 and 1 respectively, find the area of the triangle ABC, with justification
06:52View SolutionΔABC में, AD∠BAC का समद्विभाजक है । जो BC से D पर मिलती है, तो सिद्ध करें कि ar(ΔABD)ar(ΔACD)=ABAC
02:20View Solutionएक ΔABC के ∠B और ∠Cके समद्विभाजक सम्मुख AC और AB को क्रमशः बिंदु D और E पर काटते है|यदि DE∣∣BC हो, तो सिद्ध करों की ΔABC एक समद्विबाहु त्रिभुज है|
06:56View SolutionA(0,0),B(4,2)andC(6,0) are the vertices of the triangle ABC . BD is an altitude of triangle ABC . A line passes through D and parallel with AB meets BC at E . Then the product of the area (in sq. units ) of ΔABCandΔBDE will be -
07:23View SolutionIn ΔABC,D,E,F are respectively the mid points of the sides AB, BC and AC. Find ratio of the area of ΔDEF and area of ΔABC.
05:32View SolutionΔABC లో DE // BC మరియు D, E లు వరుసగా AB, AC లపై బిందువులైన
03:56View SolutionA triangle ABC has vertices A(5,1),B(−1,−7)andC(1,4) respectively. L be the line mirror passing through C and parallel to AB and a light ray eliminating from point A goes along the direction of internal bisector of the angle A, which meets the mirror and BC at E, D respectively. If sum of the areas of △ACE and △ABE is K sq units then2K5−6 is
04:13View SolutionInternal bisector of an angle ∠A of triangle ABC meets side BC at D. A line drawn through D perpendicular to AD intersects the side AC at E and the side AB at F. If a, b, c represent the sides of DABC then
06:56View Solution

