Circles of radius 5 units intersects the circle ${(x - 1)}^{2} + {(x - 2)}^{2} = 9$ in a such a way that the length of the common chord is of maximum length. If the slope of common chord is $\frac{3}{4}$ , then find the centre of the circle.

Text Solution

Verified by Experts

The correct Answer is: $(\frac{17}{5}, \frac{- 6}{5})$ and $(\frac{- 7}{5}, \frac{26}{5})$

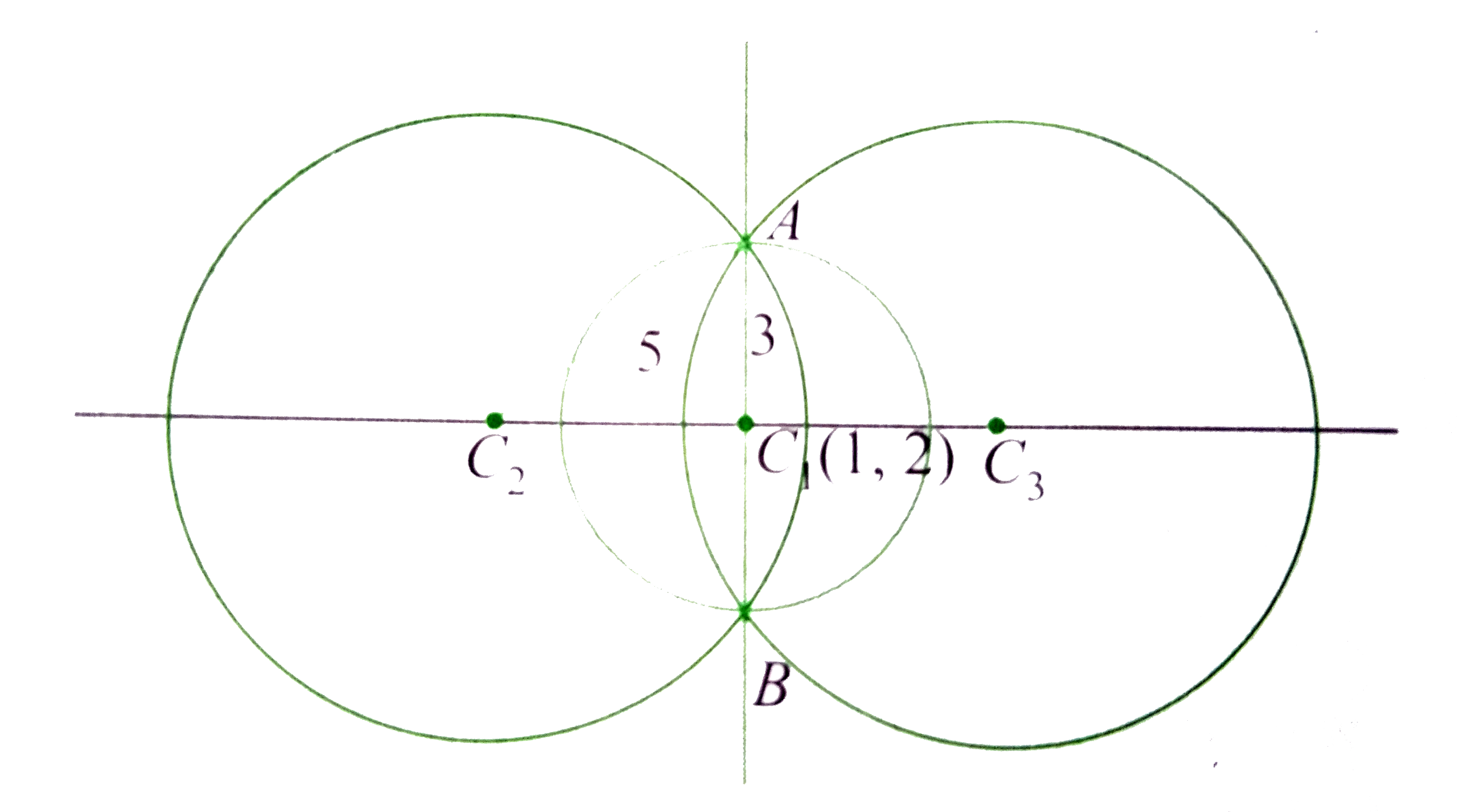
Clearly, common chord AB will be along the diameter of given circle.
In triangle $A C_{2} C_{2}, A C_{1} = 3, A C_{2} = 5$ .
$∴ C_{1} C_{2} = C_{1} C_{3} = 4$ .
Given that slope of AB is $\frac{3}{4}$ . $∴$ Slope of $C_{2} C_{3}, - \frac{4}{3} = tan θ$
Using parametric form of straight line, coordinates of $C_{2}$ and $C_{3}$ are given by
$(1 \pm 4 cos θ, 2 - + 4 sin θ) \equiv (1 \pm 4 \frac{3}{5}, 2 - + 4 \frac{4}{5})$
$\equiv (\frac{17}{5}, \frac{6}{5})$ and $(\frac{- 7}{5}, \frac{26}{5})$

|

Updated on:21/07/2023

Class 12 MATHS CIRCLE

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The circles having radii $r_{1} a n d r_{2}$ intersect orthogonally. The length of their common chord is $\frac{2 r_{1} r_{2}}{\sqrt{r 12 + r 12}}$ (b) $\frac{\sqrt{r 12 + r 12}}{2 r_{1} r_{2}}$ $\frac{r_{1} r_{2}}{\sqrt{r 12 + r 12}}$ (d) $\frac{\sqrt{r 12 + r 12}}{r_{1} r_{2}}$
A $\frac{2 r_{1} r_{2}}{\sqrt{r_{1}^{2} + r_{2}^{2}}}$
B $\frac{r_{1} r_{2}}{\sqrt{r_{1}^{2} + r_{2}^{2}}}$
C $\frac{2 r_{1}^{2} r_{2}}{\sqrt{r_{1}^{2} + r_{2}^{2}}}$
D $\frac{2 r_{2}^{2} r_{1}}{\sqrt{r_{1}^{2} + r_{2}^{2}}}$

CENGAGE PUBLICATION-CIRCLE -CONCEPT APPLICATION EXERCISE 4.7

Home
Ask Doubt
Profile