Question

Let A,B, and C be three sets such that
$A=\{(x,y)\mid \frac{x}{\cos \theta }=\frac{y}{\sin \theta }=5,\text{where}\text{'}\theta \text{'}\text{is parameter}\}$
$B=\{(x,y)\mid \frac{x-3}{\cos \varphi }=\frac{y-4}{\sin \varphi }=r\}$
$C=\{(x,y)\mid {(x-3)}^2+{(y-4)}^2\le R^2\}$
If $\varphi$ is fixed and r varies and $(A\cap B)=1$, then $\sec \varphi$ is equal to
(a) $\frac{5}{4}$
(b) $\frac{-5}{4}$
(c) $\frac{5}{3}$
(d) $\frac{-5}{3}$

Options

A. `(5)/(4)`
B. `(-5)/(4)`
C. `(5)/(3)`
D. `(-5)/(3)`

Answer

2

Explanation

`A= { (x,y)}(x)/(cos theta) =(y)/(sin theta) =5, ` where `theta ` is parameter `}`
A is set of points which lie of the circle `C_(1) : x^(2)+y^(2) =25`
`B = { (x,y)|(x-3)/(cos phi)=(y-4)/(sin phi) =r}`
If` phi` varies and r is fixed, then B is the set of all points which lie on a circle `C_(2) : (x-3)^(2) +(y-4)^(2) =r^(2)`
And if `phi` is fixed and r varies, then B is the set of all points which lie on the straight line `L : y-4= tan theta ( x-3)`.

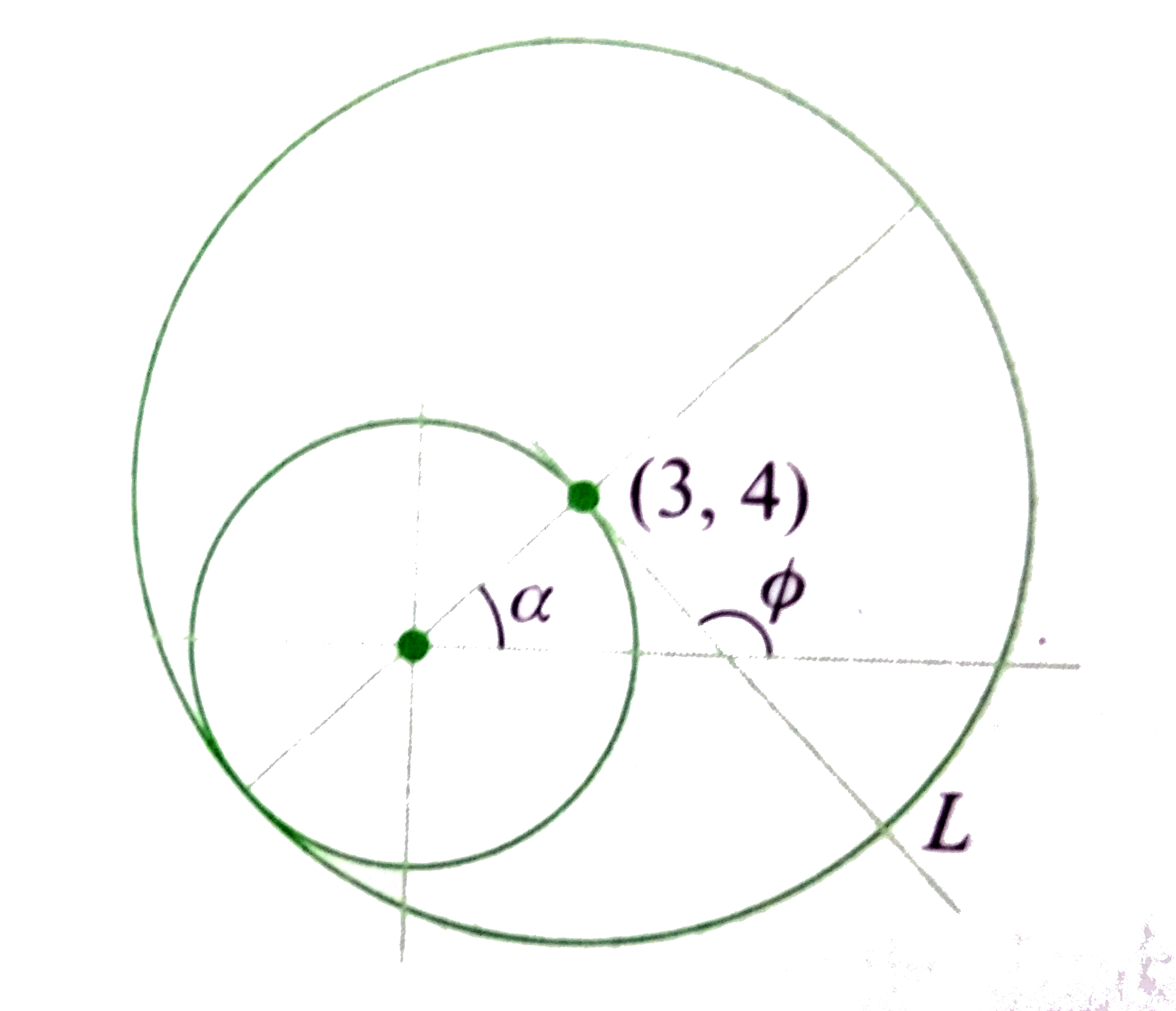
Now, if `A cap C =A`
Both circle `C_(1)` and `C_(2)` must touch each other internally.
So, R must be 10.
If `phi` is fixed and r varies and `n ( A cap B) = 1`, circle `C_(1)` touches line L.
`phi =90^(@) + alpha`
`:. sec phi = sec ( 90^(@) +alpha)= - cosect alpha = - (5)/(4)`